Hostname: page-component-586b7cd67f-g8jcs Total loading time: 0 Render date: 2024-11-28T07:15:40.410Z Has data issue: false hasContentIssue false
  • English
  • Français

Renormalization group analysis of the magnetohydrodynamic turbulence and dynamo

Published online by Cambridge University Press:  06 September 2021

Krzysztof A. Mizerski Open the ORCID record for Krzysztof A. Mizerski [Opens in a new window]
Krzysztof A. Mizerski*
Affiliation:
Department of Magnetism, Institute of Geophysics, Polish Academy of Sciences, ul. Ksiecia Janusza 64, 01-452, Warsaw, Poland
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

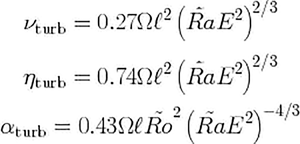
The magnetohydrodynamic (MHD) turbulence appears in engineering laboratory flows and is a common phenomenon in natural systems, e.g. stellar and planetary interiors and atmospheres and the interstellar medium. The applications in engineering are particularly interesting due to the recent advancement of tokamak devices, reaching very high plasma temperatures, thus giving hope for the production of thermonuclear fusion power. In the case of astrophysical applications, perhaps the main feature of the MHD turbulence is its ability to generate and sustain large-scale and small-scale magnetic fields. However, a crucial effect of the MHD turbulence is also the enhancement of large-scale diffusion via interactions of small-scale pulsations, i.e. the generation of the so-called turbulent viscosity and turbulent magnetic diffusivity, which typically exceed by orders of magnitude their molecular counterparts. The enhanced resistivity plays an important role in the turbulent dynamo process. Estimates of the turbulent electromotive force (EMF), including the so-called $\alpha$-effect responsible for amplification of the magnetic energy and the turbulent magnetic diffusion are desired. Here, we apply the renormalization group technique to extract the final expression for the turbulent EMF from the fully nonlinear dynamical equations (Navier–Stokes, induction equation). The simplified renormalized set of dynamical equations, including the equations for the means and fluctuations, is derived and the effective turbulent coefficients such as the viscosity, resistivity, the $\alpha$-coefficient and the Lorentz-force coefficients are explicitly calculated. The results are also used to demonstrate the influence of magnetic fields on energy and helicity spectra of strongly turbulent flows, in particular the magnetic energy spectrum.

JFM classification

MHD and Electrohydrodynamics: MHD turbulence Turbulent Flows: Turbulence theory MHD and Electrohydrodynamics: Dynamo theory
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 926 , 10 November 2021 , A13
Check for updates
Copyright
© The Author(s), 2021. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Adzhemyan, L.T., Antonov, N.V. & Vasiliev, A.N. 1999 Field Theoretic Renormalization Group in Fully Developed Turbulence. Gordon and Breach Science Publishers.Google Scholar
Adzhemyan, L.T., Vasiliev, A.N. & Gnatich, M. 1987 Turbulent dynamo as spontaneous symmetry breaking. Theor. Math. Phys. 72, 940–950.CrossRefGoogle Scholar
Arponen, H. & Horvai, P. 2007 Dynamo effect in the Kraichnan magnetohydrodynamic turbulence. J.Stat. Phys. 129, 205–239.CrossRefGoogle Scholar
Balbus, S.A. & Hawley, J.F. 1991 a A powerful local shear instability in weakly magnetized disks. I - linear analysis. Astrophys. J. 376, 214–222.CrossRefGoogle Scholar
Balbus, S.A. & Hawley, J.F. 1991 b A powerful local shear instability in weakly magnetized disks. II - nonlinear evolution. Astrophys. J. 376, 223–233.CrossRefGoogle Scholar
Barbi, D. & Münster, G. 2013 Renormalisation group analysis of turbulent hydrodynamics. Phys. Res. Intl 2013, 872796.CrossRefGoogle Scholar
Brandenburg, A. & Subramanian, K. 2005 Astrophysical magnetic fields and nonlinear dynamo theory. Phys. Rep. 417, 1–209.CrossRefGoogle Scholar
Brissaud, A., Frisch, U., Leorat, J., Lesieur, M. & Mazure, A. 1973 Helicity cascades in fully developed isotropic turbulence. Phys. Fluids 16, 1366–1367.CrossRefGoogle Scholar
Calkins, M.A. 2018 Quasi-geostrophic dynamo theory. Phys. Earth Planet. Inter. 276, 182–189.CrossRefGoogle Scholar
Calkins, M.A., Julien, K., Tobias, S.M. & Aurnou, J.M. 2015 A multiscale dynamo model driven by quasi-geostrophic convection. J.Fluid Mech. 780, 143–166.CrossRefGoogle Scholar
Chen, Q., Chen, S., Eyink, G.L. & Holm, D.D. 2003 Intermittency in the joint cascade of energy and helicity. Phys. Rev. Lett. 90, 214503.CrossRefGoogle ScholarPubMed
Childress, S. & Soward, A.M. 1972 Convection-driven hydromagnetic dynamo. Phys. Rev. Lett. 29, 837–839.CrossRefGoogle Scholar
Dormy, E. & Soward, A.M. 2007 Mathematical Aspects of Natural Dynamos. Chapman & Hall/CRC Taylor & Francis Group.CrossRefGoogle Scholar
Eyink, G.L. 1994 The renormalization group method in statistical hydrodynamics. Phys. Fluids 6, 3063–3078.CrossRefGoogle Scholar
Forster, D., Nelson, D.R. & Stephen, M.J. 1977 Large-distance and long-time properties of a randomly stirred fluid. Phys. Rev. A 16, 732–749.CrossRefGoogle Scholar
Hughes, D.W. & Tobias, S.M. 2010 An Introduction to Mean Field Dynamo Theory, 15–48. World Scientific Publishing Co.Google Scholar
Kleeorin, N. & Rogachevskii, I. 1994 Effective Ampère force in developed magnetohydrodynamic turbulence. Phys. Rev. E 50, 2716–2730.CrossRefGoogle ScholarPubMed
Kraichnan, R.H. 1959 The structure of isotropic turbulence at very high Reynolds numbers. J.Fluid Mech. 5, 497–543.CrossRefGoogle Scholar
Kraichnan, R.H. 1965 Lagrangian-history closure approximation for turbulence. Phys. Fluids 8, 575–598.CrossRefGoogle Scholar
Krause, F. & Rädler, K.-H. 1980 Mean-Field Magnetohydrodynamics and Dynamo Theory. Pergamon Press.Google Scholar
Lam, S.H. 1992 On the RNG theory of turbulence. Phys. Fluids A 4, 1007–1017.CrossRefGoogle Scholar
Landau, L.D. & Lifshitz, E.M. 1987 Fluid Mechanics. Course of Theoretical Physics, vol. 6. Elsevier.Google Scholar
Ma, S. & Mazenko, G.F. 1975 Critical dynamics of ferromagnets in 6-$\epsilon$ dimensions: general discussion and detailed calculation. Phys. Rev. B 11, 4077–4100.CrossRefGoogle Scholar
McComb, W.D. 2014 Homogeneous, Isotropic Turbulence. Phenomenology, Renormalization and Statistical Closures. Oxford University Press.CrossRefGoogle Scholar
McComb, W.D., Roberts, W. & Watt, A.G. 1992 Conditional-averaging procedure for problems with mode-mode coupling. Phys. Rev. A 45, 3507–3515.CrossRefGoogle ScholarPubMed
McComb, W.D. & Watt, A.G. 1990 Conditional averaging procedure for the elimination of the small-scale modes from incompressible fluid turbulence at high Reynolds numbers. Phys. Rev. Lett. 65, 3281–3284.CrossRefGoogle ScholarPubMed
McComb, W.D. & Watt, A.G. 1992 Two-field theory of incompressible-fluid turbulence. Phys. Rev. A 46, 4797–4812.CrossRefGoogle ScholarPubMed
Mizerski, K.A. 2018 a Large-scale hydromagnetic dynamo by Lehnert waves in nonresistive plasma. SIAM J. Appl. Maths 78, 1402–1421.CrossRefGoogle Scholar
Mizerski, K.A. 2018 b Large-scale dynamo action driven by forced beating waves in a highly conducting plasma. J.Plasma Phys. 84, 735840405.CrossRefGoogle Scholar
Mizerski, K.A. 2020 Renormalization group analysis of the turbulent hydromagnetic dynamo: effect of nonstationarity. Astrophys. J. Suppl. Ser. 251, 21 (29pp).CrossRefGoogle Scholar
Mizerski, K.A. 2021 Renormalization group analysis of the turbulent hydromagnetic dynamo: effect of anizotropy. Appl. Math. Comput. 405, 126252.Google Scholar
Mizerski, K.A. & Tobias, S.M. 2013 Large-scale convective dynamos in a stratified rotating plane layer. Geophys. Astrophys. Fluid Dyn. 107, 218–243.CrossRefGoogle Scholar
Moffatt, H.K. 1981 Some developments in the theory of turbulence. J.Fluid Mech. 106, 27–47.CrossRefGoogle Scholar
Moffatt, H.K. 1983 Transport effects associated with turbulence with particular attention to the influence of helicity. Rep. Prog. Phys. 46, 621–664.CrossRefGoogle Scholar
Moffatt, H.K. & Dormy, E. 2019 Self-Exciting Fluid Dynamos. Cambridge University Press.CrossRefGoogle Scholar
Roberts, P.H. 1994 Fundamentals of dynamo theory. In Lectures on Solar and Planetary Dynamos (ed. M. Proctor & A. Gilbert), pp. 1–58 (Publications of the Newton Institute). Cambridge University Press.CrossRefGoogle Scholar
Roberts, P.H. & King, E.M. 2013 On the genesis of the Earth's magnetism. Rep. Prog. Phys. 76, 096801.CrossRefGoogle ScholarPubMed
Roberts, P.H. & Soward, A.M. 1972 Magnetohydrodynamics of the Earth's core. Ann. Rev. Fluid Mech. 4, 117–154.CrossRefGoogle Scholar
Rogachevskii, I. & Kleeorin, N. 2004 Nonlinear theory of a ‘shear-current’ effect and mean-field magnetic dynamos. Phys. Rev. E 70, 046310.CrossRefGoogle ScholarPubMed
Rubinstein, R. & Barton, J.M. 1991 Renormalization group analysis of anisotropic diffusion in turbulent shear flows. Phys. Fluids A 3, 415–421.CrossRefGoogle Scholar
Rubinstein, R. & Barton, J.M. 1992 Renormalization group analysis of the Reynolds stress transport equation. Phys. Fluids A 4, 1759–1766.CrossRefGoogle Scholar
Ruzmaikin, A.A. & Shukurov, A.M. 1982 Spectrum of the galactic magnetic fields. Astrophys. Space Sci. 82, 397–407.CrossRefGoogle Scholar
Smith, L.M. & Reynolds, W.C. 1992 On the Yakhot-Orszag renormalization group method for deriving turbulence statistics and models. Phys. Fluids A 4, 364–390.CrossRefGoogle Scholar
Smith, L.M. & Woodruff, S.L. 1998 Renormalization-group analysis of turbulence. Annu. Rev. Fluid Mech. 30, 275–310.CrossRefGoogle Scholar
Soward, A.M. 1974 A convection-driven dynamo I. The weak field case. Philos. Trans. R. Soc. Lond. A 275, 611–651.Google Scholar
Steenbeck, M., Krause, F. & Radler, K.-H. 1966 A calculation of the mean electromotive force in an electrically conducting fluid in turbulent motion, under the influence of Coriolis forces. Z. Naturforsch. 21a, 369–376. [English translation: Roberts & Stix (1971), pp. 29–47].CrossRefGoogle Scholar
Tobias, S.M. 2021 The turbulent dynamo. J.Fluid Mech. 912, P1.CrossRefGoogle ScholarPubMed
Tobias, S.M., Cattaneo, F. & Boldyrev, S. 2013 MHD dynamos and turbulence. In Turbulence (ed. P.A. Davidson, Y. Kaneda & K.R. Sreenivasan), pp. 351–404. Cambridge University Press.CrossRefGoogle Scholar
Vincenzi, D. 2002 The Kraichnan–Kazantsev dynamo. J.Stat. Phys. 106, 1073–1091.CrossRefGoogle Scholar
Wyld, H.W. Jr. 1961 Formulation of the theory of turbulence in an incompressible fluid. Ann. Phys. 14, 143–165.CrossRefGoogle Scholar
Yakhot, V. & Orszag, S.A. 1986 Renormalization group analysis of turbulence. I. Basic theory. J.Sci. Comput. 1, 3–51.CrossRefGoogle Scholar
Yokoi, N. 2013 Cross helicity and related dynamo. Geophys. Astrophys. Fluid Dyn. 107, 114–184.CrossRefGoogle Scholar
Yokoi, N. 2018 Electromotive force in strongly compressible magnetohydrodynamic turbulence. J.Plasma Phys. 84, 735840501.CrossRefGoogle Scholar
Yokoi, N. 2020 Turbulence, transport and reconnection. In Topics in Magnetohydrodynamic Topology, Reconnection and Stability Theory (ed. D. MacTaggart & A. Hillier), vol. 59. CISM International Centre for Mechanical Sciences, Springer.CrossRefGoogle Scholar
Yokoi, N. & Yoshizawa, A. 1993 Statistical analysis of the effects of helicity in inhomogeneous turbulence. Phys. Fluids A 5, 464–477.CrossRefGoogle Scholar
Yoshizawa, A. 1990 Self-consistent turbulent dynamo modeling of reversed field pinches and planetary magnetic fields. Phys. Fluids B 2, 1589–1600.CrossRefGoogle Scholar
Yoshizawa, A. 1998 Hydrodynamic and Magnetohydrodynamic Turbulent Flows: Modelling and Statistical Theory. Kluwer Academic Publishers.CrossRefGoogle Scholar
Zeldovich, Y.B., Molchanov, S.A., Ruzmaikin, A.A. & Sokoloff, D.D. 1987 Intermittency in random media. Usp. Fiz. Nauk 152, 3–32. [English translation: 1987 Sov. Phys. Usp. 30, pp. 353–369].CrossRefGoogle Scholar
Zeldovich, Y.B., Ruzmaikin, A.A. & Sokoloff, D.D. 1990 The Almighty Chance. World Scientific.CrossRefGoogle Scholar
Zhou, Y. 2010 Renormalization group theory for fluid and plasma turbulence. Phys. Rep. 488, 1–49.CrossRefGoogle Scholar