Hostname: page-component-586b7cd67f-2brh9 Total loading time: 0 Render date: 2024-11-28T14:25:35.185Z Has data issue: false hasContentIssue false
  • English
  • Français

On the shear-driven surfactant layer instability

Published online by Cambridge University Press:  30 March 2022

Aleksey Mizev Open the ORCID record for Aleksey Mizev [Opens in a new window] ,
Andrey Shmyrov  and
Anastasia Shmyrova Open the ORCID record for Anastasia Shmyrova [Opens in a new window]
Aleksey Mizev*
Affiliation:
Institute of Continuous Media Mechanics, Ural Branch of RAS, Academic Koroleva St., 1, Perm 614000, Russia
Andrey Shmyrov
Affiliation:
Institute of Continuous Media Mechanics, Ural Branch of RAS, Academic Koroleva St., 1, Perm 614000, Russia
Anastasia Shmyrova
Affiliation:
Institute of Continuous Media Mechanics, Ural Branch of RAS, Academic Koroleva St., 1, Perm 614000, Russia
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

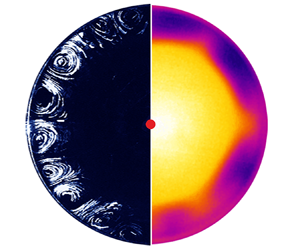
The convective flow generated by a source located at a water surface containing an insoluble surfactant has been investigated experimentally. The application of various sources, which differ in the way in which they drive the interface into motion, and two surfactants with different rheological properties made it possible to generalize the results and to develop a unified approach to describing the problem. We show that the threshold occurrence of a two-zone flow structure with an axisymmetric flow nearby the source and stagnant zone at the periphery results from the competition between two counter-directed shear stresses caused by surfactant concentration inhomogeneity and source influence. We demonstrate that, regardless of the source type, the ratio of these stresses, known as the elasticity number, can be used to predict the formation of a two-zone flow and to define the position of the boundary between the zones. We examined in detail the conditions for formation of the multi-vortex flow within the stagnant zone. Although this phenomenon has been observed by many researchers, it has not yet met a consistent physical explanation. We hypothesized that the formation of vortices can be considered as the instability of the mechanical equilibrium of the surfactant layer caused by the shear on the side of the underlying bulk flow. Based on the mechanism suggested, we introduced a non-dimensional parameter, called the surface Rayleigh number, and estimated its critical value. Finally we analysed the conditions for the occurrence of this instability, called the shear-driven surfactant layer instability in some famous problems of interfacial hydrodynamics.

JFM classification

Convection: Marangoni convection Interfacial Flows (free surface): Capillary flows
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 939 , 25 May 2022 , A24
Check for updates
Copyright
© The Author(s), 2022. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Abramzon, A.A. & Gaevoy, G.M. 1979 Surface-Active Substances (reference book) (in Russian). Khimiya, Leningrad.Google Scholar
Adam, N.K. 1952 The Physics and Chemistry of Surfaces. Oxford University Press.Google Scholar
Adamson, A.W. & Gast, A.P. 1967 Physical Chemistry of Surfaces, vol. 15. Interscience.Google Scholar
Addison, J.V. & Schechter, R.S. 1979 An experimental study of the rheological behavior of surface films. AIChE J. 25 (1), 32–41.CrossRefGoogle Scholar
Bandi, M.M., Akella, V.S., Singh, D.K., Singh, R.S. & Mandre, S. 2017 Hydrodynamic signatures of stationary Marangoni-driven surfactant transport. Phys. Rev. Lett. 119 (26), 264501.CrossRefGoogle ScholarPubMed
Beaumont, F., Liger-Belair, G. & Polidori, G. 2016 Unveiling self-organized two-dimensional (2D) convective cells in champagne glasses. J. Food. Engng 188, 58–65.CrossRefGoogle Scholar
Berg, J.C. & Acrivos, A. 1965 The effect of surface active agents on convection cells induced by surface tension. Chem. Engng Sci. 20 (8), 737–745.CrossRefGoogle Scholar
Bhamla, M.S., Chai, C., Alvarez-Valenzuela, M.A., Tajuelo, J. & Fuller, G.G. 2017 Interfacial mechanisms for stability of surfactant-laden films. PLoS One 12 (5), e0175753.CrossRefGoogle ScholarPubMed
Bickel, T. 2019 Effect of surface-active contaminants on radial thermocapillary flows. Eur. Phys. J. E 42 (10), 131.CrossRefGoogle ScholarPubMed
Bickel, T., Loudet, J.-C., Koleski, G. & Pouligny, B. 2019 Hydrodynamic response of a surfactant-laden interface to a radial flow. Phys. Rev. Fluids 4 (12), 124002.CrossRefGoogle Scholar
Birikh, R.V., Briskman, V.A., Velarde, M.G. & Legros, J.-C. 2003 Liquid Interfacial Systems: Oscillations and Instability, vol. 113. CRC.CrossRefGoogle Scholar
Boussinesq, M.J. 1913 Sur l'existence d'une viscosité superficielle, dans la mince couche de transition séparant un liquide d'un autre fluide contigu. Ann. Chim. Phys. 29, 349–357.Google Scholar
Bratukhin, Iu.K & Maurin, L.N. 1967 Thermocapillary convection in a fluid filling a half-space: PMM vol. 31, no. 3, 1967, pp. 577–580. Z. Angew. Math. Mech. 31 (3), 605–608.CrossRefGoogle Scholar
Bratukhin, Iu.K. & Maurin, L.N. 1982 Stability of thermocapillary convection in a fluid filling a half-space. Z. Angew. Math. Mech. 46 (1), 129–131.CrossRefGoogle Scholar
Bratukhin, Yu.K. & Makarov, S.O. 1992 Secondary thermocapillary motions of soliton type. Fluid Dyn. 27 (4), 463–469.CrossRefGoogle Scholar
Carpenter, B. & Homsy, G.M. 1985 The effect of surface contamination on thermocapillary flow in a two-dimensional slot. Part 2. Partially contaminated interfaces. J. Fluid Mech. 155, 429–439.CrossRefGoogle Scholar
Couder, Y., Chomaz, J.M. & Rabaud, M. 1989 On the hydrodynamics of soap films. Physica D 37 (1–3), 384–405.CrossRefGoogle Scholar
Cuenot, B., Magnaudet, J. & Spennato, B. 1997 The effects of slightly soluble surfactants on the flow around a spherical bubble. J. Fluid Mech. 339, 25–53.CrossRefGoogle Scholar
Danov, K.D., Kralchevsky, P.A. & Ivanov, I.B. 1999 Equilibrium and dynamics of surfactant adsorption monolayers and thin liquid films. In Handbook of Detergents, Part A: Properties (ed. G. Broze), pp. 303–418. CRC.CrossRefGoogle Scholar
Davis, R.E. & Acrivos, A. 1966 The influence of surfactants on the creeping motion of bubbles. Chem. Engng Sci. 21 (8), 681–685.CrossRefGoogle Scholar
Gaver, D.P. & Grotberg, J.B. 1990 The dynamics of a localized surfactant on a thin film. J. Fluid Mech. 213, 127–148.CrossRefGoogle Scholar
Goldshtik, M., Hussain, F. & Shtern, V. 1991 Symmetry breaking in vortex-source and Jeffery—Hamel flows. J. Fluid Mech. 232, 521–566.CrossRefGoogle Scholar
Halpern, D. & Grotberg, J.B. 1992 Dynamics and transport of a localized soluble surfactant on a thin film. J. Fluid Mech. 237, 1–11.CrossRefGoogle Scholar
Harper, J.F. 1974 On spherical bubbles rising steadily in dilute surfactant solutions. Q. J. Mech. Appl. Maths 27 (1), 87–100.CrossRefGoogle Scholar
Homsy, G.M. & Meiburg, E. 1984 The effect of surface contamination on thermocapillary flow in a two-dimensional slot. J. Fluid Mech. 139, 443–459.CrossRefGoogle Scholar
Joly, M. & Matijeic, E. 1972 Rheological properties of monomolecular films, Part II: experimental results, theoretical interpretation, applications. In Surface and Colloid Science (ed. E. Matijevic), vol. 5, pp. 78–193. Wiley.Google Scholar
Karakashev, S.I., Ivanova, D.S., Angarska, Z.K., Manev, E.D., Tsekov, R., Radoev, B., Slavchov, R. & Nguyen, A.V. 2010 Comparative validation of the analytical models for the Marangoni effect on foam film drainage. Colloids Surf. (A) 365 (1–3), 122–136.CrossRefGoogle Scholar
Karakashev, S.I. & Manev, E.D. 2015 Hydrodynamics of thin liquid films: retrospective and perspectives. Adv. Colloid Interface Sci. 222, 398–412.CrossRefGoogle Scholar
Koleski, G., Vilquin, A., Loudet, J.-C., Bickel, T. & Pouligny, B. 2020 Azimuthal instability of the radial thermocapillary flow around a hot bead trapped at the water–air interface. Phys. Fluids 32 (9), 092108.CrossRefGoogle Scholar
Landau, L.D. & Lifshitz, E.M. 2013 Fluid Mechanics: Course of Theoretical Physics, vol. 6. Elsevier.Google Scholar
Li, H., Li, Z., Tan, X., Wang, X., Huang, S., Xiang, Y., Lv, P. & Duan, H. 2020 Three-dimensional backflow at liquid–gas interface induced by surfactant. J. Fluid Mech. 899, A8.CrossRefGoogle Scholar
Linde, H. & Friese, P. 1971 Experimenteller nachweis einer neuen hydrodynamischen oberflachenstabilitat. Z. Phys. Chem. 247 (2), 225–232.CrossRefGoogle Scholar
Liu, H., Zhang, J., Ba, Y., Wang, N. & Wu, L. 2020 Modelling a surfactant-covered droplet on a solid surface in three-dimensional shear flow. J. Fluid Mech. 897, A33.CrossRefGoogle Scholar
Luo, Z.Y., Shang, X.L. & Bai, B.F. 2018 Marangoni effect on the motion of a droplet covered with insoluble surfactant in a square microchannel. Phys. Fluids 30 (7), 077101.CrossRefGoogle Scholar
Luo, Z.Y., Shang, X.L. & Bai, B.F. 2019 Influence of pressure-dependent surface viscosity on dynamics of surfactant-laden drops in shear flow. J. Fluid Mech. 858, 91–121.CrossRefGoogle Scholar
Maali, A., Boisgard, R., Chraibi, H., Zhang, Z., Kellay, H. & Würger, A. 2017 Viscoelastic drag forces and crossover from no-slip to slip boundary conditions for flow near air-water interfaces. Phys. Rev. Lett. 118 (8), 084501.CrossRefGoogle ScholarPubMed
Magnaudet, J. & Eames, I. 2000 The motion of high-Reynolds-number bubbles in inhomogeneous flows. Annu. Rev. Fluid Mech. 32 (1), 659–708.CrossRefGoogle Scholar
Mandracchia, B., Wang, Z., Ferraro, V., Villone, M.M., Di Maio, E., Maffettone, P.L. & Ferraro, P. 2019 Quantitative imaging of the complexity in liquid bubbles’ evolution reveals the dynamics of film retraction. Light: Sci. Appl. 8 (1), 1–12.CrossRefGoogle ScholarPubMed
Mandre, S. 2017 Axisymmetric spreading of surfactant from a point source. J. Fluid Mech. 832, 777–792.CrossRefGoogle Scholar
Manev, E.D., Vassilieff, C.S. & Ivanov, I.B. 1976 Hydrodynamics of thin liquid films effect of surface diffusion on the rate of thinning of foam films. Colloid Polym. Sci. 254 (1), 99–102.CrossRefGoogle Scholar
Manikantan, H. & Squires, T.M. 2020 Surfactant dynamics: hidden variables controlling fluid flows. J. Fluid Mech. 892, P1.CrossRefGoogle ScholarPubMed
Marusic, I. & Broomhall, S. 2021 Leonardo da Vinci and fluid mechanics. Annu. Rev. Fluid Mech. 53, 1–25.CrossRefGoogle Scholar
Matsumoto, Y., Uda, T. & Takagi, S. 2006 The effect of surfactant on rising bubbles. In IUTAM Symposium on Computational Approaches to Multiphase Flow (ed. S. Balachandar & A. Prosperetti), pp. 311–321. Springer.CrossRefGoogle Scholar
Merson, R.L. & Quinn, J.A. 1965 Stagnation in a fluid interface: properties of the stagnant film. AIChE J. 11 (3), 391–395.CrossRefGoogle Scholar
Miller, R. & Liggieri, L. 2009 Interfacial Rheology, vol. 1. CRC.CrossRefGoogle Scholar
Miller, R. & Liggieri, L. 2011 Bubble and Drop Interfaces. CRC.CrossRefGoogle Scholar
Mizev, A. 2005 Influence of an adsorption layer on the structure and stability of surface tension driven flows. Phys. Fluids 17 (12), 122107.CrossRefGoogle Scholar
Mizev, A.I. & Schwabe, D. 2009 Convective instabilities in liquid layers with free upper surface under the action of an inclined temperature gradient. Phys. Fluids 21 (11), 112102.CrossRefGoogle Scholar
Mizev, A.I. & Trofimenko, A.I. 2014 Effect of an insoluble surfactant film on the stability of the concentration-driven Marangoni flow. Fluid Dyn. 49 (1), 26–36.CrossRefGoogle Scholar
Mizev, A., Trofimenko, A., Schwabe, D. & Viviani, A. 2013 Instability of marangoni flow in the presence of an insoluble surfactant. Experiments. Eur. Phys. J.: Spec. Top. 219 (1), 89–98.Google Scholar
Mobius, D. & Miller, R. 1997 Drops and Bubbles in Interfacial Research. Elsevier.Google Scholar
Nepomnyashchy, A., Legros, J.C. & Simanovskii, I. 2006 Interfacial Convection in Multilayer Systems. Springer.Google Scholar
Nepomnyashchy, A.A., Velarde, M.G. & Colinet, P. 2001 Interfacial Phenomena and Convection. CRC.CrossRefGoogle Scholar
Palaparthi, R., Papageorgiou, D.T. & Maldarelli, C. 2006 Theory and experiments on the stagnant cap regime in the motion of spherical surfactant-laden bubbles. J. Fluid Mech. 559, 1–44.CrossRefGoogle Scholar
Pshenichnikov, A.F. & Yatsenko, S.S. 1974 Convective diffusion from a localized source of surfactant (in Russian). Hydrodynamics (Scientific notes of Perm State University) 5, 175–181.Google Scholar
Radoev, B.P., Dimitrov, D.S. & Ivanov, I.B. 1974 Hydrodynamics of thin liquid films effect of the surfactant on the rate of thinning. Colloid Polym. Sci. 252 (1), 50–55.CrossRefGoogle Scholar
Raghunandan, A., Hirsa, A.H., Underhill, P.T. & Lopez, J.M. 2018 Predicting steady shear rheology of condensed-phase monomolecular films at the air-water interface. Phys. Rev. Lett. 121 (16), 164502.CrossRefGoogle ScholarPubMed
Rahni, M.T., Karbaschi, M. & Miller, R. 2015 Computational Methods for Complex Liquid-Fluid Interfaces, vol. 5. CRC.CrossRefGoogle Scholar
Roché, M., Li, Z., Griffiths, I.M., Le Roux, S., Cantat, I., Saint-Jalmes, A. & Stone, H.A. 2014 Marangoni flow of soluble amphiphiles. Phys. Rev. Lett. 112 (20), 208302.CrossRefGoogle Scholar
Saffman, P.G. & Delbrück, M. 1975 Brownian motion in biological membranes. Proc. Natl Acad. Sci. USA 72 (8), 3111–3113.CrossRefGoogle ScholarPubMed
Savic, P. 1953 Circulation and distortion of liquid drops falling through a viscous medium report. Tech. Rep. NRC-MT-22. Natl. Res. Council, Canada.Google Scholar
Scott, J.C. 1982 Flow beneath a stagnant film on water: the Reynolds ridge. J. Fluid Mech. 116, 283–296.CrossRefGoogle Scholar
Scriven, L.E. 1960 Dynamics of a fluid interface equation of motion for Newtonian surface fluids. Chem. Engng Sci. 12 (2), 98–108.CrossRefGoogle Scholar
Shmyrov, A., Mizev, A., Demin, V., Petukhov, M. & Bratsun, D. 2018 On the extent of surface stagnation produced jointly by insoluble surfactant and thermocapillary flow. Adv. Colloid Interface Sci. 255, 10–17.CrossRefGoogle ScholarPubMed
Shmyrov, A.V., Mizev, A.I., Demin, V.A., Petukhov, M.I. & Bratsun, D.A. 2019 Phase transitions on partially contaminated surface under the influence of thermocapillary flow. J. Fluid Mech. 877, 495–533.CrossRefGoogle Scholar
Shmyrova, A. & Shmyrov, A. 2021 Experimental study of the flow structure stability on the bubble surface. J. Phys.: Conf. Ser. 1945 (1), 012053.Google Scholar
Shtern, V. & Hussain, F. 1993 Azimuthal instability of divergent flows. J. Fluid Mech. 256, 535–560.CrossRefGoogle Scholar
Squire, H.B. 1955 Radial jets. In 50 Jahre Grenzschichtforschung (ed. H. Görtler & W. Tollmien), pp. 47–54. Springer.CrossRefGoogle Scholar
Starov, V. & Ivanov, I. 2004 Fluid Mechanics of Surfactant and Polymer Solutions, vol. 463. Springer.CrossRefGoogle Scholar
Suda, S., Suda, T., Ohmura, T. & Ichikawa, M. 2021 Straight-to-curvilinear motion transition of a swimming droplet caused by the susceptibility to fluctuations. Phys. Rev. Lett. 127, 088005.CrossRefGoogle ScholarPubMed
Suja, V.C., Kar, A., Cates, W., Remmert, S.M., Savage, P.D. & Fuller, G.G. 2018 Evaporation-induced foam stabilization in lubricating oils. Proc. Natl Acad. Sci. USA 115 (31), 7919–7924.CrossRefGoogle Scholar
Tasoglu, S., Demirci, U. & Muradoglu, M. 2008 The effect of soluble surfactant on the transient motion of a buoyancy-driven bubble. Phys. Fluids 20 (4), 040805.CrossRefGoogle Scholar
Thomson, J. 1855 XLII. On certain curious motions observable at the surfaces of wine and other alcoholic liquors. Lond. Edinb. Dublin Philos. Mag. J. Sci. 10 (67), 330–333.CrossRefGoogle Scholar
Vargaftik, N.B., Volkov, B.N. & Voljak, L.D. 1983 International tables of the surface tension of water. J. Phys. Chem. Ref. Data 12 (3), 817–820.CrossRefGoogle Scholar
Velarde, M.G. 2012 Physicochemical Hydrodynamics: Interfacial Phenomena, vol. 174. Springer.Google Scholar
Vogel, M.J. & Hirsa, A.H. 2002 Concentration measurements downstream of an insoluble monolayer front. J. Fluid Mech. 472, 283–305.CrossRefGoogle Scholar
Wang, S.-P., Zhang, A.-M., Liu, Y.-L., Zhang, S. & Cui, P. 2018 Bubble dynamics and its applications. J. Hydrodyn. 30 (6), 975–991.CrossRefGoogle Scholar
Warncke, A.A., Gharib, M.M. & Roesgen, T.T. 1996 Flow measurements near a Reynolds ridge. Trans. ASME J. Fluids Engng 118 (3), 621–624.CrossRefGoogle Scholar
Wegener, M., Eppinger, T., Bäumler, K., Kraume, M., Paschedag, A.R. & Bänsch, E. 2009 Transient rise velocity and mass transfer of a single drop with interfacial instabilities—numerical investigations. Chem. Engng Sci. 64 (23), 4835–4845.CrossRefGoogle Scholar
White, A.R. & Ward, T. 2019 Surface remobilization of buoyancy-driven surfactant-laden drops at low Reynolds and capillary numbers. AIChE J. 65 (1), 294–304.CrossRefGoogle Scholar

Mizev et al. supplementary movie 1

See word file for movie caption

Download Mizev et al. supplementary movie 1(Video)
Video 5.3 MB

Mizev et al. supplementary movie 2

See word file for movie caption

Download Mizev et al. supplementary movie 2(Video)
Video 9.3 MB

Mizev et al. supplementary movie 3

See word file for movie caption

Download Mizev et al. supplementary movie 3(Video)
Video 3.9 MB

Mizev et al. supplementary movie 4

See word file for movie caption

Download Mizev et al. supplementary movie 4(Video)
Video 2.5 MB

Mizev et al. supplementary movie 5

See word file for movie caption

Download Mizev et al. supplementary movie 5(Video)
Video 8.7 MB

Mizev et al. supplementary movie 6

See word file for movie caption

Download Mizev et al. supplementary movie 6(Video)
Video 5.5 MB

Mizev et al. supplementary movie 7

See word file for movie caption

Download Mizev et al. supplementary movie 7(Video)
Video 6.8 MB
Supplementary material: File

Mizev et al. supplementary material

Captions for movies 1-7

Download Mizev et al. supplementary material(File)
File 22.3 KB