Hostname: page-component-586b7cd67f-l7hp2 Total loading time: 0 Render date: 2024-11-28T14:14:30.278Z Has data issue: false hasContentIssue false
  • English
  • Français

On the origins of transverse jet shear layer instability transition

Published online by Cambridge University Press:  11 March 2020

Takeshi Shoji Open the ORCID record for Takeshi Shoji [Opens in a new window] ,
Elijah W. Harris ,
Andrea Besnard ,
Stephen G. Schein  and
Ann R. Karagozian Open the ORCID record for Ann R. Karagozian [Opens in a new window]
Takeshi Shoji
Affiliation:
Department of Mechanical and Aerospace Engineering, University of California Los Angeles, Los Angeles, CA90095-1597, USA
Elijah W. Harris
Affiliation:
Department of Mechanical and Aerospace Engineering, University of California Los Angeles, Los Angeles, CA90095-1597, USA
Andrea Besnard
Affiliation:
Department of Mechanical and Aerospace Engineering, University of California Los Angeles, Los Angeles, CA90095-1597, USA
Stephen G. Schein
Affiliation:
Department of Mechanical and Aerospace Engineering, University of California Los Angeles, Los Angeles, CA90095-1597, USA
Ann R. Karagozian*
Affiliation:
Department of Mechanical and Aerospace Engineering, University of California Los Angeles, Los Angeles, CA90095-1597, USA
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

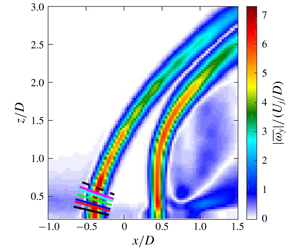
This experimental study explores the physical mechanisms by which a transverse jet’s upstream shear layer can transition from being a convective instability to an absolute/global instability as the jet-to-cross-flow momentum flux ratio $J$ is reduced. As first proposed in computational studies by Iyer & Mahesh (J. Fluid Mech., vol. 790, 2016, pp. 275–307), the upstream shear layer just beyond the jet injection may be analogous to a local counter-current shear layer, which is known for a planar geometry to become absolutely unstable at a large enough counter-current shear layer velocity ratio, $R_{1}$. The present study explores this analogy for a range of transverse jet momentum flux ratios and jet-to-cross-flow density ratios $S$, for jets containing differing species concentrations (nitrogen, helium and acetone vapour) at several different jet Reynolds numbers. These studies make use of experimental data extracted from stereo particle image velocimetry as well as simultaneous stereo particle image velocimetry and acetone planar laser-induced fluorescence imaging. They provide experimental evidence for the relevance of the counter-current shear layer analogy to upstream shear layer instability transition in a nozzle-generated transverse jet.

JFM classification

Instability: Absolute/convective instability Wakes/Jets: Jets Wakes/Jets: Shear layers
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 890 , 10 May 2020 , A7
Copyright
© The Author(s), 2020. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Alves, L. S. de B., Kelly, R. E. & Karagozian, A. R. 2007 Local stability analysis of an inviscid transverse jet. J. Fluid Mech. 581, 401–418.CrossRefGoogle Scholar
Alves, L. S. de B., Kelly, R. E. & Karagozian, A. R. 2008 Transverse-jet shear-layer instabilities. Part 2. Linear analysis for large jet-to-crossflow velocity ratio. J. Fluid Mech. 602, 383–401.CrossRefGoogle Scholar
Bagheri, S., Schlatter, P., Schmid, P. J. & Henningson, D. S. 2009 Global stability of a jet in crossflow. J. Fluid Mech. 624, 33–44.CrossRefGoogle Scholar
Bird, R. B., Stewart, W. E. & Lightfoot, E. N. 1960 Transport Phenomena. John Wiley & Sons.Google Scholar
Canzonieri, K.2009 Experimental studies on low density jets in crossflow. Master’s thesis, University of California, Los Angeles, Department of Mechanical and Aerospace Engineering.Google Scholar
Coenen, W. & Sevilla, A. 2012 The structure of the absolutely unstable regions in the near field of low-density jets. J. Fluid Mech. 713, 123–149.CrossRefGoogle Scholar
Davitian, J., Getsinger, D., Hendrickson, C. & Karagozian, A. R. 2010 Transition to global instability in transverse-jet shear layers. J. Fluid Mech. 661, 294–315.CrossRefGoogle Scholar
Fric, T. F. & Roshko, A. 1994 Vortical structure in the wake of a transverse jet. J. Fluid Mech. 279, 1–47.CrossRefGoogle Scholar
Getsinger, D. R.2012 Shear layer instabilities and mixing in variable density transverse jet flows. PhD thesis, University of California, Los Angeles.Google Scholar
Getsinger, D. R., Gevorkyan, L., Smith, O. I. & Karagozian, A. R. 2014 Structural and stability characteristics of jets in crossflow. J. Fluid Mech. 760, 342–367.CrossRefGoogle Scholar
Getsinger, D. R., Hendrickson, C. & Karagozian, A. R. 2012 Shear layer instabilities in low-density transverse jets. Exp. Fluids 53, 783–801.CrossRefGoogle Scholar
Gevorkyan, L., Shoji, T., Getsinger, D. R., Smith, O. I. & Karagozian, A. R. 2016 Transverse jet mixing characteristics. J. Fluid Mech. 790, 237–274.CrossRefGoogle Scholar
Gevorkyan, L., Shoji, T., Peng, W. Y. & Karagozian, A. R. 2018 Influence of the velocity field on scalar transport in gaseous transverse jets. J. Fluid Mech. 834, 173–219.CrossRefGoogle Scholar
Hallberg, M. P. & Strykowski, P. J. 2006 On the universality of global modes in low-density axisymmetric jets. J. Fluid Mech. 569, 493–507.CrossRefGoogle Scholar
Huerre, P. & Monkewitz, P. A. 1985 Absolute and convective instabilities in free shear layer. J. Fluid Mech. 159, 151–168.CrossRefGoogle Scholar
Huerre, P. & Monkewitz, P. A. 1990 Local and global instabilities in spatially developing flows. Annu. Rev. Fluid Mech. 22, 473–537.CrossRefGoogle Scholar
Hussain, A. K. M. F. & Zaman, K. B. M. Q. 1978 The free shear layer tone phenomenon and probe interference. J. Fluid Mech. 87, 349–383.CrossRefGoogle Scholar
Iyer, P. S. & Mahesh, K. 2016 A numerical study of shear layer characteristics of low-speed transverse jets. J. Fluid Mech. 790, 275–307.CrossRefGoogle Scholar
Jendoubi, S. & Strykowski, P. J. 1994 Absolute and convective instability of axisymmetric jets with external flow. Phys. Fluids 6, 3000–3009.CrossRefGoogle Scholar
Karagozian, A. R. 2010 Transverse jets and their control. Prog. Energy Combust. Sci. 36, 531–553.CrossRefGoogle Scholar
Megerian, S., Davitian, J., Alves, L. S. de B. & Karagozian, A. R. 2007 Transverse-jet shear-layer instabilities. Part 1. Experimental studies. J. Fluid Mech. 593, 93–129.CrossRefGoogle Scholar
Meyer, K. E., Pedersen, J. M. & Özcan, O. 2007 A turbulent jet in crossflow analysed with proper orthogonal decomposition. J. Fluid Mech. 583, 199–227.CrossRefGoogle Scholar
Pavithran, S. & Redekopp, L. G. 1989 The absolute-convective transition in subsonic mixing layers. Phys. Fluids A 1, 1736–1739.CrossRefGoogle Scholar
Peplinski, A., Schlatter, P. & Henningson, D. S. 2015 Global stability and optimal perturbation for a jet in cross-flow. Eur. J. Mech (B/Fluids) 49, 438–447.CrossRefGoogle Scholar
Poling, B. E., Prausnitz, J. M. & O’Connell, J. P. 2001 The Properties of Gases and Liquids, 5th edn. McGraw-Hill.Google Scholar
Regan, M. A. & Mahesh, K. 2017 Global linear stability analysis of jets in cross-flow. J. Fluid Mech. 828, 812–836.CrossRefGoogle Scholar
Shoji, T.2017 Mixing and structural characteristics of unforced and forced jets in crossflow. PhD thesis, University of California, Los Angeles.Google Scholar
Strykowski, P. J. & Niccum, D. L. 1991 The stability of countercurrent mixing layers in circular jets. J. Fluid Mech. 227, 309–343.CrossRefGoogle Scholar
Strykowski, P. J. & Niccum, D. L. 1992 The influence of velocity and density ratio on the dynamics of spatially developing mixing layers. Phys. Fluids A 4 (4), 770–781.CrossRefGoogle Scholar