Hostname: page-component-586b7cd67f-dlnhk Total loading time: 0 Render date: 2024-11-28T14:24:58.242Z Has data issue: false hasContentIssue false
  • English
  • Français

On quasi-steady boundary-layer separation in supersonic flow. Part 2. Downstream moving separation point

Published online by Cambridge University Press:  03 August 2020

A. I. Ruban Open the ORCID record for A. I. Ruban [Opens in a new window] ,
A. Djehizian ,
J. Kirsten  and
M. A. Kravtsova
A. I. Ruban*
Affiliation:
Department of Mathematics, Imperial College London, 180 Queen's Gate, LondonSW7 2AZ, UK
A. Djehizian
Affiliation:
Department of Mathematics, Imperial College London, 180 Queen's Gate, LondonSW7 2AZ, UK
J. Kirsten
Affiliation:
Department of Mathematics, Imperial College London, 180 Queen's Gate, LondonSW7 2AZ, UK
M. A. Kravtsova
Affiliation:
Department of Mathematics, Imperial College London, 180 Queen's Gate, LondonSW7 2AZ, UK
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

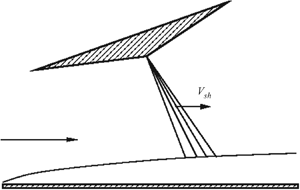
In this paper we study the perturbations produced in the boundary layer by an impinging oblique shock wave or Prandtl–Meyer expansion fan. The flow outside the boundary layer is assumed supersonic, and we also assume that the point, where the shock wave/expansion fan impinges on the boundary layer, moves downstream. To study the flow, it is convenient to use the coordinate frame moving with the shock; in this frame, the body surface moves upstream. We first study numerically the case when the shock velocity $V_{sh} = O (Re^{-1/8})$. In this case the interaction of the boundary layer with the shock can be described by the classical equations of the triple-deck theory. We find that, as $V_{sh}$ increases, the boundary layer proves to be more prone to separation when exposed to the expansion fan, not the compression shock. Then we assume $V_{sh}$ to be in the range $1 \gg V_{sh} \gg Re^{-1/8}$. Under these conditions, the process of the interaction between the boundary layer and the shock/expansion fan can be treated as inviscid and quasi-steady if considered in the reference frame moving with the shock/expansion fan. The inviscid analysis allows us to determine the pressure distribution in the interaction region. We then turn our attention to a thin viscous sublayer that lies closer to the body surface. In this sublayer the flow is described by classical Prandtl's equations. The solution to these equations develops a singularity provided that the expansion fan is strong enough. The flow analysis in a small vicinity of the singular point shows an accelerated ‘expansion’ of the flow similar to the one reported by Neiland (Izv. Akad. Nauk SSSR, Mech. Zhidk. Gaza, vol. 5, 1969a, pp. 53–60) in his analysis of supersonic flow separation from a convex corner.

JFM classification

Boundary Layers: Boundary layer separation Aerodynamics: High-speed flow
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 900 , 10 October 2020 , A9
Copyright
© The Author(s), 2020. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Blasius, H. 1908 Grenzschichten in flüssigkeiten mit kleiner reibung. Z. Mathematik Physik 56, 1–37.Google Scholar
Bogolepov, V. V. & Neiland, V. Ya. 1971 Supersonic viscous flow over a small roughnesses on the surface of a body. Tr. TsAGI, No. 1363.Google Scholar
Cassel, K. W., Smith, F. T. & Walker, J. D. A. 1996 The onset of instability in unsteady boundary-layer separation. J. Fluid Mech. 315, 223–256.CrossRefGoogle Scholar
Christov, C. I. & Tsankov, I. T. 1993 Numerical investigation of the laminar boundary layer flow around an impulsively moved circular cylinder. Comput. Methods Appl. Mech. Engng 109 (1–2), 1–15.CrossRefGoogle Scholar
Cowley, S. J. 1983 Computer extension and analytic continuation of Blasius’ expansion for impulsive flow past a circular cylinder. J. Fluid Mech. 135, 389–405.CrossRefGoogle Scholar
Degani, A. T., Li, Q. & Walker, J. D. A. 1996 Unsteady separation from the leading edge of a thin airfoil. Phys. Fluids 8 (3), 704–714.CrossRefGoogle Scholar
van Dommelen, L. L. & Shen, S. F. 1980 The spontaneous generation of the singularity in a separating laminar boundary layer. J. Comput. Phys. 38 (2), 125–140.CrossRefGoogle Scholar
van Dommelen, L. L. & Shen, S. F. 1982 The genesis of separation. In Numerical and Physical Aspects of Aerodynamic Flows (ed. T. Cebeci), pp. 293–311. Springer.CrossRefGoogle Scholar
van Dommelen, L. L. & Shen, S. F. 1983 An unsteady interactive separation process. AIAA J. 21 (3), 358–362.CrossRefGoogle Scholar
Elliott, J. W., Smith, F. T. & Cowley, S. J. 1983 Breakdown of boundary layers: (i) on moving surfaces; (ii) in semi-similar unsteady flow; (iii) in fully unsteady flow. Geophys. Astrophys. Fluid Dyn. 25 (1–2), 77–138.CrossRefGoogle Scholar
Goldstein, S. 1948 On laminar boundary-layer flow near a position of separation. Q. J. Mech. Appl. Maths 1 (1), 43–69.CrossRefGoogle Scholar
Hartree, D. R. 1939 A solution of the laminar boundary-layer equations for retarded flow. Aeronautical Research Council Reports and Memoranda No. 2426 (issued 1949).Google Scholar
Howarth, L. 1938 On the solution of the laminar boundary layer equations. Proc. R. Soc. Lond. A 164, 547–579.CrossRefGoogle Scholar
Ingham, D. B. 1984 Unsteady separation. J. Comput. Phys. 53 (1), 90–99.CrossRefGoogle Scholar
Kirchhoff, G. 1869 Zur theorie freier flüssigkeitsstrahlen. J. Reine Angew. Math. 70 (4), 289–298.Google Scholar
Kirsten, J. 2018 Viscous-inviscid interaction on moving walls. PhD thesis, Imperial College London.Google Scholar
Kluwick, A. & Wohlfahrt, H. 1986 Hot-wire-anemometer study of the entry flow in a curved duct. J. Fluid Mech. 165, 335–353.CrossRefGoogle Scholar
Krapivskii, P. L. & Neiland, V. Ya. 1982 Boundary-layer separation on a moving body surface in supersonic flow. Uch. Zap. TsAGI 13 (3), 32–42.Google Scholar
Kravtsova, M. A., Zametaev, V. B. & Ruban, A. I. 2005 An effective numerical method for solving viscous-inviscid interaction problems. Phil. Trans. R. Soc. A 363 (1830), 1157–1167.CrossRefGoogle ScholarPubMed
Landau, L. D. & Lifshitz, E. M. 1944 Mechanics of Continuous Media. Gostekhizdat.Google Scholar
Levi-Civita, T. 1907 Scie e leggi di resistenza. Rendconte de Circolo Matematico di Palermo XXIII, 1–37.Google Scholar
Messiter, A. F. 1970 Boundary-layer flow near the trailing edge of a flat plate. SIAM J. Appl. Maths 18 (1), 241–257.CrossRefGoogle Scholar
Moore, F. K. 1958 On the separation of the unsteady laminar boundary-layer. In Boundary Layer Research (ed. H. Görtler), pp. 296–311. Springer.CrossRefGoogle Scholar
Nakayama, Y. 1988 Visualized Flow. Fluid Motion in Basic and Engineering Situations Revealed by Flow Visualization. Pergamon.Google Scholar
Neiland, V. Ya. 1969 a Asymptotic theory for calculating heat fluxes near the corner of a body contour. Izv. Akad. Nauk SSSR, Mech. Zhidk. Gaza 5, 53–60.Google Scholar
Neiland, V. Ya. 1969 b Theory of laminar boundary layer separation in supersonic flow. Izv. Akad. Nauk SSSR, Mech. Zhidk. Gaza (4), 53–57.Google Scholar
Neiland, V. Ya. & Sychev, V. V. 1966 Asymptotic solutions of the Navier–Stokes equations in regions with large local perturbations. Izv. Akad. Nauk SSSR, Mech. Zhidk. Gaza (4), 43–49.Google Scholar
Neiland, V. Ya., Bogolepov, V. V., Dudin, G. N. & Lipatov, I. I. 2007 Asymptotic Theory of Supersonic Viscous Flows. Elsevier, Butterworth-Heinemann.Google Scholar
Peridier, V. J., Smith, F. T. & Walker, J. D. A. 1991 Vortex-induced boundary-layer separation. Part1. The unsteady limit problem ${R}e \to \infty$. J. Fluid Mech. 232, 99–131.CrossRefGoogle Scholar
Prandtl, L. 1904 Über flüssigkeitsbewegung bei sehr kleiner Reibung. In Verh. III. Intern. Math. Kongr., Heidelberg, pp. 484–491. Teubner, 1905.Google Scholar
Rott, N. 1956 Unsteady viscous flow in the vicinity of a stagnation point. Q. Appl. Maths 13 (4), 444–451.CrossRefGoogle Scholar
Ruban, A. I. 2018 Fluid Dynamics. Part 3. Boundary Layers. Oxford University Press.Google Scholar
Ruban, A. I., Araki, D., Yapalparvi, R. & Gajjar, J. S. B. 2011 On unsteady boundary-layer separation in supersonic flow. Part 1. Upstream moving separation point. J. Fluid Mech. 678, 124–155.CrossRefGoogle Scholar
Ruban, A. I. & Vonatsos, K. N. 2008 Discontinuous solutions of the boundary-layer equations. J. Fluid Mech. 614, 407–424.CrossRefGoogle Scholar
Sears, W. R. 1956 Some recent developments in airfoil theory. J. Aeronaut. Sci. 23 (5), 490–499.CrossRefGoogle Scholar
Stewartson, K. 1969 On the flow near the trailing edge of a flat plate. Mathematika 16 (1), 106–121.CrossRefGoogle Scholar
Stewartson, K., Cebeci, T. & Chang, K. C. 1980 A boundary layer collision in a curved duct. Q. J. Mech. Appl. Maths 33, 59–75.CrossRefGoogle Scholar
Stewartson, K. & Williams, P. G. 1969 Self-induced separation. Proc. R. Soc. Lond. A 312, 181–206.Google Scholar
Sychev, V. V. 1972 Laminar separation. Mekh. Zhid. Gaza (3), 47–59 (translation in Fluid Dyn. 7 (3), 407–417).Google Scholar
Sychev, V. V., Ruban, A. I., Sychev, Vic. V. & Korolev, G. L. 1998 Asymptotic Theory of Separated Flows. Cambridge University Press.CrossRefGoogle Scholar
Sychev, Vic. V. 1979 Asymptotic theory of nonstationary separation. Mekh. Zhid. Gaza (6), 21–32 (translation in Fluid Dyn. 14 (6), 829–838).Google Scholar
Sychev, Vic. V. 1980 On certain singulartities in solutions of the boundary-layer equations on a moving surface. Prikl. Mat. Mekh. 44 (5), 831–838.Google Scholar
Sychev, Vic. V. 1983 Theory of unsteady boundary-layer separation and wake breakdown. Usp. Mekh. 6, 13–51.Google Scholar
Sychev, Vic. V. 1984 On the asymptotic theory of laminar separation from a moving surface. Prikl. Mat. Mekh. 48 (2), 247–253 (translation in J. Appl. Math. Mech. 48 (2), 171–176).Google Scholar
Sychev, Vic. V. 1987 Analytical solution of the problem of flow near the boundary-layer separation point on a moving wall. Prikl. Mat. Mekh. 51 (3), 519–521 (translation in J. Appl. Math. Mech. 51 (3), 405–407).Google Scholar
Walker, J. D. A. 1978 The boundary layer due to rectilinear vortex. Proc. R. Soc. Lond. A 359 (1697), 167–188.Google Scholar
Yapalparvi, R. & van Dommelen, L. L. 2012 Numerical solution of unsteady boundary-layer separation in supersonic flow: upstream moving wall. J. Fluid Mech. 706, 413–430.CrossRefGoogle Scholar
Zhuk, V. I. 1982 On local recirculation zones in the supersonic boundary layer on a moving surface. J. Comput. Math. Phys. USSR 22 (5), 249–255.CrossRefGoogle Scholar