Article contents
Mixed convection in a periodically heated channel
Published online by Cambridge University Press: 03 March 2015
Abstract
Mixed convection in a channel with flow driven by a pressure gradient and subject to spatially periodic heating along one of the walls has been studied. The pattern of the heating is characterized by the wavenumber  ${\it\alpha}$ and its intensity is expressed in terms of the Rayleigh number
${\it\alpha}$ and its intensity is expressed in terms of the Rayleigh number  $\mathit{Ra}_{p}$. The primary convection has the form of counter-rotating rolls with the wavevector parallel to the wavevector of the heating. The resulting net heat flow between the walls increases proportionally to
$\mathit{Ra}_{p}$. The primary convection has the form of counter-rotating rolls with the wavevector parallel to the wavevector of the heating. The resulting net heat flow between the walls increases proportionally to 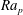 $\mathit{Ra}_{p}$ but the growth saturates when
$\mathit{Ra}_{p}$ but the growth saturates when 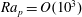 $\mathit{Ra}_{p}=O(10^{3})$. The most effective heating pattern corresponds to
$\mathit{Ra}_{p}=O(10^{3})$. The most effective heating pattern corresponds to 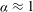 ${\it\alpha}\approx 1$, as this leads to the most intense transverse motion. The primary convection is subject to transition to secondary states with the onset conditions depending on
${\it\alpha}\approx 1$, as this leads to the most intense transverse motion. The primary convection is subject to transition to secondary states with the onset conditions depending on  ${\it\alpha}$. The conditions leading to transition between different forms of secondary motion have been determined using the linear stability theory. Three patterns of secondary motion may occur at small Reynolds numbers
${\it\alpha}$. The conditions leading to transition between different forms of secondary motion have been determined using the linear stability theory. Three patterns of secondary motion may occur at small Reynolds numbers 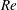 $\mathit{Re}$, i.e. longitudinal rolls, transverse rolls and oblique rolls, with the critical conditions varying significantly as a function of
$\mathit{Re}$, i.e. longitudinal rolls, transverse rolls and oblique rolls, with the critical conditions varying significantly as a function of  ${\it\alpha}$. An increase of
${\it\alpha}$. An increase of  ${\it\alpha}$ leads to the elimination of the longitudinal rolls and, eventually, to the elimination of the oblique rolls, with the transverse rolls assuming the dominant role. For large
${\it\alpha}$ leads to the elimination of the longitudinal rolls and, eventually, to the elimination of the oblique rolls, with the transverse rolls assuming the dominant role. For large  ${\it\alpha}$, the transition is driven by the Rayleigh–Bénard mechanism; while for
${\it\alpha}$, the transition is driven by the Rayleigh–Bénard mechanism; while for 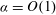 ${\it\alpha}=O(1)$, the spatial parametric resonance dominates. The global flow characteristics are identical regardless of whether the heating is applied at the lower or the upper wall.
${\it\alpha}=O(1)$, the spatial parametric resonance dominates. The global flow characteristics are identical regardless of whether the heating is applied at the lower or the upper wall.
JFM classification
- Type
- Papers
- Information
- Copyright
- © 2015 Cambridge University Press
References
- 30
- Cited by



