Hostname: page-component-586b7cd67f-rdxmf Total loading time: 0 Render date: 2024-12-01T03:06:57.491Z Has data issue: false hasContentIssue false
  • English
  • Français

Linear and weakly nonlinear analyses of cylindrical Couette flow with axial and radial flows

Published online by Cambridge University Press:  06 July 2017

Denis Martinand Open the ORCID record for Denis Martinand [Opens in a new window] ,
Eric Serre  and
Richard M. Lueptow
Denis Martinand*
Affiliation:
Aix-Marseille Univ., CNRS, Centrale Marseille, M2P2, Marseille, France
Eric Serre
Affiliation:
Aix-Marseille Univ., CNRS, Centrale Marseille, M2P2, Marseille, France
Richard M. Lueptow
Affiliation:
Department of Mechanical Engineering, Northwestern University, Evanston, IL 60208, USA
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Extending previous linear stability analyses of the instabilities developing in permeable Taylor–Couette–Poiseuille flows where axial and radial throughflows are superimposed on the usual Taylor–Couette flow, we further examine the linear behaviour and expand the analysis to consider the weakly nonlinear behaviour of convective-type instabilities by means of the derivation of the fifth-order amplitude equation together with direct numerical simulations. Special attention is paid to the influence of the radius ratio 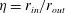$\unicode[STIX]{x1D702}=r_{in}/r_{out}$, and particularly to wide gaps (small $\unicode[STIX]{x1D702}$) and how they magnify the effects of the radial flow. The instabilities take the form of pairs of counter-rotating toroidal vortices superseded by helical ones as the axial flow is increased. Increasing the radial inflow draws these vortices near the inner cylinder, where they shrink relative to the annular gap, when this gap is wide. Strong axial and radial flows in a narrow annular gap lead to a very large azimuthal wavenumber with steeply sloped helical vortices. Strong radial outflow in a wide annular gap results in very large helical vortices. The analytical and numerical saturated vortices match quite well. In addition, radial inflows or outflows can turn the usually supercritical bifurcation from laminar to vortical flow into a subcritical one. The radial flow above which this change occurs decreases as the radius ratio 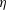$\unicode[STIX]{x1D702}$ decreases. A practical motivation for this weakly nonlinear analysis is found in modelling dynamic filtration devices, which rely on vortical instabilities to reduce the processes of accumulation on their membranes.

JFM classification

Instability: Instability Instability: Nonlinear instability
Type
Papers
Information
Journal of Fluid Mechanics , Volume 824 , 10 August 2017 , pp. 438 - 476
Check for updates
Copyright
© 2017 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Aljishi, M. F., Ruo, A. C., Park, J. H., Nasser, B., Kim, W. S. & Joo, Y. L. 2013 Effect of flow structure at the onset of instability on barium sulfate precipitation in Taylor–Couette crystallizers. J. Cryst. Growth 373, 20–31.Google Scholar
Babcock, K. L., Ahlers, G. & Cannell, D. S. 1991 Noise-sustained structure in Taylor–Couette flow with through-flow. Phys. Rev. Lett. 67, 3388–3391.Google Scholar
Beaudoin, G. & Jaffrin, M. Y. 1989 Plasma filtration in Couette flow membrane devices. Artif. Organs 13 (1), 43–51.Google Scholar
Beavers, G. S. & Joseph, D. D. 1967 Boundary conditions at a naturally permeable wall. J. Fluid Mech. 30, 197–207.Google Scholar
Belfort, G., Mikulasek, P., Pimbley, J. M. & Chung, K. Y. 1993a Diagnosis of membrane fouling using a rotating annular filter. 2. Dilute particle suspension of known particle size. J. Membr. Sci. 77 (1), 23–39.Google Scholar
Belfort, G., Pimbley, J. M., Greiner, A. & Chung, K. Y. 1993b Diagnosis of membrane fouling using a rotating annular filter. 1. Cell culture media. J. Membr. Sci. 77 (1), 1–22.Google Scholar
Bühler, K. 1984 Instabilitaten spiralformiger Strömungen im Zylinderspalt. Z. Angew. Math. Mech. 64 (4), 180–184.Google Scholar
Bühler, K. 1990 Symmetric and asymmetric Taylor vortex flow in spherical gaps. Acta Mechanica 81 (1–2), 3–38.CrossRefGoogle Scholar
Chandrasekhar, S. 1960 The hydrodynamic instability of viscid flow between coaxial cylinders. Proc. Natl Acad. Sci. 46, 141–143.Google Scholar
Chung, K. C. & Astill, K. N. 1977 Hydrodynamic instability of viscous flow between rotating coaxial cylinders with fully developed axial flow. J. Fluid Mech. 81, 641–655.CrossRefGoogle Scholar
Czarny, O., Serre, E., Bontoux, P. & Lueptow, R. M. 2002 Spiral and wavy vortex flows in short counter-rotating Taylor–Couette cells. Theor. Comput. Fluid Dyn. 16 (1), 5–15.Google Scholar
Czarny, O., Serre, E., Bontoux, P. & Lueptow, R. M. 2003 Interaction between Ekman pumping and the centrifugal instability in Taylor–Couette flow. Phys. Fluids 15 (2), 467–477.Google Scholar
Davey, A., DiPrima, R. C. & Stuart, J. T. 1968 On the instability of Taylor vortices. J. Fluid Mech. 31, 17–52.Google Scholar
DiPrima, R. C. 1960 The stability of a viscous fluid between rotating cylinders with an axial flow. J. Fluid Mech. 9, 621–631.CrossRefGoogle Scholar
Donnelly, R. J. & Fultz, D. 1960 Experiments on the stability of spiral flow between rotating cylinders. Proc. Natl Acad. Sci. 46, 1150–1154.CrossRefGoogle ScholarPubMed
Fontaine, G., Poncet, S. & Serre, E. 2014 Multidomain extension of a divergence-free pseudo-spectral algorithm for the direct numerical simulation of wall-confined rotating flows. In Spectral and High Order Methods for Partial Differential Equations – ICOSAHOM 2012, Lecture Notes in Computational Science and Engineering, vol. 95, pp. 261–271. Springer.Google Scholar
Gallet, B., Doering, C. R. & Spiegel, E. A. 2010 Destabilising Taylor–Couette flow with suction. Phys. Fluids 22, 034105.CrossRefGoogle Scholar
Giordano, R. L. C., Giordano, R. C., Prazeres, D. M. F. & Cooney, C. L. 2000 Analysis of a Taylor–Poiseuille vortex flow reactor – II: reactor modeling and performance assessment using glucose–fructose isomerization as test reaction. Chem. Engng Sci. 55, 3611–3626.CrossRefGoogle Scholar
Gravas, N. & Martin, B. W. 1978 Instability of viscous axial flow in annuli having a rotating inner cylinder. J. Fluid Mech. 86, 385–394.Google Scholar
Hallström, B. & Lopez-Leiva, M. 1978 Description of a rotating ultrafiltration module. Desalination 24 (1–3), 273–279.CrossRefGoogle Scholar
Hasoon, M. A. & Martin, B. W. 1977 The stability of a viscous axial flow in an annulus with a rotating inner cylinder. Proc. R. Soc. Lond. A 352, 351–380.Google Scholar
Huerre, P. & Monkewitz, P. A. 1985 Absolute and convective instabilities in free shear layers. J. Fluid Mech. 159, 151–168.Google Scholar
Johnson, E. C. & Lueptow, R. M. 1997 Hydrodynamic stability of flow between rotating porous cylinders with radial and axial flow. Phys. Fluids 9 (12), 3687–3696.Google Scholar
Kaye, J. & Elgar, E. C. 1958 Modes of adiabatic and diabatic fluid flow in an annulus with an inner rotating cylinder. Trans. ASME 80, 753–765.Google Scholar
Kerswell, R. R. 2015 Instability driven by boundary inflow across shear: a way to circumvent Rayleigh’s stability criterion in accretion disks? J. Fluid Mech. 784, 619–663.Google Scholar
Kolyshkin, A. & Vaillancourt, R. 1997 Convective instability boundary of Couette flow between rotating porous cylinder with axial and radial flow. Phys. Fluids 9 (4), 910–918.CrossRefGoogle Scholar
Kroner, K. H. & Nissinen, V. 1988 Dynamic filtration of microbial suspension using an axially rotating filter. J. Membr. Sci. 36, 85–100.Google Scholar
Lueptow, R. M., Docter, A. & Min, K. 1992 Stability of axial flow in an annulus with a rotating inner cylinder. Phys. Fluids A 4 (11), 2446–2455.Google Scholar
Lueptow, R. M. & Hajiloo, A. 1995 Flow in a rotating membrane plasma separator. Trans. Am. Soc. Artif. Intern. Organs 41 (2), 182–188.Google Scholar
Margaritis, A. & Wilke, C. R. 1978 The Rotorfermentor. I. Description of the apparatus, power requirements, and mass transfer characteristics. Biotechnol. Bioengng 20 (5), 709–726.Google Scholar
Martinand, D., Serre, E. & Lueptow, R. M. 2009 Absolute and convective instability of cylindrical Couette flow with axial and radial flows. Phys. Fluids 21, 104102.Google Scholar
Martinand, D., Serre, E. & Lueptow, R. M. 2014 Mechanisms for the transition to waviness for Taylor vortices. Phys. Fluids 26, 094102.CrossRefGoogle Scholar
Min, K. & Lueptow, R. M. 1994a Circular Couette flow with pressure-driven axial flow and a porous inner cylinder. Exp. Fluids 17, 190–197.Google Scholar
Min, K. & Lueptow, R. M. 1994b Hydrodynamic stability of viscous flow between rotating porous cylinders with radial flow. Phys. Fluids 6 (1), 144–151.Google Scholar
Ng, B. S. & Turner, E. R. 1982 On the linear stability of spiral flow between rotating cylinders. Proc. R. Soc. Lond. A 382, 83–102.Google Scholar
Ohashi, K., Tashiro, K., Kushiya, F., Matsumoto, T., Yoshida, S., Endo, M., Horio, T., Osawa, K. & Sakai, K. 1988 Rotation-induced Taylor vortex enhances filtrate flux in plasma separation. Trans. Am. Soc. Artif. Intern. Organs J. 34 (3), 300–307.Google Scholar
Raspo, I., Hughes, S., Serre, E., Randriamampianina, A. & Bontoux, P. 2002 A spectral projection method for the simulation of complex three-dimensional rotating flows. Comput. Fluids 31 (4–7), 745–767.CrossRefGoogle Scholar
Recktenwald, A., Lücke, M. & Müller, H. W. 1993 Taylor vortex formation in axial through-flow: linear and weakly nonlinear analysis. Phys. Rev. E 48 (6), 4444–4454.Google ScholarPubMed
Schwarz, K. W., Springlett, B. E. & Donnelly, R. J. 1964 Modes of instability in spiral flow between rotating cylinders. J. Fluid Mech. 20, 281–289.Google Scholar
Schwille, J. A., Mitra, D. & Lueptow, R. M. 2002 Design parameters for rotating filtration. J. Membr. Sci. 204 (1–2), 53–65.Google Scholar
Serre, E., Sprague, M. A. & Lueptow, R. M. 2008 Stability of Taylor–Couette flow in a finite-length cavity with radial through-flow. Phys. Fluids 20 (3), 034106.Google Scholar
Snyder, H. A. 1962 Experiments on the stability of spiral flow at low axial Reynolds numbers. Proc. R. Soc. Lond. A 265, 198–214.Google Scholar
Snyder, H. A. 1965 Experiments on the stability of two types of spiral flow. Ann. Phys. 31, 292–313.Google Scholar
Sobolik, V., Izrar, B., Lusseyran, F. & Skali, S. 2000 Interaction between the Ekman layer and the Couette–Taylor instability. Intl J. Heat Mass Transfer 43 (24), 4381–4393.Google Scholar
Sorour, M. M. & Coney, J. E. R. 1979 The characteristics of spiral vortex flow at high Taylor numbers. J. Mech. Engng Sci. 21, 65–71.Google Scholar
Syed, A. & Fruh, W. G. 2003 Modelling of mixing in a Taylor–Couette reactor with axial flow. J. Chem. Technol. Biotechnol. 78 (2–3), 227–235.CrossRefGoogle Scholar
Takeuchi, D. I. & Jankowski, D. F. 1981 A numerical and experimental investigation of the stability of spiral Poiseuille flow. J. Fluid Mech. 102, 101–126.Google Scholar
Taylor, G. I. 1923 Stability of a viscous liquid contained between two rotating cylinders. Phil. Trans. R. Soc. Lond. A 223, 289–343.Google Scholar
Tilton, N., Martinand, D., Serre, E. & Lueptow, R. M. 2010 Pressure-driven radial flow in a Taylor–Couette cell. J. Fluid Mech. 660, 527–537.CrossRefGoogle Scholar
Wereley, S. T., Akonur, A. & Lueptow, R. M. 2002 Particle–fluid velocities and fouling in rotating flitration of a suspension. J. Membr. Sci. 209 (2), 469–484.Google Scholar
Wereley, S. T. & Lueptow, R. M. 1999 Velocity field for Taylor–Couette flow with an axial flow. Phys. Fluids 11 (12), 3637–3649.Google Scholar