Hostname: page-component-586b7cd67f-t7fkt Total loading time: 0 Render date: 2024-12-01T01:30:34.918Z Has data issue: false hasContentIssue false
  • English
  • Français

Large-eddy simulation of temporally developing double helical vortices

Published online by Cambridge University Press:  23 January 2019

J.-B. Chapelier Open the ORCID record for J.-B. Chapelier [Opens in a new window] ,
B. Wasistho  and
C. Scalo
J.-B. Chapelier*
Affiliation:
School of Mechanical Engineering, Purdue University, West Lafayette, IN 47907, USA
B. Wasistho
Affiliation:
Kord Technologies, Huntsville, AL 35806, USA
C. Scalo
Affiliation:
School of Mechanical Engineering, Purdue University, West Lafayette, IN 47907, USA
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

This paper investigates the transient regime and turbulent wake characteristics of temporally developing double helical vortices via high-fidelity large-eddy simulation (LES) for circulation Reynolds numbers in the range 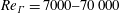$Re_{\unicode[STIX]{x1D6E4}}=7000{-}70\,000$, vortex-core radii between 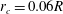$r_{c}=0.06R$ and 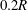$0.2R$ and helical pitches in the range 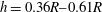$h=0.36R{-}0.61R$, where 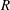$R$ is the initial helix radius. The present study achieves three objectives: (i) assess the influence of 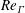$Re_{\unicode[STIX]{x1D6E4}}$, 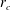$r_{c}$ and $h$ on the growth rates of the helical vortex instability driven by mutual inductance; (ii) characterize the type of vortex reconnection events that appear during transition; (iii) study the characteristics of turbulence in the far wake, and in particular quantify the anisotropy in the flow. The initial transient dynamics is conveniently described in terms of the non-dimensional time 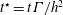$t^{\star }=t\unicode[STIX]{x1D6E4}/h^{2}$, yielding the dimensionless growth rate of 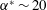$\unicode[STIX]{x1D6FC}^{\ast }\sim 20$ and collapsing of all the LES data for a given 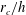$r_{c}/h$ ratio. The vortex-core displacement growth rate is found to be Reynolds-number independent, and decreases for larger 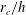$r_{c}/h$ ratios. Several vortex reconnection events are identified during the transition, mostly initiated by the leap frogging of helical vortices. This phenomenon causes the entanglement of orthogonal vortex filaments, leading to their separation, followed by the creation of elongated threads in the axial direction. The turbulent wake generated by the breakdown of the helical vortices is found to be highly anisotropic with the axial fluctuations being dominant compared to the radial and azimuthal fluctuations (near one-dimensional turbulence). The study of integral length scales shows the presence of a strong large-scale anisotropy, retaining the memory of the initial helical pitch $h$, in particular for the integral scale in the axial direction. The large-scale anisotropy is propagated through the inertial and dissipative ranges, determined from the computation of the moments of velocity gradients in the three directions.

JFM classification

Turbulent Flows: Turbulence simulation Vortex Flows: Vortex breakdown Vortex Flows: Vortex dynamics
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 863 , 25 March 2019 , pp. 79 - 113
Copyright
© 2019 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Alekseenko, S. V., Kuibin, P. A., Okulov, V. L. & Shtork, S. I. 1999 Helical vortices in swirl flow. J. Fluid Mech. 382, 195–243.10.1017/S0022112098003772Google Scholar
Bhagwat, M. J. & Leishman, J. G. 2014 Self-induced velocity of a vortex ring using straight-line segmentation. J. Am. Helicopter Soc. 59 (1), 1–7.10.4050/JAHS.59.012004Google Scholar
Boratav, O. N., Pelz, R. B. & Zabusky, N. J. 1992 Reconnection in orthogonally interacting vortex tubes: direct numerical simulations and quantifications. Phys. Fluids 4 (3), 581–605.10.1063/1.858329Google Scholar
Carter, D. W. & Coletti, F. 2017 Scale-to-scale anisotropy in homogeneous turbulence. J. Fluid Mech. 827, 250–284.10.1017/jfm.2017.496Google Scholar
Chapelier, J.-B., Wasistho, B. & Scalo, C. 2018 A coherent vorticity preserving eddy-viscosity correction for large-eddy simulation. J. Comput. Phys. 359, 164–182.10.1016/j.jcp.2018.01.012Google Scholar
Crow, S. C. 1970 Stability theory for a pair of trailing vortices. AIAA J. 8 (12), 2172–2179.10.2514/3.6083Google Scholar
Delbende, I., Piton, B. & Rossi, M. 2015 Merging of two helical vortices. Eur. J. Mech. (B/Fluids) 49, 363–372.10.1016/j.euromechflu.2014.04.005Google Scholar
Delbende, I., Rossi, M. & Daube, O. 2012 DNS of flows with helical symmetry. Theor. Comput. Fluid Dyn. 26 (1–4), 141–160.10.1007/s00162-011-0241-yGoogle Scholar
Dufresne, N. P. & Wosnik, M. 2013 Velocity deficit and swirl in the turbulent wake of a wind turbine. Mar. Tech. Soc. J. 47 (4), 193–205.10.4031/MTSJ.47.4.20Google Scholar
Felli, M., Camussi, R. & Di Felice, F. 2011 Mechanisms of evolution of the propeller wake in the transition and far fields. J. Fluid Mech. 682, 5–53.10.1017/jfm.2011.150Google Scholar
Ganapathisubramani, B., Lakshminarasimhan, K. & Clemens, N. T. 2008 Investigation of three-dimensional structure of fine scales in a turbulent jet by using cinematographic stereoscopic particle image velocimetry. J. Fluid Mech. 598, 141–175.10.1017/S0022112007009706Google Scholar
George, W. K. & Hussein, H. J. 1991 Locally axisymmetric turbulence. J. Fluid Mech. 233, 1–23.10.1017/S0022112091000368Google Scholar
Gupta, B. P. & Loewy, R. G. 1974 Theoretical analysis of the aerodynamic stability of multiple, interdigitated helical vortices. AIAA J. 12 (10), 1381–1387.10.2514/3.49493Google Scholar
Ivanell, S., Mikkelsen, R., Sørensen, J. N. & Henningson, D. 2010 Stability analysis of the tip vortices of a wind turbine. Wind Energy 13 (8), 705–715.10.1002/we.391Google Scholar
Jaque, R. S. & Fuentes, O. V. 2017 Reconnection of orthogonal cylindrical vortices. Eur. J. Mech. (B/Fluids) 62, 51–56.10.1016/j.euromechflu.2016.11.001Google Scholar
Kida, S. & Takaoka, M. 1994 Vortex reconnection. Annu. Rev. Fluid Mech. 26 (1), 169–177.10.1146/annurev.fl.26.010194.001125Google Scholar
Laporte, F. & Corjon, A. 2000 Direct numerical simulations of the elliptic instability of a vortex pair. Phys. Fluids 12 (5), 1016–1031.10.1063/1.870357Google Scholar
Lele, S. K. 1992 Compact finite difference schemes with spectral-like resolution. J. Comput. Phys. 103 (1), 16–42.10.1016/0021-9991(92)90324-RGoogle Scholar
Lesieur, M., Métais, O. & Comte, P. 2005 Large-Eddy Simulations of Turbulence. Cambridge University Press.10.1017/CBO9780511755507Google Scholar
Martin, P. B. & Leishman, J. G. 2003 Trailing vortex measurements in the wake of a hovering rotor blade with various tip shapes. In 58th American Helicopter Society International Annual Forum.Google Scholar
Nagarajan, S., Lele, S. K. & Ferziger, J. H. 2003 A robust high-order compact method for large eddy simulation. J. Comput. Phys. 191, 392–419.10.1016/S0021-9991(03)00322-XGoogle Scholar
Nemes, A., Lo Jacono, D., Blackburn, H. M. & Sheridan, J. 2015 Mutual inductance of two helical vortices. J. Fluid Mech. 774, 298–310.10.1017/jfm.2015.288Google Scholar
Okulov, V. L. 2004 On the stability of multiple helical vortices. J. Fluid Mech. 521, 319–342.10.1017/S0022112004001934Google Scholar
Okulov, V. L., Naumov, I. V., Mikkelsen, R. F., Kabardin, I. K. & Sørensen, J. N. 2014 A regular strouhal number for large-scale instability in the far wake of a rotor. J. Fluid Mech. 747, 369–380.10.1017/jfm.2014.174Google Scholar
Okulov, V. L., Naumov, I. V., Mikkelsen, R. F. & Sørensen, J. N. 2015 Wake effect on a uniform flow behind wind-turbine model. J. Phys.: Conf. Ser. 625, 012011.Google Scholar
Okulov, V. L. & Sørensen, J. N. 2007 Stability of helical tip vortices in a rotor far wake. J. Fluid Mech. 576, 1–25.10.1017/S0022112006004228Google Scholar
Pope, S. B. 2000 Turbulent Flows. Cambridge University Press.10.1017/CBO9780511840531Google Scholar
Quaranta, H. U., Bolnot, H. & Leweke, T. 2015 Long-wave instability of a helical vortex. J. Fluid Mech. 780, 687–716.10.1017/jfm.2015.479Google Scholar
Sherry, M., Nemes, A., Lo Jacono, D., Blackburn, H. M. & Sheridan, J. 2013 The interaction of helical tip and root vortices in a wind turbine wake. Phys. Fluids 25 (11), 117102.10.1063/1.4824734Google Scholar
Simonsen, A. J. & Krogstad, P. 2005 Turbulent stress invariant analysis: clarification of existing terminology. Phys. Fluids 17 (8), 088103.10.1063/1.2009008Google Scholar
Sørensen, J. N., Naumov, I. V. & Okulov, V. L. 2011 Multiple helical modes of vortex breakdown. J. Fluid Mech. 683, 430–441.10.1017/jfm.2011.308Google Scholar
Van Hoydonck, W. R., Bakker, R. J. J. & Van Tooren, M. J. L. 2010 A new method for rotor wake analysis using nurbs primitives. In 36th European Rotorcraft Forum Proceedings, Association Aéronautique et Astronautique de France.Google Scholar
Vatistas, G. H., Kozel, V. & Mih, W. C. 1991 A simpler model for concentrated vortices. Exp. Fluids 11 (1), 73–76.10.1007/BF00198434Google Scholar
Vatistas, G. H., Panagiotakakos, G. D. & Manikis, F. I. 2015 Extension of the n-vortex model to approximate the effects of turbulence. J. Aircraft 52 (5), 1721–1725.10.2514/1.C033238Google Scholar
Walther, J. H., Guenot, M., Machefaux, E., Rasmussen, J. T., Chatelain, P., Okulov, V. L., Sørensen, J. N., Bergdorf, M. & Koumoutsakos, P. 2007 A numerical study of the stabilitiy of helical vortices using vortex methods. J. Phys.: Conf. Ser. 75, 012034.Google Scholar
Widnall, S. E. 1972 The stability of a helical vortex filament. J. Fluid Mech. 54 (4), 641–663.10.1017/S0022112072000928Google Scholar
Zabusky, N. J. & Melander, M. V. 1989 Three-dimensional vortex tube reconnection: morphology for orthogonally-offset tubes. Physica D 37 (1–3), 555–562.Google Scholar