Hostname: page-component-cd9895bd7-q99xh Total loading time: 0 Render date: 2024-12-21T14:50:48.208Z Has data issue: false hasContentIssue false
  • English
  • Français

The effect of viscous relaxation on the spatiotemporal stability of capillary jets

Published online by Cambridge University Press:  02 September 2011

Alejandro Sevilla
Alejandro Sevilla*
Affiliation:
Área de Mecánica de Fluidos, Universidad Carlos III de Madrid, 28911 Leganés, Spain
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

The linear spatiotemporal stability properties of axisymmetric laminar capillary jets with fully developed initial velocity profiles are studied for large values of both the Reynolds number, 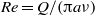, and the Froude number, 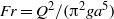, where is the injector radius, 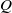the volume flow rate, the kinematic viscosity and the gravitational acceleration. The downstream development of the basic flow and its stability are addressed with an approximate formulation that takes advantage of the jet slenderness. The base flow is seen to depend on two parameters, namely a Stokes number, , and a Weber number, 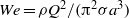, where 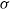is the surface tension coefficient, while its linear stability depends also on the Reynolds number. When non-parallel terms are retained in the local stability problem, the analysis predicts a critical value of the Weber number, 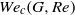, below which a pocket of local absolute instability exists within the near field of the jet. The function 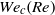is computed for the buoyancy-free jet, showing marked differences with the results previously obtained with uniform velocity profiles. It is seen that, in accounting for gravity effects, it is more convenient to express the parametric dependence of the critical Weber number with use made of the Morton and Bond numbers, 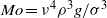and 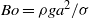, as replacements for 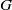and 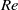. This alternative formulation is advantageous to describe jets of a given liquid for a known value of 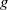, in that the resulting Morton number becomes constant, thereby leaving 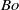as the only relevant parameter. The computed function 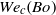for a water jet under Earth gravity is shown to be consistent with the experimental results of Clanet and Lasheras for the transition from jetting to dripping of water jets discharging into air from long injection needles, which cannot be properly described with a uniform velocity profile assumed at the jet exit.

JFM classification

Instability: Absolute/convective instability Interfacial Flows (free surface): Interfacial Flows (free surface) Wakes/Jets: Jets
Type
Papers
Information
Journal of Fluid Mechanics , Volume 684 , 10 October 2011 , pp. 204 - 226
Copyright
Copyright © Cambridge University Press 2011

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

1. Ambravaneswaran, B., Subramani, H. J., Phillips, S. D. & Basaran, O. A. 2004 Dripping–jetting transitions in a dripping faucet. Phys. Rev. Lett. 93, 034501.CrossRefGoogle Scholar
2. Barrero, A. & Loscertales, I. G. 2007 Micro- and nanoparticles via capillary flows. Annu. Rev. Fluid Mech. 39, 89–106.CrossRefGoogle Scholar
3. Basaran, O. A. 2002 Small-scale free surface flows with breakup: drop formation and emerging applications. AIChE J. 48, 1842–1848.CrossRefGoogle Scholar
4. Bogy, D. B. 1979 Drop formation in a circular liquid jet. Annu. Rev. Fluid Mech. 11, 207–228.CrossRefGoogle Scholar
5. Chomaz, J.-M. 2005 Global instabilities in spatially developing flows: non-normality and nonlinearity. Annu. Rev. Fluid Mech. 37, 357–392.CrossRefGoogle Scholar
6. Chomaz, J.-M., Huerre, P. & Redekopp, L. G. 1988 Bifurcation to local and global modes in spatially developing flows. Phys. Rev. Lett. 60, 25–28.CrossRefGoogle ScholarPubMed
7. Clanet, C. & Lasheras, J. C. 1999 Transition from dripping to jetting. J. Fluid Mech. 383, 307–326.Google Scholar
8. Couairon, A. & Chomaz, J.-M. 1999 Fully nonlinear global modes in slowly varying flows. Phys. Fluids 11, 3688–3703.CrossRefGoogle Scholar
9. Deissler, R. J. 1987 The convective nature of instability in plane Poiseuille flow. Phys. Fluids 30 (8), 2303–2305.Google Scholar
10. Duda, J. L. & Vrentas, J. S. 1967 Fluid mechanics of laminar liquid jets. Chem. Engng Sci. 22, 855–869.CrossRefGoogle Scholar
11. Eggers, J. 1997 Nonlinear dynamics and breakup of free surface flows. Rev. Mod. Phys. 69, 865–929.Google Scholar
12. Eggers, J. & Villermaux, E. 2008 Physics of liquid jets. Rep. Prog. Phys. 71, 036601.Google Scholar
13. Gavis, J. 1964 Contribution of surface tension to expansion and contraction of capillary jets. Phys. Fluids 7 (7), 1097–1098.CrossRefGoogle Scholar
14. Gordillo, J. M. & Gekle, S. 2010 Generation and breakup of Worthington jets after cavity collapse. Part 2: tip breakup of stretched jets. J. Fluid Mech. 663, 331–346.Google Scholar
15. Gordillo, J. M. & Pérez-Saborid, M. 2005 Aerodynamic effects in the break-up of liquid jets: on the first wind-induced break-up regime. J. Fluid Mech. 541 (541), 1–20.CrossRefGoogle Scholar
16. Gordillo, J. M., Pérez-Saborid, M. & Ganán-Calvo, A. M. 2001 Linear stability of co-flowing liquid–gas jets. J. Fluid Mech. 448, 23–51.CrossRefGoogle Scholar
17. Goren, S. L. 1966 Development of the boundary layer at a free surface from a uniform shear flow. J. Fluid Mech. 25, 87–95.CrossRefGoogle Scholar
18. Goren, S. L. & Wronski, S. 1966 The shape of low-speed capillary jets of Newtonian liquids. J. Fluid Mech. 25, 185–198.Google Scholar
19. Harmon, D. B. 1955 Drop sizes from low speed jets. J. Franklin Inst. 259 (6), 519–522.CrossRefGoogle Scholar
20. Herbert, T. 1997 Parabolized stability equations. Annu. Rev. Fluid Mech. 29, 245–283.Google Scholar
21. Herrada, M. A., Del Pino, C. & Fernández-Feria, R. 2008 Stability of the boundary layer flow on a long thin rotating cylinder. Phys. Fluids 20, 034105.CrossRefGoogle Scholar
22. Huerre, P. & Monkewitz, P. 1990 Local and global instabilities in spatially developing flows. Annu. Rev. Fluid Mech. 22, 473–537.CrossRefGoogle Scholar
23. Le Dizès, S. 1997 Global modes in falling capillary jets. Eur. J. Mech. (B/Fluids) 16, 761–778.Google Scholar
24. Leib, S. J. & Goldstein, M. E. 1986a Convective and absolute instability of a viscous liquid jet. Phys. Fluids 29 (4), 952–954.CrossRefGoogle Scholar
25. Leib, S. J. & Goldstein, M. E. 1986b The generation of capillary instabilities on a liquid jet. J. Fluid Mech. 168, 479–500.CrossRefGoogle Scholar
26. Lesshafft, L., Huerre, P., Sagaut, P. & Terracol, M. 2006 Nonlinear global modes in hot jets. J. Fluid Mech. 554, 393–409.Google Scholar
27. Lin, S. P. & Reitz, R. D. 1998 Drop and spray formation from a liquid jet. Annu. Rev. Fluid Mech. 30, 85–105.CrossRefGoogle Scholar
28. Miles, J. W. 1960 The hydrodynamic stability of a thin film of liquid in uniform shearing motion. J. Fluid Mech. 8, 593–610.CrossRefGoogle Scholar
29. Monkewitz, P. A., Davis, J., Bojorquez, B. & Yu, M.-H. 1988 The breakup of a liquid jet at high Weber number. Bull. Am. Phys. Soc. 33, 2273.Google Scholar
30. Monkewitz, P. A., Huerre, P. & Chomaz, J.-M. 1993 Global linear stability analysis of weakly non-parallel shear flows. J. Fluid Mech. 251, 1–20.Google Scholar
31. Og˜uz, H. 1998 On the relaxation of laminar jets at high Reynolds numbers. Phys. Fluids 10 (2), 361–367.Google Scholar
32. Og˜uz, H. & Prosperetti, A. 1993 Dynamics of bubble growth and detachment from a needle. J. Fluid Mech. 257, 111–145.CrossRefGoogle Scholar
33. O’Donnell, B., Chen, J. N. & Lin, S. P. 2001 Transition from convective to absolute instability in a liquid jet. Phys. Fluids 13 (9), 2732–2734.Google Scholar
34. Osborne, B. P. & Steinberg, T. A. 2006 An experimental investigation into liquid jetting modes and break-up mechanisms conducted with a new reduced gravity facility. Microgravity Sci. Technol. XVIII (3/4), 57–61.CrossRefGoogle Scholar
35. Philippe, C. & Dumargue, P. 1991 Etude de l’etablissement d’un jet liquide laminaire emergeant d’une conduite cylindrique verticale semi-infinie et soumis a l’influence de la gravite. Z. Angew. Math. Phys. 42, 227–242.CrossRefGoogle Scholar
36. Pier, B. & Huerre, P. 2001 Nonlinear self-sustained structures and fronts in spatially developing wake flows. J. Fluid Mech. 145, 145–174.CrossRefGoogle Scholar
37. Pier, B., Huerre, P., Chomaz, J.-M. & Couairon, A. 1998 Steep nonlinear global modes in spatially developing media. Phys. Fluids 10 (10), 2433–2435.Google Scholar
38. Rayleigh, W. S. 1878 On the instability of jets. Proc. Lond. Math. Soc. 10, 4–13.CrossRefGoogle Scholar
39. Sauter, U. S. & Buggisch, H. W. 2005 Stability of initially slow viscous jets driven by gravity. J. Fluid Mech. 533, 237–257.Google Scholar
40. Sevilla, A., Gordillo, J. M. & Martínez-Bazán, C. 2005 Transition from bubbling to jetting in a coaxial air–water jet. Phys. Fluids 17, 018105.CrossRefGoogle Scholar
41. Sevilla, A. & Martínez-Bazán, C. 2004 Vortex shedding in high Reynolds number axisymmetric bluff-body wakes: local linear instability and global bleed control. Phys. Fluids 16, 3460.Google Scholar
42. Smith, M. K. & Davis, S. H. 1982 The instability of sheared liquid layers. J. Fluid Mech. 121, 187–206.CrossRefGoogle Scholar
43. Smith, S. W. J. & Moss, H. 1917 Experiments with mercury jets. Proc. R. Soc. A 93, 373–393.Google Scholar
44. Söderberg, L. D. 2003 Absolute and convective instability of a relaxational plane liquid jet. J. Fluid Mech. 493, 89–119.CrossRefGoogle Scholar
45. Theofilis, V. 2011 Global linear instability. Annu. Rev. Fluid Mech. 43, 319–352.Google Scholar
46. Tillett, J. P. K. 1968 On the laminar flow in a free jet of liquid at high Reynolds number. J. Fluid Mech. 32, 273–292.Google Scholar
47. Utada, A. S., Chu, L.-Y., Fernandez-Nieves, A., Link, D. R., Holtze, C. & Weitz, D. A. 2007 Dripping, jetting, drops, and wetting: the magic of microfluidics. MRS Bull. 32, 702–708.CrossRefGoogle Scholar
48. Vihinen, I., Honohan, A. M. & Lin, S. P. 1997 Image of absolute instability in a liquid jet. Phys. Fluids 9 (11), 3117–3119.Google Scholar
49. Weber, C. 1931 On the breakdown of a fluid jet. Z. Angew. Math. Mech. 11, 136–141.CrossRefGoogle Scholar
50. Wilkes, E. D., Phillips, S. D. & Basaran, O. A. 1999 Computational and experimental analysis of dynamics of drop formation. Phys. Fluids 11 (12), 3577–3598.CrossRefGoogle Scholar