Hostname: page-component-586b7cd67f-2plfb Total loading time: 0 Render date: 2024-12-01T00:33:37.528Z Has data issue: false hasContentIssue false
  • English
  • Français

Effect of the eigenvalues of the velocity gradient tensor on particle collisions

Published online by Cambridge University Press:  29 February 2016

Vincent E. Perrin  and
Harmen J. J. Jonker
Vincent E. Perrin*
Affiliation:
Geoscience and Remote Sensing, Delft University of Technology, 2628 CN Delft, The Netherlands
Harmen J. J. Jonker
Affiliation:
Geoscience and Remote Sensing, Delft University of Technology, 2628 CN Delft, The Netherlands
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

This study uses the eigenvalues of the local velocity gradient tensor to categorize the local flow structures in incompressible turbulent flows into different types of saddle nodes and vortices and investigates their effect on the local collision kernel of heavy particles. Direct numerical simulation (DNS) results show that most of the collisions occur in converging regions with real and negative eigenvalues. Those regions are associated not only with a stronger preferential clustering of particles, but also with a relatively higher collision kernel. To better understand the DNS results, a conceptual framework is developed to compute the collision kernel of individual flow structures. Converging regions, where two out of three eigenvalues are negative, posses a very high collision kernel, as long as a critical amount of rotation is not exceeded. Diverging regions, where two out of three eigenvalues are positive, have a very low collision kernel, which is governed by the third and negative eigenvalue. This model is not suited for particles with Stokes number 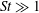$St\gg 1$, where the contribution of particle collisions from caustics is dominant.

JFM classification

Multiphase and Particle-laden Flows: Particle/fluid flow Mathematical Foundations: Topological fluid dynamics
Type
Papers
Information
Journal of Fluid Mechanics , Volume 792 , 10 April 2016 , pp. 36 - 49
Check for updates
Copyright
© 2016 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Ashurst, Wm. T., Kerstein, A. R., Kerr, R. M. & Gibson, C. H. 1987 Alignment of vorticity and scalar gradient with strain rate in simulated Navier–Stokes turbulence. Phys. Fluids 30 (8), 2343.Google Scholar
Bec, J., Homann, H. & Ray, S. S. 2014 Gravity-driven enhancement of heavy particle clustering in turbulent flow. Phys. Rev. Lett. 112 (18), 184501.CrossRefGoogle ScholarPubMed
Bijlard, M. J., Oliemans, R. V. A., Portela, L. M. & Ooms, G. 2010 Direct numerical simulation analysis of local flow topology in a particle-laden turbulent channel flow. J. Fluid Mech. 653, 35–56.Google Scholar
Blackburn, H. M., Mansour, N. N. & Cantwell, B. J. 1996 Topology of fine-scale motions in turbulent channel flow. J. Fluid Mech. 310, 269–292.Google Scholar
Canuto, C. G., Hussaini, M. Y. & Quarteroni, A. 2007 Spectral Methods: Evolution to Complex Geometries and Applications to Fluid Dynamics. Springer.CrossRefGoogle Scholar
Chen, M., Kontomaris, K. & McLaughlin, J. B. 1998 Direct numerical simulation of droplet collisions in a turbulent channel flow. Part I: collision algorithm. Intl J. Multiphase Flow 24 (7), 1079–1103.Google Scholar
Chong, M. S., Perry, A. E. & Cantwell, B. J. 1990 A general classification of three-dimensional flow fields. Phys. Fluids A 2 (5), 765.Google Scholar
Eaton, J. K. & Fessler, J. R. 1994 Preferential concentration of particles by turbulence. Intl J. Multiphase Flow 20, 169–209.Google Scholar
Elsinga, G. E. & Marusic, I. 2010 Universal aspects of small-scale motions in turbulence. J. Fluid Mech. 662 (September), 514–539.CrossRefGoogle Scholar
Falkovich, G., Fouxon, A. & Stepanov, M. G. 2002 Acceleration of rain initiation by cloud turbulence. Nature 419 (6903), 151–154.Google Scholar
Gatignol, R. 1983 The Faxén formulas for a rigid particle in an unsteady non-uniform Stokes-flow. J. Méc. Théor. Appl. 2 (2), 143–160.Google Scholar
Gustavsson, K., Vajedi, S. & Mehlig, B. 2014 Clustering of particles falling in a turbulent flow. Phys. Rev. Lett. 112 (21), 214501.Google Scholar
Hunt, J. C. R., Wray, A. A. & Moin, P. 1988 Eddies, streams, and convergence zones in turbulent flows. In Proceedings of FEDSM 2006 ASME JOINT US European Fluids Engineering Summer Meeting, Miami, FL, USA, 2006 July 17–20, pp. 193–208.Google Scholar
Maxey, M. R. 1987 The gravitational settling of aerosol particles in homogeneous turbulence and random flow fields. J. Fluid Mech. 174, 441.Google Scholar
Maxey, M. R. & Riley, J. J. 1983 Equation of motion for a small rigid sphere in a nonuniform flow. Phys. Fluids 26 (4), 883–889.Google Scholar
Perrin, V. E. & Jonker, H. J. J. 2014 Preferred location of droplet collisions in turbulent flows. Phys. Rev. E 89 (3), 33005.Google Scholar
Reeks, M. W., Fabbro, L. & Soldati, A. 2006 In search of random uncorrelated particle motion (RUM) in a simple random flow field. In …2nd Joint US- …, p. 8.Google Scholar
Rouson, D. W. I. & Eaton, J. K. 2001 On the preferential concentration of solid particles in turbulent channel flow. J. Fluid Mech. 428, 149–169.CrossRefGoogle Scholar
Shaw, R. A., Reade, W. C., Collins, L. R. & Verlinde, J. 1998 Preferential concentration of cloud droplets by turbulence: effects on the early evolution of cumulus cloud droplet spectra. J. Atmos. Sci. 55 (11), 1965–1976.Google Scholar
Squires, K. D. & Eaton, J. K. 1991 Preferential concentration of particles by turbulence. Phys. Fluids A 3 (5), 1169.Google Scholar
Sundaram, S. & Collins, L. R. 1997 Collision statistics in an isotropic particle-laden turbulent suspension. Part 1. Direct numerical simulations. J. Fluid Mech. 335, 75–109.Google Scholar
Voßkuhle, M., Pumir, A., Lévêque, E. & Wilkinson, M. 2014 Prevalence of the sling effect for enhancing collision rates in turbulent suspensions. J. Fluid Mech. 749, 841–852.Google Scholar
Wang, L. P., Wexler, A. S. & Zhou, Y. 2000 Statistical mechanical description and modelling of turbulent collision of inertial particles. J. Fluid Mech. 415, 117–153.Google Scholar
Wilkinson, M. & Mehlig, B. 2005 Caustics in turbulent aerosols. Europhys. Lett. 71 (2), 186.Google Scholar
Woittiez, E. J. P., Jonker, H. J. J. & Portela, L. M. 2009 On the combined effects of turbulence and gravity on droplet collisions in clouds: a numerical study. J. Atmos. Sci. 66 (7), 1926–1943.CrossRefGoogle Scholar
Zhou, J., Adrian, R. J. & Balachandar, S. 1996 Autogeneration of near-wall vortical structures in channel flow. Phys. Fluids 8 (1), 288.Google Scholar
Zhou, J., Adrian, R. J., Balachandar, S. & Kendall, T. M. 1999 Mechanisms for generating coherent packets of hairpin vortices in channel flow. J. Fluid Mech. 387, 353–396.Google Scholar