Hostname: page-component-cd9895bd7-mkpzs Total loading time: 0 Render date: 2024-12-19T13:45:46.915Z Has data issue: false hasContentIssue false
  • English
  • Français

Counter-hairpin vortices in the turbulent wake of a sharp trailing edge

Published online by Cambridge University Press:  28 November 2011

Sina Ghaemi  and
Fulvio Scarano
Sina Ghaemi*
Affiliation:
Department of Aerodynamics, Delft University of Technology, Kluyverweg 1, 2629 HS, Delft, The Netherlands
Fulvio Scarano
Affiliation:
Department of Aerodynamics, Delft University of Technology, Kluyverweg 1, 2629 HS, Delft, The Netherlands
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

The unsteady organization and evolution of coherent structures within the turbulent boundary layer and subsequent wake of the sharp symmetric trailing edge of a NACA0012 aerofoil are investigated. The experiments are conducted in an open test-section wind tunnel at based on the aerofoil chord and 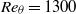based on the boundary layer momentum thickness. An initial characterization of the flow field using two-component particle image velocimetry (PIV) is followed by the investigation of the unsteady organization and evolution of coherent structures by time-resolved three-dimensional PIV based on a tomographic approach (Tomo-PIV). The inspection of the turbulent boundary layer prior to the trailing edge in the region between 0.15 and 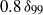demonstrated streaks of low- and high-speed flow, while the low-speed streaks are observed to be more coherent along with strong interaction with hairpin-type vortical structures similar to a turbulent boundary layer at zero pressure gradient. The wake region demonstrated gradual deterioration of both the low- and the high-speed streaks with downstream progress. However, the low-speed streaks are observed to lose their coherence at a faster rate relative to the high-speed streaks as the turbulent flow develops towards the far wake. The weakening of the low-speed streaks is due to the disappearance of the viscous sublayer after the trailing edge and gradual mixing through the transport of the remaining low-speed flow towards the free stream. This transport of low-speed flow is performed by the ejection events induced by the hairpin vortices as they also persist into the developing wake. The higher persistence of the high-speed streaks is associated with counter-hairpin vortical activities as they oppose the deterioration of the high-speed streaks by frequently sweeping the high-speed flow towards the wake centreline. These vortical structures are regarded as counter-hairpin vortices as they exhibit opposite characteristics relative to the hairpin vortices of a turbulent boundary layer. They are topologically similar to the hairpins as they appear to be U-shaped but with inverted orientation, as the spanwise portion is in the vicinity of the wake centreline and the legs are inclined at an approximately 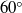to the wake axis in the downstream direction demonstrating a strain-dominated topology. The counter-hairpin vortices are partially wrapped around the high-speed streaks and contribute to the wake development by transporting high-speed flow towards the wake centreline. Similar to the hairpin vortices of a turbulent boundary layer, the occurrence of a complete counter-hairpin vortex is occasional while its derivatives (portions of spanwise or quasi-streamwise vortices) are more frequently observed. Therefore, a pattern recognition algorithm is applied to establish characterization based on an ensemble-averaged counter-hairpin vortex. The formation of the counter-hairpin vortices is due to an additional degree of interaction between the low- and high-speed streaks after the trailing edge across the wake centreline. The shear layer produced along the wake centreline by neighbouring low- and high-speed streaks promotes the formation of spanwise vortices that form the counter-hairpin vortices by connection to quasi-streamwise vortices. Finally, a conceptual model is proposed to depict the three-dimensional unsteady organization and evolution of coherent structures in the wake region based on the hairpin and counter-hairpin vortex signatures.

JFM classification

Boundary Layers: Boundary layer structure Boundary Layers: Free shear layers Turbulent Flows: Turbulent boundary layers
Type
Papers
Information
Journal of Fluid Mechanics , Volume 689 , 25 December 2011 , pp. 317 - 356
Copyright
Copyright © Cambridge University Press 2011

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

1. Adrian, R. J. 2007 Hairpin vortex organization in wall turbulence. Phys. Fluids 19, 041301.CrossRefGoogle Scholar
2. Adrian, R. J. & Liu, Z.-C. 2002 Observation of vortex packets in direct numerical simulation of fully turbulent channel flow. J Vis. 5, 9–19.CrossRefGoogle Scholar
3. Adrian, R. J., Meinhart, C. D. & Tomkins, C. D. 2000 Vortex organization in the outer region of the turbulent boundary layer. J. Fluid Mech. 422, 1–54.CrossRefGoogle Scholar
4. Andreopoulos, J. & Bradshaw, P. 1980 Measurements of interacting turbulent shear layers in the near wake of a flat plate. J. Fluid Mech. 100, 639–668.CrossRefGoogle Scholar
5. Blackwelder, R. F. & Eckelmann, H. 1979 Streamwise vortices associated with the bursting phenomenon. J. Fluid Mech. 94, 577–594.CrossRefGoogle Scholar
6. Blake, W. K. 1986 Mechanics of Flow Induced Sound and Vibration, vols. I and II. Academic.Google Scholar
7. Bogucz, E. A. & Walker, J. D. A. 1988 The turbulent near wake at a sharp trailing-edge. J. Fluid Mech. 196, 555–584.CrossRefGoogle Scholar
8. Brooks, T. F. & Hodgson, T. H. 1981 Trailing edge noise prediction from measured surface pressures. J. Sound Vib. 78, 69–117.CrossRefGoogle Scholar
9. Brooks, T. F., Pope, D. S. & Marcolini, M. A. 1989 Aerofoil Self-Noise and Production. 1218. NASA Reference Publication.Google Scholar
10. Brouwer, H. H. & Rienstra, S. W. 2008 Aeroacoustics research in Europe: the CEAS–ASC Report on 2007 highlights. J. Sound Vib. 318, 625–654.CrossRefGoogle Scholar
11. Carlier, J. & Stanislas, M. 2005 Experimental study of eddy structures in a turbulent boundary layer using particle image velocimetry. J. Fluid Mech. 535, 143–188.CrossRefGoogle Scholar
12. Chevray, R. & Kovasznay, L. S. G. 1969 Turbulence measurements in the wake of a thin flat plate. AIAA J. 8, 1641–1643.CrossRefGoogle Scholar
13. Corino, E. R. & Brodkey, R. S. 1969 A visual investigation of the wall region in turbulent flow. J. Fluid Mech. 37, 1–30.CrossRefGoogle Scholar
14. Darmofal, D. L. 1993 The role of vorticity dynamics in vortex breakdown. AIAA 24th Fluid Dynamics Conference, Orlando, FL, AIAA 93-3036.Google Scholar
15. Dean, R. B. & Bradshaw, P. 1976 Measurements of interacting turbulent shear layers in a duct. J. Fluid Mech. 78, 641–676.CrossRefGoogle Scholar
16. Elsinga, G. E. 2008 Tomographic particle image velocimetry and its application to turbulent boundary layers, PhD thesis, Delft University of Technology.Google Scholar
17. Elsinga, G. E., Adrian, R. J., Van Oudheusden, B. W. & Scarano, F. 2010 Three-dimensional vortex organization in a high-Reynolds-number supersonic turbulent boundary layer. J. Fluid Mech. 644, 35–60.CrossRefGoogle Scholar
18. Elsinga, G. E., Scarano, F., Wieneke, B. & Van Oudheusden, B. W. 2006 Tomographic particle image velocimetry. Exp. Fluids 41, 933–947.CrossRefGoogle Scholar
19. Ferré, J. A. & Giralt, F. 1989 Pattern-recognition analysis of the velocity field in plane turbulent wakes. J. Fluid Mech. 198, 27–64.CrossRefGoogle Scholar
20. Ffowcs Williams, J. E. & Hall, L. H. 1970 Aerodynamic sound generation by turbulent flow in the vicinity of a scattering half plane. J. Fluid Mech. 40, 657–670.CrossRefGoogle Scholar
21. Finez, A., Jondeau, E., Roger, M. & Jacob, M. C. 2010 Broadband noise reduction with trailing edge brushes. 16th AIAA/CEAS Aeroacoustics Conference, Stockholm, Sweden, AIAA 2010-3980.Google Scholar
22. Ganapathisubramani, B., Longmire, E. & Marusic, I. 2003 Characteristics of vortex packets in turbulent boundary layers. J. Fluid Mech. 478, 35–46.CrossRefGoogle Scholar
23. Ghaemi, S. & Scarano, F. 2010 Multi-pass light amplification for tomographic particle image velocimetry applications. Meas. Sci. Technol. 22, 5.Google Scholar
24. Guala, M., Hommema, S. & Adrian, R. 2006 Large scale and very large scale motions in turbulent pipe flows. J. Fluid Mech. 554, 521–542.CrossRefGoogle Scholar
25. Guezennec, Y. G., Piomelli, U. & Kim, J. 1989 On the shape and dynamics of wall structures in turbulent channel flow. Phys. Fluids A 1, 764–766.CrossRefGoogle Scholar
26. Haji-Haidari, A. & Smith, C. R. 1988 Development of the turbulent near wake of a tapered thick flat plate. J. Fluid Mech. 189, 135–163.CrossRefGoogle Scholar
27. Hamelin, J. & Alving, A. E. 1996 A low-shear turbulent boundary layer. Phys. Fluids 8 (3), 789–804.CrossRefGoogle Scholar
28. Hayakawa, M. & Hussain, F. 1989 Three-dimensionality of organized structures in a plane turbulent wake. J. Fluid Mech. 206, 375–404.CrossRefGoogle Scholar
29. Hayakawa, M. & Iida, S. I. 1992 Behavior of turbulence in the near wake of a thin flat plate at low Reynolds numbers. Phys. Fluids A 4 (10), 2282–2291.CrossRefGoogle Scholar
30. Head, M. R. & Bandyopadhyay, P. 1981 New aspects of turbulent boundary-layer structure. J. Fluid Mech. 107, 297–338.CrossRefGoogle Scholar
31. Hebbar, K. S. 1986 Mean and turbulence measurements in the boundary layer and wake of a symmetric aerofoil. Exp. Fluids 4, 214–222.CrossRefGoogle Scholar
32. Herman, G. T. & Lent, A. 1976 Iterative reconstruction algorithms. Comput. Biol. Med. 6, 273–294.CrossRefGoogle ScholarPubMed
33. Herpin, S., Wong, C. Y., Stanislas, M. & Soria, J. 2008 Stereoscopic PIV measurements of a turbulent boundary layer with a large spatial dynamic range. Exp. Fluids 45, 745–763.CrossRefGoogle Scholar
34. Humble, R. A., Elsinga, G. E., Scarano, F. & Van Oudheusden, B. W. 2009 Three-dimensional unsteady flow organization of a shock wave/turbulent boundary layer interaction. J. Fluid Mech. 622, 33–62.CrossRefGoogle Scholar
35. Hunt, J. C. R., Wray, A. A. & Moin, P. 1988 Eddies, stream, and convergence zones in turbulent flows. Research Report CTR-S88, pp. 193–208. Center for Turbulence.Google Scholar
36. Hussain, A. K. M. F. 1986 Coherent structures and turbulence. J. Fluid Mech. 173, 303–356.CrossRefGoogle Scholar
37. Hussain, F. & Hayakawa, M. 1987 Education of large-scale organized structures in a turbulent plane wake. J. Fluid Mech. 180, 193–229.CrossRefGoogle Scholar
38. Hutchins, N. & Marusic, I. 2007 Evidence of very long meandering features in the logarithmic region of turbulent boundary layers. J. Fluid Mech. 579, 1–28.CrossRefGoogle Scholar
39. Kline, S. J., Reynolds, W. C., Schraub, F. A. & Runstadler, P. W. 1967 The structure of turbulent boundary layers. J. Fluid Mech. 30, 741–773.CrossRefGoogle Scholar
40. Kundu, P. K. & Cohen, I. M. 2004 Fluid Mechanics, 3rd edn. Elsevier.Google Scholar
41. Marsden, A. L., Wang, M., Dennis, J. E. & Moin, P. 2007 Trailing-edge noise reduction using derivative-free optimization and large-eddy simulation. J. Fluid Mech. 572, 13–36.CrossRefGoogle Scholar
42. Meinhart, C. D. & Adrian, R. J. 1995 On the existence of uniform momentum zones in a turbulent boundary layer. Phys. Fluids 7 (4), 694–696.CrossRefGoogle Scholar
43. Meinhart, C. D., Wereley, S. T. & Santiago, J. G. 2000 A PIV algorithm for estimating time-averaged velocity fields. Trans. ASME: J. Fluids Engng 122, 285.Google Scholar
44. Miau, J. & Karlsson, S. K. F. 1987 Flow structures in the developing region of a symmetric wake and an unsymmetric wake. Phys. Fluids 30 (8), 2389–2399.CrossRefGoogle Scholar
45. Morris, S. C. & Foss, J. F. 2003 Boundary layer to shear layer – the transitional region. J. Fluid Mech. 494, 187–221.CrossRefGoogle Scholar
46. Nakayama, A. & Liu, B. 1990 The turbulent near wake of a flat plate at low Reynolds number. J. Fluid Mech. 217, 93–114.CrossRefGoogle Scholar
47. Nash, E. C., Lowson, M. V. & McAlpine, A. 1999 Boundary-layer instability noise of aerofoils. J. Fluid Mech. 382, 27–61.CrossRefGoogle Scholar
48. Perry, A. E. & Chong, M. S. 1982 On the mechanism of wall turbulence. J. Fluid Mech. 119, 173–217.CrossRefGoogle Scholar
49. Pope, S. B. & Whitelaw, J. H. 1976 The calculation of near-wake flows. J. Fluid Mech. 73, 9–32.CrossRefGoogle Scholar
50. Ramaprian, B. R., Patel, V. C. & Sastry, M. S. 1982 The symmetric turbulent wake of a flat plate. AIAA J. 20 (9), 1228–1235.CrossRefGoogle Scholar
51. Robin, S. K. 1991 Coherent motions in the turbulent boundary layer. Annu. Rev. Fluid Mech. 23, 601–639.CrossRefGoogle Scholar
52. Robinson, J. L. 1969 Similarity solutions in several turbulent shear flows. Report 1242. National Physical Laboratory, Teddington, UK.Google Scholar
53. Sagrado, A. G., Hynes, T. & Hodson, H. 2006 Experimental investigation into trailing edge noise sources. AIAA Paper 2006-2476.Google Scholar
54. Sandberg, R. D. & Sandham, N. D. 2008 Direct numerical simulation of turbulent flow past a trailing edge and the associated noise generation. J. Fluid Mech. 596, 353–385.CrossRefGoogle Scholar
55. Scarano, F., Benocci, C. & Riethmuller, M. L. 1999 Pattern recognition analysis of the turbulent flow past a backward facing step. Phys. Fluids 11 (12), 3808–3818.CrossRefGoogle Scholar
56. Scarano, F. & Moore, P. 2011 An advection-based model to increase the temporal resolution of PIV time series. Exp. Fluids doi:10.1007/s00348-011-1158-3.Google ScholarPubMed
57. Scarano, F. & Poelma, C. 2009 Three-dimensional vorticity patterns of cylinder wakes. Exp. Fluids 47, 69–83.CrossRefGoogle Scholar
58. Scarano, F. & Reithmuller, M. L. 2000 Advances in iterative multigrid PIV image processing. Exp. Fluids 29, 51–60.CrossRefGoogle Scholar
59. Schoppa, W. & Hussain, F. 2000 Coherent structure dynamics in near-wall turbulence. Fluid Dyn. Res. 26, 119–139.CrossRefGoogle Scholar
60. Schröder, A., Geisler, R., Elsinga, G. E., Scarano, F & Dierksheide, U. 2008 Investigation of a turbulent spot and tripped turbulent boundary layer flow using time-resolved tomographic PIV. Exp. Fluids 44, 305–316.CrossRefGoogle Scholar
61. Scott, C. M. & Foss, J. F. 2003 Turbulent boundary layer to single-stream shear layer: the transition region. J. Fluid Mech. 494, 187–221.Google Scholar
62. Sharland, I. J. 1964 Sources of noise in axial flow fans. J. Sound Vib. 1 (3), 302–322.CrossRefGoogle Scholar
63. Smith, C. R. & Metzler, S. P. 1983 The characteristics of low-speed streaks in the near-wall region of a turbulent boundary layer. J. Fluid Mech. 129, 27–54.CrossRefGoogle Scholar
64. Soloff, S. M., Adrian, R. J. & Liu, Z. C. 1997 Distortion compensation for generalized stereoscopic particle image velocimetry. Meas. Sci. Technol. 8, 1441–1454.CrossRefGoogle Scholar
65. Spalart, P. 1988 Direct simulation of a turbulent boundary layer up to . J. Fluid Mech. 187, 61–98.CrossRefGoogle Scholar
66. Stanislas, M., Perret, L. & Foucaut, J.-M. 2008 Vortical structures in the turbulent boundary layer: a possible route to a universal representation. J. Fluid Mech. 602, 327–382.CrossRefGoogle Scholar
67. Tam, C. K. W. 1974 Discrete tones of isolated aerofoils. J. Acoust. Soc. Am. 55, 1173–1177.CrossRefGoogle Scholar
68. Tardu, S. 1995 Characteristics of single and clusters of bursting events in the inner layer, Part 1: VITA events. Exp. Fluids 20, 112.Google Scholar
69. Theodorsen, T. 1952 Mechanism of turbulence. In Proceedings of the 2nd Midwestern Conference on Fluid Mechanics, Ohio State University, Columbus, OH, pp. 1–19.Google Scholar
70. Tomkins, C. D. & Adrian, R. J. 2003 Spanwise structure and scale growth in turbulent boundary layers. J. Fluid Mech. 490, 37–74.CrossRefGoogle Scholar
71. Townsend, A. A. 1965 Self-preserving flow inside a turbulent boundary layer. J. Fluid Mech. 22, 773–797.CrossRefGoogle Scholar
72. Townsend, A. A. 1966 The flow in a turbulent boundary layer after a change in surface roughness. J. Fluid Mech. 26, 255–266.CrossRefGoogle Scholar
73. Tummers, M. J. 1999 Investigation of a turbulent wake in an adverse pressure gradient using laser Doppler anemometry, PhD thesis, Faculty of Aerospace Engineering, Delft University of Technology, The Netherlands.Google Scholar
74. Wallace, J. M., Brodkey, R. S. & Eckelmann, H. 1997 Pattern-recognized structures in bounded turbulent shear flows. J. Fluid Mech. 83, 673–693.CrossRefGoogle Scholar
75. Wallace, J. M., Eckelmann, H. & Brodkey, R. S. 1972 The wall region in turbulent shear flow. J. Fluid Mech. 54, 39–48.CrossRefGoogle Scholar
76. Westerweel, J. 1997 Fundamentals of digital particle image velocimetry. Meas. Sci. Technol. 8, 1379–1392.CrossRefGoogle Scholar
77. White, F. M. 1974 Viscous Fluid Flow. McGraw-Hill.Google Scholar
78. Wieneke, B. 2008 Volume self-calibration for 3D particle image velocimetry. Exp. Fluids 45, 549–556.CrossRefGoogle Scholar
79. Williamson, C. H. K. 1996 Vortex dynamics in the cylinder wake. Annu. Rev. Fluid Mech. 28, 477–539.CrossRefGoogle Scholar
80. Wygnanski, I., Champagne, F. & Marsali, B. 1986 On the large-scale structures in two-dimensional, small-deficit, turbulent wakes. J. Fluid Mech. 168, 31–71.CrossRefGoogle Scholar
81. Zhou, J., Adrian, R. J., Balachandra, S. & Kendall, T. M. 1999 Mechanisms for generating coherent packets of hairpin vortices in channel flow. J. Fluid Mech. 387, 353–396.CrossRefGoogle Scholar

Ghaemi and Scarano supplementary material

Movie 1. Time-resolved evolution of the low and high speed streaks and the vortical structures in the turbulent boundary layer upstream of the trailing-edge at Reθ= 1300 measured using the Tomo-PIV technique. The original measurement frequency of 2700 Hz has been increased in this movie to 27000 Hz using the advection equation following Scarano & Moore (2011). The visualization shows significant accumulation of the vortical structures around the low speed streaks.

Download Ghaemi and Scarano supplementary material(Video)
Video 13.3 MB

Ghaemi and Scarano supplementary material

Movie 1. Time-resolved evolution of the low and high speed streaks and the vortical structures in the turbulent boundary layer upstream of the trailing-edge at Reθ= 1300 measured using the Tomo-PIV technique. The original measurement frequency of 2700 Hz has been increased in this movie to 27000 Hz using the advection equation following Scarano & Moore (2011). The visualization shows significant accumulation of the vortical structures around the low speed streaks.

Download Ghaemi and Scarano supplementary material(Video)
Video 12.1 MB

Ghaemi and Scarano supplementary material

Movie 2. Time-resolved Tomo-PIV visualization of the low and high speed streaks and the vortical structures in the immediate wake of the sharp symmetric trailing-edge of NACA 0012 at Reθ= 1300. The temporal resolution has been increased in this movie to 27000 Hz using the advection equation following Scarano & Moore (2011). Vortical activities are observed around both the low and the high speed streaks.

Download Ghaemi and Scarano supplementary material(Video)
Video 14.6 MB

Ghaemi and Scarano supplementary material

Movie 2. Time-resolved Tomo-PIV visualization of the low and high speed streaks and the vortical structures in the immediate wake of the sharp symmetric trailing-edge of NACA 0012 at Reθ= 1300. The temporal resolution has been increased in this movie to 27000 Hz using the advection equation following Scarano & Moore (2011). Vortical activities are observed around both the low and the high speed streaks.

Download Ghaemi and Scarano supplementary material(Video)
Video 12.5 MB