Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Tallis, G. M.
and
Chesson, P.
1982.
Identifiability of mixtures.
Journal of the Australian Mathematical Society. Series A. Pure Mathematics and Statistics,
Vol. 32,
Issue. 3,
p.
339.
1983.
Nonparametric Functional Estimation.
p.
483.
1992.
Identifiability in Stochastic Models.
p.
229.
Van Zandt, Trisha
and
Ratcliff, Roger
1995.
Statistical mimicking of reaction time data: Single-process models, parameter variability, and mixtures.
Psychonomic Bulletin & Review,
Vol. 2,
Issue. 1,
p.
20.
Sapatinas, Theofanis
1995.
Identifiability of mixtures of power-series distributions and related characterizations.
Annals of the Institute of Statistical Mathematics,
Vol. 47,
Issue. 3,
p.
447.
Korolev, V. Yu.
1995.
Convergence of Random Sequences with the Independent Random Indices I.
Theory of Probability & Its Applications,
Vol. 39,
Issue. 2,
p.
282.
Butler, Steven M.
and
Louis, Thomas A.
1997.
Consistency of maximum likelihood estimators in general random effects models for binary data.
The Annals of Statistics,
Vol. 25,
Issue. 1,
Al-Hussaini, Essam K.
and
Sultan, Khalaf S.
2001.
Advances in Reliability.
Vol. 20,
Issue. ,
p.
139.
Everitt, B.S.
2004.
Encyclopedia of Statistical Sciences.
Everitt, B. S.
2005.
Encyclopedia of Statistical Sciences.
Karlis, Dimitris
and
Xekalaki, Evdokia
2007.
Mixed Poisson Distributions.
International Statistical Review,
Vol. 73,
Issue. 1,
p.
35.
Stoyanov, Jordan
and
Lin, Gwo Dong
2011.
Mixtures of power series distributions: identifiability via uniqueness in problems of moments.
Annals of the Institute of Statistical Mathematics,
Vol. 63,
Issue. 2,
p.
291.
Ghosal, Subhashis
and
Roy, Anindya
2011.
Identifiability of the proportion of null hypotheses in skew-mixture models for the p-value distribution.
Electronic Journal of Statistics,
Vol. 5,
Issue. none,
Everitt, B. S.
2014.
Wiley StatsRef: Statistics Reference Online.
Yang, Lei
and
Wu, Xianyi
2014.
A new sufficient condition for identifiability of countably infinite mixtures.
Metrika,
Vol. 77,
Issue. 3,
p.
377.
Kota, Pavan K.
LeJeune, Daniel
Drezek, Rebekah A.
and
Baraniuk, Richard G.
2022.
Extreme Compressed Sensing of Poisson Rates From Multiple Measurements.
IEEE Transactions on Signal Processing,
Vol. 70,
Issue. ,
p.
2388.
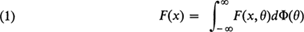 where Φ is a d.f., then it is of interest to determine conditions under which F(x) and F(x, θ) uniquely determine Φ. If there is only one Φ satisfying (1), F is said to be an identifiable mixture. Usually a consistency assumption is used whereby it is presumed that there exists at least one solution to (1).
where Φ is a d.f., then it is of interest to determine conditions under which F(x) and F(x, θ) uniquely determine Φ. If there is only one Φ satisfying (1), F is said to be an identifiable mixture. Usually a consistency assumption is used whereby it is presumed that there exists at least one solution to (1).

