Published online by Cambridge University Press: 14 July 2016
For each positive integer n, let Sn be the nth partial sum of a sequence of i.i.d. random variables which assume the values +1 and −1 with respective probabilities p and 1 – p, having mean μ= 2p − 1. The exact distribution of the random variable 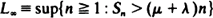 , where sup Ø= 0, is given for the case that λ > 0 and μ+ λ= k/(k + 2) for any non-negative integer k. Tables to the 99.99 percentile of some of these distributions, as well as a limiting distribution, are given for the special case of a symmetric simple random walk (p = 1/2).
, where sup Ø= 0, is given for the case that λ > 0 and μ+ λ= k/(k + 2) for any non-negative integer k. Tables to the 99.99 percentile of some of these distributions, as well as a limiting distribution, are given for the special case of a symmetric simple random walk (p = 1/2).
Present address: Department of Statistics, University of Kentucky, Lexington, KY 40506, USA.
This investigation was partially supported by a Research Development Fund Award from the State University of New York Research Foundation.