Article contents
Wilson spaces and stable splittings of BTr
Published online by Cambridge University Press: 18 May 2009
Extract
Let Q(X) denote 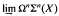 and let BTr denote the classifying space of the r-torus. In [8], Segal showed that Q(BT1) is homotopy equivalent to a product BU × F where BU denotes the classifying space for stable complex vector bundles and F a space with finite homotopy groups. This result has been a very useful one. For example, in [5] it was used to show that up to a stable homotopy equivalence there is only one loop structure on the 3-sphere at each odd prime p. (The subsequent work of Dwyer, Miller, and Wilkerson shows this result is even true unstably, at every prime p.) In [6] it was used to classify, up to homology, the stable self maps of the projective spaces ℂPn and ℍPn. In [5] I asked if a splitting similar to Segal's might exist for Q(BTr) when r≥2. In particular, since the homotopy and homology groups of BU are torsion free it seemed natural to ask if Q(BTr), when r>, could likewise contain a retract with torsion free homology and homotopy groups and whose complement is rationally trivial. The purpose of this note is to show that the answer is no.
and let BTr denote the classifying space of the r-torus. In [8], Segal showed that Q(BT1) is homotopy equivalent to a product BU × F where BU denotes the classifying space for stable complex vector bundles and F a space with finite homotopy groups. This result has been a very useful one. For example, in [5] it was used to show that up to a stable homotopy equivalence there is only one loop structure on the 3-sphere at each odd prime p. (The subsequent work of Dwyer, Miller, and Wilkerson shows this result is even true unstably, at every prime p.) In [6] it was used to classify, up to homology, the stable self maps of the projective spaces ℂPn and ℍPn. In [5] I asked if a splitting similar to Segal's might exist for Q(BTr) when r≥2. In particular, since the homotopy and homology groups of BU are torsion free it seemed natural to ask if Q(BTr), when r>, could likewise contain a retract with torsion free homology and homotopy groups and whose complement is rationally trivial. The purpose of this note is to show that the answer is no.
- Type
- Research Article
- Information
- Copyright
- Copyright © Glasgow Mathematical Journal Trust 1994
References
REFERENCES
- 1
- Cited by


