Hostname: page-component-586b7cd67f-2brh9 Total loading time: 0 Render date: 2024-11-24T18:41:39.375Z Has data issue: false hasContentIssue false
  • English
  • Français

SYMMETRY BREAKING FOR GROUND-STATE SOLUTIONS OF HÉNON SYSTEMS IN A BALL

Published online by Cambridge University Press:  08 December 2010

HAIYANG HE
HAIYANG HE*
Affiliation:
Department of Mathematics, Hunan Normal University, Changsha Hunan 410081, The People's Republic of China e-mail: [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

We consider in this paper the problem (1)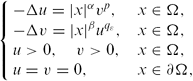 where Ω is the unit ball in ℝN centred at the origin, 0 ≤ α < pN,β > 0, N ≥ 3. Suppose qϵ → q as ϵ → 0+ and qϵ, q satisfy, respectively, 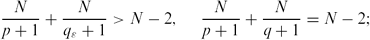we investigate the asymptotic estimates of the ground-state solutions (uϵ, vϵ) of (1) as β → + ∞ with p, qϵ fixed. We also show the symmetry-breaking phenomenon with α, β fixed and qϵ → q as ϵ → 0+. In addition, the ground-state solution is non-radial provided that ϵ > 0 is small or β is large enough.

Keywords

35J5035J60
Type
Research Article
Information
Glasgow Mathematical Journal , Volume 53 , Issue 2 , May 2011 , pp. 245 - 255
Copyright
Copyright © Glasgow Mathematical Journal Trust 2010

References

REFERENCES

1.Byeon, J. and Wang, Z-Q., On the Hénon equation: Asymptotic profile of ground state II, J. Differ. Equ. 216 (2005), 78–108.CrossRefGoogle Scholar
2.Byeon, J. and Wang, Z-Q., On the Hénon equation: Asymptotic profile of ground state I, Ann. Inst. H. Poincaré Anal. Non Linéaire 23 (2006), 803–828.CrossRefGoogle Scholar
3.Cao, D. and Peng, S., The asymptotic behavior of the ground state solutions for Hénon equation, J. Math. Anal. Appl. 278 (2003), 1–17.CrossRefGoogle Scholar
4.Chen, X., Li, C. and Ou, B., Classification of solution for a system of integral equations, Commun. Partial Differ. Equ. 30 (2005), 59–65.CrossRefGoogle Scholar
5.De Figueiredo, D. G. and Felmer, P. L., On superquadratic elliptic systems, Trans. Am. Math. Soc. 343 (1994), 99–106.CrossRefGoogle Scholar
6.de Figueiredo, D. G., Peral, I. and Rossi, J. D., The critical hyperbola for a hamiltonian elliptic system with weights, Ann. Mat. Pura Appl. 187 (2008), 531–545.CrossRefGoogle Scholar
7.Gidas, B., Ni, W. M. and Nirenberg, L., Symmetry of positive solutions of nonlinear elliptic equations in ℝN, in Mathematical analysis and applications: Part A, in Adv. in Math. Suppl. Stud., Vol. 7 (Academic Press, New York, 1981) pp. 369–402.Google Scholar
8.Guerra, I. A., Solutions of an elliptic system with a nearly critical exponent, Ann. Inst. H. Poincaré Anal. Non Linéaire 25 (2008), 181–200.CrossRefGoogle Scholar
9.He, H. and Yang, J., Asymptotic behavior of Solutions for Hénon systems with nearly critical exponent, J. Math. Anal. Appl. 347 (2008), 459–471.CrossRefGoogle Scholar
10.Hénon, M., Numerical experiments on the stability of spherical stellar systems, Astronom. Astrophys. Lib. 24 (1973), 229–238.Google Scholar
11.Hulshof, J. and van der Vorst, R. C. A. M., Differential systems with strongly indefinite variational struture, J. Funct. Anal. 114 (1993), 32–58.CrossRefGoogle Scholar
12.Hulshof, J. and van der Vorst, R. C. A. M., Asymptotic behavior of ground states, Proc. Am. Math. Soc. 124 (1996), 2423–2431.CrossRefGoogle Scholar
13.Liu, F. and Yang, J., Nontrivial solutions of Hardy-Hénon type elliptic systems, Acta Math. Sci. 27 (2007), 673–688.Google Scholar
14.Ni, W. M., A nonlinear Dirichlet problem on the unit ball and its applications, Indiana Univ. Math. J. 31 (1982), 801–807.CrossRefGoogle Scholar
15.Smets, D., Su, J. B. and Willem, M., Non-radial ground states for the Hénon equation, Comm. Contemp. Math. 4 (2002), 467–480.CrossRefGoogle Scholar
16.Smets, D. and Willem, M., Partial symmetry and asymptotic behavior for some elliptic variational problem, Calc. Var. Partial Differ. Equ. 18 (2005), 57–75.CrossRefGoogle Scholar