Finite volume schemes for a nonlinear hyperbolic equation. Convergence towards the entropy solution and error estimate

Abstract

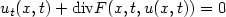In this paper, we study some finite volume schemes for the nonlinear hyperbolic equation ${u_t}(x,t)+\mbox{div}F(x,t,u(x,t))=0$ with the initial condition $u_{0}\in{L^\infty}(\mathbb{R}^N)$ 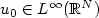. Passing to the limit in these schemes, we prove the existence of an entropy solution $u\in{L^infty}(\mathbb{R}^N\times\mathbb{R}_+)$ 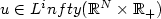. Proving also uniqueness, we obtain the convergence of the finite volume approximation to the entropy solution in $L^p_{loc}(\mathbb{R}^N\times\mathbb{R}_+)$ 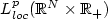, 1 ≤ p ≤ +∞. Furthermore, if ${u_0}\in {L^\infty}\cap\mbox{BV}_{loc}(\mathbb{R}^N)$ 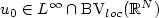, we show that $u\in\mbox{BV}_{loc}(\mathbb{R}^N\times\mathbb{R}_+)$ 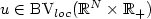, which leads to an “ $h^{\frac{1}{4}}$ ” error estimate between the approximate and the entropy solutions (where h defines the size of the mesh).