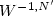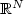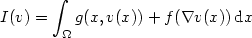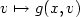Research Article
Variational approximation for detectingpoint-like target problems*
-
- Published online by Cambridge University Press:
- 06 August 2010, pp. 909-930
-
- Article
- Export citation
Solving the Cahn-Hilliard variational inequalitywith a semi-smooth Newton method
-
- Published online by Cambridge University Press:
- 18 August 2010, pp. 931-954
-
- Article
- Export citation
Analytical results on a model for damagingin domains and interfaces*
-
- Published online by Cambridge University Press:
- 18 August 2010, pp. 955-974
-
- Article
- Export citation
Optimality conditions for semilinear parabolic equations withcontrols in leading term*
-
- Published online by Cambridge University Press:
- 23 August 2010, pp. 975-994
-
- Article
- Export citation
Deterministic state-constrained optimal control problems without controllability assumptions
-
- Published online by Cambridge University Press:
- 06 August 2010, pp. 995-1015
-
- Article
- Export citation
Numerical solution of an inverse initial boundary value problem forthe wave equation in the presenceof conductivity imperfections ofsmall volume
-
- Published online by Cambridge University Press:
- 06 August 2010, pp. 1016-1034
-
- Article
- Export citation
Two-scale homogenization for a modelin strain gradient plasticity
-
- Published online by Cambridge University Press:
- 28 October 2010, pp. 1035-1065
-
- Article
- Export citation
Estimate of the pressure when its gradientis the divergence of a measure. Applications
-
- Published online by Cambridge University Press:
- 28 October 2010, pp. 1066-1087
-
- Article
- Export citation
On the null-controllability of diffusion equations
-
- Published online by Cambridge University Press:
- 23 August 2010, pp. 1088-1100
-
- Article
- Export citation
Exact controllability of a multilayer Rao-Nakra platewith clamped boundary conditions
-
- Published online by Cambridge University Press:
- 08 November 2010, pp. 1101-1132
-
- Article
- Export citation
A Haar-Rado type theorem for minimizersin Sobolev spaces
-
- Published online by Cambridge University Press:
- 28 October 2010, pp. 1133-1143
-
- Article
- Export citation
Strong stabilization of controlled vibrating systems
-
- Published online by Cambridge University Press:
- 08 November 2010, pp. 1144-1157
-
- Article
- Export citation
Scaling laws for non-Euclidean plates and theW2,2 isometric immersions of Riemannian metrics
-
- Published online by Cambridge University Press:
- 28 October 2010, pp. 1158-1173
-
- Article
- Export citation
Maximum principle for forward-backward doubly stochastic control systems and applications*
-
- Published online by Cambridge University Press:
- 08 November 2010, pp. 1174-1197
-
- Article
- Export citation
Approximate controllability by birth controlfor a nonlinear population dynamics model
-
- Published online by Cambridge University Press:
- 02 December 2010, pp. 1198-1213
-
- Article
- Export citation