Hostname: page-component-78c5997874-m6dg7 Total loading time: 0 Render date: 2024-11-16T05:19:26.545Z Has data issue: false hasContentIssue false
  • English
  • Français

A relaxation result for autonomous integral functionals with discontinuous non-coercive integrand

Published online by Cambridge University Press:  15 March 2004

Carlo Mariconda  and
Giulia Treu
Carlo Mariconda
Affiliation:
Dipartimento di Matematica pura e applicata, Università di Padova, 7 via Belzoni, 35131 Padova, Italy; [email protected].; [email protected].
Giulia Treu
Affiliation:
Dipartimento di Matematica pura e applicata, Università di Padova, 7 via Belzoni, 35131 Padova, Italy; [email protected].; [email protected].
Get access

Abstract

Let $L:\Bbb R^N\times\Bbb R^N\rightarrow\Bbb R$ be a Borelian function andconsider the following problems $$\inf\left\{F(y)=\int_a^bL(y(t),y'(t))\,{\rm d}t:\,y\in AC([a,b],\Bbb R^N), y(a)=A,\,y(b)=B\right\} \qquad\quad\! (P)$$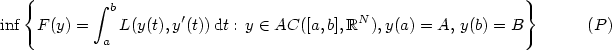 
 $$ \inf\left\{F^{**}(y)=\int_a^bL^{**}(y(t),y'(t))\,{\rm d}t:\,y\in AC([a,b],\Bbb R^N), y(a)=A,\,y(b)=B\right\}\cdot \quad\;\ \! (P^{**})$$ 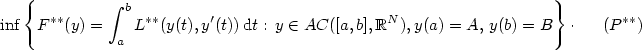We give a sufficient condition, weaker then superlinearity, under which $\inf F=\inf F^{**}$ 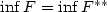if L is just continuous in x. We then extend a result of Cellina on the Lipschitz regularity of the minima of (P) when L is not superlinear.

Keywords

Lipschitzregularitynon-coercivediscontinuouscalculus of variations.
Type
Research Article
Information
ESAIM: Control, Optimisation and Calculus of Variations , Volume 10 , Issue 2 , April 2004 , pp. 201 - 210
Copyright
© EDP Sciences, SMAI, 2004

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

G. Alberti and F. Serra Cassano, Non-occurrence of gap for one-dimensional autonomous functionals. Ser. Adv. Math. Appl. Sci. Calculus of variations, homogenization and continuum mechanics 18 (1993) 1-17.
Amar, M., Bellettini, G. and Venturini, S., Integral representation of functionals defined on curves of W 1,p . Proc. R. Soc. Edinb. Sect. A 128 (1998) 193-217. CrossRef
Ambrosio, L., Ascenzi, O. and Buttazzo, G., Lipschitz regularity for minimizers of integral functionals with highly discontinuous integrands. J. Math. Anal. Appl. 142 (1989) 301-316. CrossRef
G. Buttazzo, Semicontinuity, relaxation and integral representation in the calculus of variations. Pitman Res. Notes Math. Ser. 207 (1989).
A. Cellina, The classical problem of the calculus of variations in the autonomous case: Relaxation and lipschitzianity of solutions. Preprint (2001).
G. Dal Maso and H. Frankowska, Autonomous Integral Functionals with Discontinuous Nonconvex Integrands: Lipschitz Regularity of Minimizers, DuBois-Reymond Necessary Conditions, and Hamilton-Jacobi Equations. Preprint (2002).
I. Ekeland and R. Témam, Convex analysis and variational problems. Classics Appl. Math. 28 (1999).
C. Mariconda and G. Treu, Lipschitz regularity of the minimizers of autonomous integral functionals with discontinuous non-convex integrands of slow growth. Dipartimento di Matematica pura e applicata, Università di Padova 10 (2003) preprint.
W. Rudin, Functional analysis. International Series in Pure and Applied Mathematics, McGraw-Hill, Inc., New York (1991).