A class of minimum principles for characterizingthe trajectories and the relaxation of dissipative systems

Abstract

This work is concerned with the reformulation of evolutionary problems in a weak form enabling consideration of solutions that may exhibit evolving microstructures. This reformulation is accomplished by expressing the evolutionary problem in variational form, i.e., by identifying a functional whose minimizers represent entire trajectories of the system. The particular class of functionals under consideration is derived by first defining a sequence of time-discretized minimum problems and subsequently formally passing to the limit of continuous time. The resulting functionals may be regarded as aweighted dissipation-energy functional with a weight decaying with a rate $1/\epsilon$ . 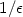The corresponding Euler-Lagrange equation leads to anelliptic regularization of the original evolutionary problem. The Γ-limit of these functionals for $\epsilon\to 0$ 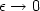is highlydegenerate and provides limited information regarding the limiting trajectories of the system. Instead we seek to characterize the minimizing trajectories directly. The special class of problems characterized by a rate-independent dissipation functional is amenable to a particularly illuminating analysis. For these systems it is possible to derive a priori bounds that are independent of the regularizing parameter, whence it is possible to extract convergent subsequences and find the limiting trajectories. Under general assumptions on the functionals, we show that all such limits satisfy the energetic formulation (S) & (E) for rate-independent systems. Moreover, we show that the accumulation points of the regularized solutions solve the associated limiting energetic formulation.