On the widths of the Arnol’d tongues

Abstract

Let 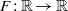$F: \mathbb{R} \rightarrow \mathbb{R} $ be a real analytic increasing diffeomorphism with 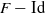$F- \mathrm{Id} $ being 1-periodic. Consider the translated family of maps 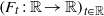$\mathop{({F}_{t} : \mathbb{R} \rightarrow \mathbb{R} )}\nolimits_{t\in \mathbb{R} } $ defined as ${F}_{t} (x)= F(x)+ t$. Let 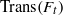$\mathrm{Trans} ({F}_{t} )$ be the translation number of 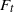${F}_{t} $ defined by

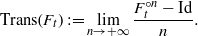$$\mathrm{Trans} ({F}_{t} ): = \lim _{n\rightarrow + \infty }\frac{{ F}_{t}^{\circ n} - \mathrm{Id} }{n} .$$

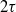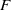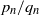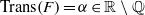Assume that there is a Herman ring of modulus $2\tau $ associated to $F$ and let ${p}_{n} / {q}_{n} $ be the $n$th convergent of $\mathrm{Trans} (F)= \alpha \in \mathbb{R} \setminus \mathbb{Q} $. Denoting by 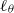${\ell }_{\theta } $ the length of the interval 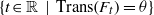$\{ t\in \mathbb{R} ~\mid ~\mathrm{Trans} ({F}_{t} )= \theta \} $, we prove that the sequence $({\ell }_{{p}_{n} / {q}_{n} } )$ decreases exponentially fast with respect to ${q}_{n} $. More precisely,

$$\mathop {\mathrm{lim\hphantom{,}sup} }\limits _{n\rightarrow + \infty } \frac{1}{{q}_{n} } \log {\ell }_{{p}_{n} / {q}_{n} } \leq - 2\pi \tau .$$

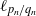There is a relation between ${\ell }_{{p}_{n} / {q}_{n} } $ and the width of the Arnol’d tongue, which confirms that the widths of the tongues decrease exponentially fast under suitable conditions.