Hostname: page-component-cd9895bd7-gvvz8 Total loading time: 0 Render date: 2024-12-25T05:55:48.137Z Has data issue: false hasContentIssue false
  • English
  • Français

A new proof of the non-tameness of the Nagata automorphism from the point of view of the Sarkisov program

Part of: Affine geometry Birational geometry

Published online by Cambridge University Press:  01 July 2008

Takashi Kishimoto
Takashi Kishimoto*
Affiliation:
Department of Mathematics, Faculty of Science, Saitama University, Saitama 338-8570, Japan (email: [email protected])
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

The Nagata automorphism is a kind of complicated automorphism on the affine 3-space 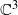. For a long time, it remained unknown whether or not the Nagata automorphism is tame until Shestakov and Umirbaev at last proved that it is not tame in 2004, by purely algebraic methods (e.g. Poisson algebra). In this paper, we consider a certain necessary condition for a given automorphism on 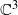to be tame from the point of view of the Sarkisov program established by Corti. Furthermore, by using it, we shall give a new algebro-geometric proof of the non-tameness of the Nagata automorphism.

Keywords

Nagata automorphismSarkisov programelementary links

MSC classification

Secondary: 14R10: Affine spaces (automorphisms, embeddings, exotic structures, cancellation problem) 14E07: Birational automorphisms, Cremona group and generalizations
Type
Research Article
Information
Compositio Mathematica , Volume 144 , Issue 4 , July 2008 , pp. 963 - 977
Copyright
Copyright © Foundation Compositio Mathematica 2008