Isometric actions on L_p-spaces: dependence on the value of p

Abstract

Answering a question by Chatterji–Druţu–Haglund, we prove that, for every locally compact group 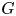$G$, there exists a critical constant 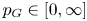$p_G \in [0,\infty ]$ such that 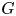$G$ admits a continuous affine isometric action on an 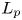$L_p$ space (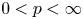$0< p<\infty$) with unbounded orbits if and only if 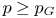$p \geq p_G$. A similar result holds for the existence of proper continuous affine isometric actions on 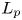$L_p$ spaces. Using a representation of cohomology by harmonic cocycles, we also show that such unbounded orbits cannot occur when the linear part comes from a measure-preserving action, or more generally a state-preserving action on a von Neumann algebra and 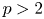$p>2$. We also prove the stability of this critical constant 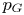$p_G$ under 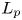$L_p$ measure equivalence, answering a question of Fisher.

1. Introduction

The study of affine isometric actions of groups on Banach spaces is an important theme in mathematics that is related to many other topics such as group cohomology, fixed point properties and geometric group theory. The case of actions on Hilbert spaces is very well-studied. For example, it is known that a second countable locally compact group $G$ has an affine isometric action on Hilbert spaces without fixed points (respectively, proper) if and only if $G$ does not have Kazhdan's property (T) (respectively, has the Haagerup property). The question of the behaviour of actions on other $L^p$ spaces (raised by Gromov in [Gro93, § $6.D_3$]) is less well-understood. There are interesting phenomena that can occur: while the groups $G=\mathrm {Sp}(n,1)$ have property (T), Pansu showed in [Pan89] that they admit affine isometric actions without fixed points on $L_p(G)$ for all $p > 4n+2$ (and actually proper actions by [DCTV08]). This was generalized by Bourdon and Pajot in [BP03]. In [Yu05], Yu proved that any hyperbolic group $\Gamma$ admits a proper affine isometric action on $\ell ^p(\Gamma \times \Gamma )$ for all $p$ large enough, see also [Nic13]. For more results and references, we refer to [BFGM07] where a systematic study of affine isometric actions of groups on $L_p$-spaces was undertaken. As suggested by the previous results, it is natural to expect that for a given group $G$, it should be ‘easier’ to act isometrically on an $L_p$-space when the value of $p$ gets larger. The following questions by Chatterji, Druţu and Haglund makes this expectation precise.

Question 1.1 [CDH10, Question 1.8]

Let $G$ be a group and $p>q \geq 2$. If every isometric action of $G$ on an $L^p$ space has a fixed point, is the same true for $L^q$ spaces? If $G$ admits a proper isometric action on an $L^q$ space, does it also admit a proper isometric action on an $L^p$ space?

The fact that these questions have a positive answer is sometimes referred to as Druţu's conjecture [Now15, LO21].

The main result of this paper confirms this intuition and, in particular, answers these questions. In this statement as in the whole paper, $L_p$ space means $L_p(X,\mu )$ for a standard measure space $(X,\mu )$.

Theorem 1 Let $G$ be a topological group. Take $0 < p \leq q < \infty$. Then for every continuous affine isometric action $\alpha : G \curvearrowright L_p$, there exists a continuous affine isometric action $\beta : G \curvearrowright L_q$ such that $\| \alpha _g(0) \|^p_{L_p}=\| \beta _g(0) \|^q_{L_q}$ for all $g \in G$.

Theorem 1 implies, in particular, that if a group $G$ has a continuous action by isometries on an $L_p$ space with unbounded (respectively, metrically proper) orbits, then it has such an action on an $L_q$ space.

Corollary 2 For every topological group $G$:

1. (i) the set of values of $p \in (0,\infty )$ such that $G$ admits a continuous action by isometries on an $L_p$ space with unbounded orbits is an interval of the form $(p_G,\infty )$ or $[p_G,\infty )$ for some $p_G \in \{0\} \cup [2,\infty ]$;

2. (ii) the set of values of $p \in (0,\infty )$ such that $G$ admits a proper continuous action by isometries on an $L_p$ space is an interval of the form $(p'_G,\infty )$ or $[p'_G,\infty )$ for some $p'_G \in \{0\} \cup [2,\infty ]$.

Recall that for $1 \leq p<\infty$, an action by isometries on an $L_p$ space has a fixed point if and only if it has bounded orbits ([BFGM07, Lemma 2.14] for $p \neq 1$, [BGM12] for $p=1$). Thus, Corollary 2 answers positively Question 1.1. A partial answer for $\ell _p$ spaces had already been obtained independently by Czuroń [Czu17] and Lavy and Olivier [LO21]. In [LO21] actions coming from ergodic probability measure-preserving actions were also covered. Unlike these previous results, it is worth mentioning that in Theorem 1, the linear part of the action $\beta$ that we construct is very different from the linear part of the original action $\alpha$. We refer to Theorem 4.3 for a more precise statement.

When $G$ is a second countable locally compact group, it is known that $p_G > 0$ if and only if $G$ has property (T) [BFGM07], in which case we must have $p_G \geq 2$ (in fact, by an argument of Fisher and Margulis from [FM05] that appears in [BFGM07], one can even show that $p_G > 2$ and that $G$ admits a continuous action by isometries on an $L_p$ space without fixed points for $p=p_G$, see also [LO21, DK18, Sal19]). Similarly, it is known that $p'_G > 0$ if and only if $G$ does not have the Haagerup property, in which case $p'_G \geq 2$ (see [Now06]). This last fact, as well as the fact that $p_G \notin (0,2)$, if often stated for second countable locally compact groups, but they are true for arbitrary topological groups, see Proposition 3.1 (but the fact that $p'_G \notin (0,2)$ is meaningful only for locally compact groups, as $p'_G=\infty$ trivially for nonlocally compact groups). The critical constant $p_G$ and $p'_G$ are different in general. For example, if $G$ is a locally compact group that has neither Kazhdan's property (T) nor Haagerup property, then $p_G=0$ and $p'_G \geq 2$. It is also known that, among Gromov-hyperbolic groups, the value of $p_G$ is unbounded, and explicit lower bounds have been obtained for random groups [DM19] (see also [LS21, Opp20]).

The linear part of the action $\beta$ constructed in Theorem 1 comes from an action on $(X,\mu )$ preserving an infinite measure. It is not possible to achieve the same with a finite measure. Indeed, when $G$ has property (T), any affine action on $L_p$ ($1\leq p<\infty$) whose linear part comes from a probability measure-preserving action has a fixed point. This is known when $G$ is discrete [LO21] or when $G$ admits a finite Kazhdan set [CK20]. In general, this is a particular case of the following result dealing with noncommutative $L_p$ spaces. Its proof relies on a general observation of independent interest: under a spectral gap and uniform convexity assumption, any cohomology class with values in an isometric representation has a unique harmonic representant (Lemma 5.1).

Theorem 3 Let $G$ be a locally compact property (T) group. An action $\alpha : G \curvearrowright L_p(M)$ by affine isometries on the noncommutative $L_p$ space of a von Neumann algebra $M$ has a fixed point in the following two cases:

• • $1< p\leq 2$ and $M=B(H)$ for a Hilbert space $H$;

• • $2 \leq p <\infty$ and the linear part of $\alpha$ comes from an action by automorphisms of $M$ preserving a faithful normal state.

We insist that the first conclusion of the theorem is not trivial. It is indeed an open question by Masato Mimura whether a locally compact property (T) group can have an unbounded action by isometries on a noncommutative $L_p$ space for $p<2$ (as explained previously this is not possible for usual $L_p$ spaces), and the previous result provides a negative answer for Schatten classes.

It is known that the metric space $(L_p,\|\cdot \|_p^{ {p}/{q}})$ isometrically embeds into $(L_q,\|\cdot \|_q)$ when $p \leq q$ (see [MN04, Remark 5.10]). This implies the following inequalities for the compression exponents [NP11] of a compactly generated group $G$

(1.1)\begin{equation} \forall 0< p < q<\infty, \quad p \alpha_p(G) \leq q \alpha_q(G). \end{equation}

As a consequence of Theorem 1, we obtain the same inequalities for the equivariant compression exponents:

(1.2)\begin{equation} \forall 0< p < q<\infty, \quad p \alpha_p^\#(G) \leq q \alpha_q^\#(G). \end{equation}

This inequality is often strict, see [NP11].

We also note that Theorem 1 can be applied to the whole isometry group of $L_p$ and this yields the following corollary.

Corollary 4 Take $0 < p \leq q < \infty$. Then $\mathord {\text { Isom}}(L_p)$ is isomorphic as a topological group to a closed subgroup of $\mathord {\text { Isom}}(L_q)$.

Note that if $p > 2$, the subgroup of translations $L_p \subset \mathord {\text { Isom}}(L_p)$ is not unitarily representable [Meg08, Theorem 3.1]. In particular, it cannot be embedded as a closed subgroup of $\mathord {\text { Isom}}(L_2)$, which is unitarily representable (by using the affine Gaussian functor [AIM21, Proposition 4.8] for example).

In view of Corollary 2, it is interesting to determine the parameters $p_G$ and $p'_G$, at least for some classes of groups. This motivates the study of their permanence properties, that we initiate in the last section of his paper. In particular, we prove the following result, which shows that they behave nicely with respect to $L_p$-measure equivalence (see Theorem 5 and the definitions preceding it). This answers a question by David Fisher (private communication).

Theorem 5 If two compactly generated locally compact groups $G_1$ and $G_2$ are $L_p$ measure equivalent, then the critical constants defined in Corollary 2 satisfy

\[ \min(p_{G_1},p) = \min(p_{G_2},p)\quad \textrm{and}\quad \min(p'_{G_1},p) = \min(p'_{G_2},p). \]

This paper is organized as follows. After some preliminaries in § 2, Theorem 1 and its Corollary 4 are proved in § 3 for $p=2$ and § 4 in the general case. Section 5 deals with harmonic cocycles and the proof of Theorem 3. In § 6, stability properties of the constants $p_G$ and $p'_G$ are investigated and in particular, Theorem 5 is proved.

2. Preliminaries

2.1 Nonsingular actions

Let $(X,\mu )$ be a $\sigma$-finite standard measure space (we always assume that our measure spaces are standard and we omit the $\sigma$-algebra). We denote by $[\mu ]$ the measure class of $\mu$. We denote by $\mathord {\text { Aut}}(X,[\mu ])$ the group of all nonsingular (preserving the measure class $[\mu ]$) automorphisms of $(X,\mu )$ up to equality almost everywhere. It is known that $\mathord {\text { Aut}}(X,[\mu ])$ is a Polish group for the topology of pointwise convergence on probability measures: a sequence $\theta _n \in \mathord {\text { Aut}}(X,[\mu ])$ converges to the identity if and only if $\lim _n \| (\theta _n)_* \nu - \nu \|_1=0$ for every probability measure $\nu \in [\mu ]$. We denote by $\mathord {\text { Aut}}(X,\mu )$ the group of all measure-preserving automorphisms of $(X,\mu )$ up to equality almost everywhere. It is a closed subgroup of $\mathord {\text { Aut}}(X,[\mu ])$. A continuous nonsingular action $\sigma : G \curvearrowright (X,\mu )$ of a topological group $G$ is a continuous homomorphism $\sigma : G \ni g \mapsto \sigma _g \in \mathord {\text { Aut}}(X,[\mu ])$.

2.2 Cohomology

Let $\pi : G \curvearrowright V$ be a continuous linear representation of a topological group $G$ on a topological vector space $V$. We denote by $Z^1(G,\pi,V)$ the set of all continuous $1$-cocycles, i.e. all continuous maps $c : G \rightarrow V$ such that $c(gh)=c(g)+g \cdot c(h)$ for all $g,h \in G$. We denote by $B^1(G,\pi,V) \subset Z^1(G,\pi,V)$ the set of all coboundaries, i.e. cocycles $c$ of the form $c(g)=g \cdot v -v$ for some $v \in V$.

Let $\sigma : G \curvearrowright (X,\mu )$ be a continuous nonsingular action of a topological group $G$. Let $A$ be an abelian topological group (here we use the additive notation for $A$ but this might change sometimes when $A=\mathbf {T}$). We denote by $Z^1_\sigma (G,A)$ the set of all $A$-valued $1$-cocycles of $\sigma$, i.e. all continuous functions $c : G \mapsto L_0(X,\mu,A)$ such that $c(gh)=c(g)+\sigma _g(c(h))$. Here for every $f \in L_0(X,\mu,A)$, we use the notation $\sigma _g(f)=f \circ \sigma _g^{-1}$. We denote by $B^1_\sigma (G,A)$ the set of all $1$-coboundaries, i.e. cocycles of the form $g \mapsto \sigma _g(f)-f$ for some $f \in L_0(X,\mu,A)$. Finally, we denote by $H^1_\sigma (G,A)=Z^1_\sigma (G,A)/B^1_\sigma (G,A)$ the cohomology group of $\sigma$.

2.3 Skew-product actions

Let $\sigma : G \curvearrowright (X,\mu )$ be a continuous nonsingular action of a topological group $G$. Suppose that $A$ is a locally compact abelian group and let $m$ be the Haar measure of $A$. Then for every $c \in Z^1_\sigma (G,A)$, we can define a new continuous nonsingular action $\sigma \rtimes c$ of $G$ on $(X \times A, \mu \otimes m)$ by the formula

\[ (\sigma \rtimes c)_g(x,a)=(gx,a+c(g^{-1})(x)). \]

The action $\sigma \rtimes c$ is called the skew-product action of $\sigma$ by $c$. Define a function $h : X \times A \rightarrow A$ by $h(x,a)=a$ for all $(x,a) \in X \times A$. Then, by construction, we have $c(g) \otimes 1=(\sigma \rtimes c)_g(h)-h$. Thus, the skew-product action $\sigma \rtimes c$ turns the cocycle $c$ into a coboundary.

2.4 The Maharam extension

Let $\sigma : G \curvearrowright (X,\mu )$ be a continuous nonsingular action of a topological group $G$. Then we can define the Radon–Nikodym cocycle $D \in Z^1_\sigma (G,\mathbf {R}^*_+)$ by the formula $D(g)= {{d} (\sigma _g)_* \mu }/{{d}\mu }$ for all $g \in G$. The skew-product action $\widetilde {\sigma }=\sigma \rtimes D^{-1} : G \curvearrowright X \times \mathbf {R}^*_+$ is called the Maharam extension of $\sigma$. Note that $\widetilde {\sigma }$ preserves the measure $\mu \otimes {d} \lambda$ where $d \lambda$ is the restriction to $\mathbf {R}^*_+$ of the Lebesgue measure of $\mathbf {R}$.

2.5 Isometric actions on $L_p$-spaces

Take $p > 0$ and let $(X,\mu )$ be a $\sigma$-finite measure space. For every $\theta : \mathord {\text { Aut}}(X,[\mu ])$, we define a linear isometry of $L_p(X,\mu )$ given by

\[ f \mapsto \bigg( \frac{\theta_* \mu}{\mu} \bigg)^{1/p} \theta(f). \]

The group $L_0(X,[\mu ],\mathbf {T})$ also acts by multiplication on $L_p(X,\mu )$. We thus obtain a continuous linear isometric representation

\[ \pi^{p,\mu} : \mathord{\text{ Aut}}(X,[\mu]) \ltimes L_0(X,[\mu],\mathbf{T}) \rightarrow \mathcal{O}(L_p(X,\mu)). \]

It follows from the Banach–Lamperti theorem that this map is surjective when $p \neq 2$. Note that if $\nu \in [\mu ]$, the canonical isometry $L_p(X,\mu ) \rightarrow L_p(X,\nu )$ given by $f \mapsto ( {\mu }/{\nu } )^{1/p}f$ is equivariant with respect to the natural actions $\pi ^{p,\mu }$ and $\pi ^{p,\nu }$ of $\mathord {\text { Aut}}(X,[\mu ]) \ltimes L_0(X,[\mu ],\mathbf {T})$.

Let $\sigma : G \rightarrow \mathord {\text { Aut}}(X,[\mu ])$ be a continuous nonsingular action of a topological group $G$. Then $\sigma ^{p,\mu } =\pi ^{p,\mu } \circ \sigma$ is a continuous linear isometric representation of $G$ on $L_p(X,\mu )$. Let $D \in Z^1_\sigma (G,R^*_+)$ be the Radon–Nikodym cocycle. Then for every $p > 0$ and every $g \in G$, the isometry $\sigma ^{p,\mu }(g)$ is given by the formula

\[ \sigma^{p,\mu}(g) : L_p(X,\mu) \ni f \mapsto D(g)^{1/p} \sigma_g(f) \in L_p(X,\mu). \]

Now take some cocycle $\omega \in Z^1_\sigma (G,\mathbf {T})$. Then the map $g \mapsto \omega (g) \sigma ^p_g$ is again a continuous linear isometric representation of $G$ on $L_p(X,\mu )$. Conversely, if $p \neq 2$, it follows from the Banach–Lamperti theorem that every continuous linear isometric representation of $G$ on $L_p(X,\mu )$ is of the form $\pi : g \mapsto \omega (g) \sigma ^{p,\mu }_g$ for some continuous nonsingular action $\sigma$ of $G$ and some cocycle $\omega \in Z^1_\sigma (G,\mathbf {T})$.

Let $\alpha$ be an affine isometric action of $G$ on some $L_p$-space. As the affine isometry group $\mathord {\text { Isom}}(L_p(X,\mu ))$ decomposes as a semi-direct product

\[ \mathord{\text { Isom}}(L_p(X,\mu))=\mathcal{O}(L_p(X,\mu)) \ltimes L_p(X,\mu), \]

where $L_p(X,\mu )$ acts by translations, we see that $\alpha$ is of the form $\alpha _g(f)=\pi (g)f+c(g)$ where $\pi$ is an isometric linear representation of $G$ on $L_p(X,\mu )$ and $c \in Z^1(G,\pi,L_p(X,\mu ))$ is a cocycle. Observe that even when $\pi =\sigma ^{p,\mu }$ for some nonsingular action $\sigma : G \curvearrowright (X,\mu )$, we do not have $Z^1(G,\sigma ^{p,\mu },L_p(X,\mu )) \subset Z^1_\sigma (G,\mathbf {C})$ unless $\sigma$ preserves the measure $\mu$.

3. The case $p=2$

Let $G$ be a topological group and let $p > 0$. We denote by $K^p(G)$ the set of all continuous functions $\psi : G \rightarrow \mathbf {R}_+$ of the form $\psi (g)=\| \alpha _g(0)\|_{L_p}^p$ for some continuous affine isometric action $\alpha$ of $G$ on some $L_p$-space. Note that $K^2(G)$ is the set of all continuous functions on $G$ that are conditionally of negative type.

By using the Gaussian functor, one has the following (classical) result.

Proposition 3.1 Let $G$ be a topological group. Take $\psi \in K^2(G)$ and $p > 0$. Then there exists a continuous probability measure-preserving action $\sigma : G \curvearrowright (X,\mu )$ and a cocycle $c \in Z^1_\sigma (G,\mathbf {R})$ such that $\psi (g)^{ {p}/{2}}=\| c(g) \|_{L_p}^p$ for all $g \in G$. In particular, $\psi ^{ {p}/{2}} \in K^p(G)$ for all $p > 0$.

Proof. By definition, there exists an orthogonal representation $\pi : G \rightarrow \mathcal {O}(H)$ on some Hilbert space $H$ and a cocycle $c \in Z^1(G,\pi, H)$ such that $\psi (g)=\|c(g)\|^2$ for all $g \in G$. Let $\sigma _\pi : G \curvearrowright (X,\mu )$ be the Gaussian action associated to $\sigma$; see, for example, the construction in [CCJ⁺01, Proof of Theorem 2.2.2]. This means that there exists a linear map $\xi \mapsto \widehat {\xi } \in L_0(X,\mu,\mathbf {R})$ such that $\widehat {\xi }$ is a centered Gaussian random variable of variance $\| \xi \|^2$ for all $\xi \in H$, and that $\sigma _{\pi }(\widehat {\xi })=\widehat {\pi (g) \xi }$ for all $\xi \in H$. Let $\widehat {c}(g)=\widehat {c(g)} \in L_p(X,\mu )$ for all $g \in G$. Then $\widehat {c}$ is a cocycle for $\sigma _{\pi }$ and a computation shows that $\| \widehat {c}(g) \|^p_{L_p}=C_p \| c(g)\|^p$ for all $g \in G$ and some constant $C_p > 0$.

Corollary 3.2 For every topological group $G$, we have $K^2(G) \subset K^p(G)$ for all $p \geq 2$.

Proof. The function $x \mapsto x^\alpha$ is a Bernstein function for all $0 < \alpha \leq 1$. It follows that for every $\psi \in K^2(G)$, we have $\psi ^{\alpha } \in K^2(G)$, hence $\psi ^{\alpha ({p}/{2})} \in K^p(G)$. The conclusion follows by taking $\alpha = {2}/{p}$.

4. Proof of the main theorem

Proposition 4.1 Let $G$ be a topological group. For every $p > 0$ and every $\psi \in K^p(G)$, there exists a continuous nonsingular action $\sigma : G \curvearrowright (X,\mu )$ and a cocycle $c \in Z^1(G, \sigma ^{p,\mu },L_p(X,\mu ))$ such that $\psi (g)=\| c(g)\|_{L_p}^p$ for all $g \in G$.

Proof. We may assume that $p \neq 2$ thanks to Proposition 3.1. By definition, there exists an affine isometric action $\alpha : G \curvearrowright L_p(X,\mu )$ for some probability space $(X,\mu )$ such that $\psi (g)=\| \alpha _g(0)\|_{L_p}^p$ for all $g \in G$. Write $\alpha _g(f)=\pi _g(f)+c(g)$ where $\pi$ is an isometric linear representation of $G$ on $L_p(X,\mu )$ and $c \in Z^1(G,\pi,L_p(X,\mu ))$. As $p \neq 2$, we can write $\pi (g)=\omega (g) \sigma ^{p,\mu }_g$ where $\sigma : G \curvearrowright (X,\mu )$ is some nonsingular action and $\omega \in Z^1_\sigma (G,\mathbf {T})$. Consider the skew-product nonsingular action $\tilde {\sigma }=\sigma \rtimes \omega : G \curvearrowright (X \times \mathbf {T}, \mu \otimes m)$ where $m$ is the Haar measure of $\mathbf {T}$. Observe that $\tilde {\sigma }_g(u)u^*=\omega (g) \otimes 1$ where $u$ is the function on $X \times \mathbf {T}$ given by $u(x,z)=z$ for all $(x,z) \in X \times \mathbf {T}$. It follows that $\tilde {c}: g \mapsto u c(g)$ defines an element $\tilde {c} \in Z^1(G,\tilde {\sigma }^p, L_p(X \times \mathbf {T},\mu \otimes m))$ such that $\| \tilde {c}(g)\|_p=\| c(g)\|_p$ for all $g \in G$, where $m$ is the Haar measure of $\mathbf {T}$.

Lemma 4.2 Take $0 < p < q < \infty$. Let $\varphi : \mathbf {C} \rightarrow \mathbf {R}$ be a nonzero, radial, compactly supported, Lipschitz function. Then there exists a constant $C(q) > 0$ such that for all $w \in \mathbf {C}$, we have

(4.1)\begin{equation} \int_{\mathbf{C}} \int_0^\infty |\varphi(z+\lambda^{-1/p}w) - \varphi(z)|^q \,{d}\lambda \,{d} z = C(q) |w|^p. \end{equation}

Proof. Let $S$ be the Lebesgue measure of the support of $\varphi$, $M=\| \varphi \|_\infty$ and let $K$ be the Lipschitz constant of $\varphi$. Then we have $|\varphi (z+\lambda ^{-1/p}) - \varphi (z)| \leq \min ( 2M,K \lambda ^{-1/p})$ for all $z \in \mathbf {C}$ and all $\lambda \in \mathbf {R}^*_+$. Therefore, we have

\[ \int_{\mathbf{C}} |\varphi(z+\lambda^{-1/p}) - \varphi(z)|^q \,{d} z \leq 2S \min( (2M)^q , K^q \lambda^{-q/p}). \]

As $q > p$, the function $\lambda \mapsto \min ( (2M)^q, K^q \lambda ^{-q/p})$ is integrable on $\mathbf {R}^*_+$. Therefore, we can define

\[ C(q)=\int_{\mathbf{C}} \int_0^\infty |\varphi(z+\lambda^{-1/p}) - \varphi(z)|^q \,{d}\lambda \,{d} z < +\infty. \]

As $\varphi$ is not constant, we have $C(q) > 0$. Finally, the statement for all $w \in \mathbf {C}$ follows from the change of variable $\lambda \mapsto |w|^p\lambda$ and the fact that $\varphi$ is radial.

Theorem 4.3 Let $G$ be a topological group. Take $0 < p < q < \infty$. Then for every $\psi \in K^p(G)$, there exists a continuous measure-preserving action $\sigma : G \curvearrowright (Y,\nu )$ and a function $h \in L_\infty (Y,\nu )$ such that $b(g)=\sigma _g(h)-h \in L_q(Y,\nu )$ with $\psi (g)=\| b(g)\|_{L_q}^q$ for all $g \in G$. In particular, $\psi \in K^q(G)$.

Proof. By Proposition 4.1, there exists a nonsingular action $\sigma : G \curvearrowright (X,\mu )$ and a cocycle $c \in Z^1(G, \sigma ^{p,\mu },L_p(X,\mu ))$ such that $\psi (g)=\| c(g)\|_{L_p}^p$ for all $g \in G$. Let $\widetilde {\sigma } : G {\curvearrowright } (\widetilde {X},\widetilde {\mu })$ be the Maharam extension of $\sigma$. This means that $(\widetilde {X},\widetilde {\mu }) = (X \times \mathbf {R}^*_+,\mu \otimes d \lambda )$ where $d \lambda$ is the restriction to $\mathbf {R}^*_+$ of the Lebesgue measure of $\mathbf {R}$ and $\widetilde {\sigma } : G {\curvearrowright } (\widetilde {X},\widetilde {\mu })$ is the measure-preserving action given by $g(x,\lambda ) = (gx,( ({d g^{-1} \mu }/{d\mu })(x))^{-1} \lambda )$. Define $\widetilde c \in Z^1_{\widetilde {\sigma }}(G,\mathbf {C})$ by the formula $\widetilde {c}(g,x,\lambda ) = \lambda ^{- {1}/{p}} c(g,x)$ (observe that $\widetilde {c}$ indeed satisfies the cocycle relation thanks to the term $\lambda ^{-1/p}$). Let $\rho : G {\curvearrowright } (Y,\nu )$ be the skew-product action of $\widetilde {\sigma }$ by $\widetilde c$. This is the measure space $(Y,\nu )=(\widetilde X \times \mathbf {C}, \widetilde {\mu } \otimes {d}z )$ and $\rho$ is the measure-preserving action given by $g(\widetilde x,z) = (g \widetilde x,z+\widetilde c(g^{-1},\widetilde x))$. Let $\varphi \colon \mathbf {C} \to \mathbf {R}$ be a nonzero, radial, compactly supported, Lipschitz function. Define a function $h \in L_\infty (Y,\nu )$ by $h(\widetilde x,z)=\varphi (z)$, and let $b(g) = \rho _g(h) - h$ for all $g \in G$. Then Lemma 4.2 shows that $b$ is a cocycle with values in $L_q(Y,\nu )$ that satisfies the conclusion of the theorem up to a constant $C(q) > 0$.

Remark 4.4 The idea of the proof of Theorem 4.3 and of the cocycle $\widetilde {c}$ becomes very natural if one uses the notion of modular bundle and Haagerup's canonical $L_p$-spaces as explained in [AIM21, §§ A.2 and A.3]. Indeed, by viewing the canonical $L_p$-space $L_p(X)$ as a subspace of $L_0(\mathrm {Mod}(X))$, the isometric linear representation $\sigma ^p : G \curvearrowright L_p(X)$ associated to some nonsingular action $\sigma : G \curvearrowright X$ is identified with the restriction to $L_p(X)$ of the Maharam extension $\mathrm {Mod}(\sigma ) : G \curvearrowright \mathrm {Mod}(X)$. We can therefore identify every cocycle $c \in Z^1(G,\sigma ^p,L_p(X))$ with a cocycle $\widetilde {c} \in Z^1_{\mathrm {Mod}(\sigma )}(G,\mathbf {C})$. This is crucial in order to be able to use the skew-product construction.

Proof of Corollary 4 Let $G=\mathord {\text { Isom}}(L_p)$ with its canonical affine isometric action on $L_p$. By applying Theorem 1, we obtain a continuous homomorphism $\Psi : \mathord {\text { Isom}}(L_p) \rightarrow \mathord {\text { Isom}}(L_q)$ such that

\[ \| g(0) \|_{L_p}^p=\| \Psi(g)(0) \|_{L_q}^q \quad \text{ for all } g \in \mathord{\text { Isom}}(L_p). \]

We have to show that $\Psi$ is a homeomorphism on its range. Take $(g_n)_{n \in \mathbf {N}}$ a sequence in $\mathord {\text { Isom}}(L_p)$ and suppose that $\Psi (g_n) \to \text {id}$. We have to show that $g_n \to \text {id}$. Take $f \in L_p$ and let $\tau _f \in \mathord {\text { Isom}}(L_p)$ be the translation by $f$. Then

\[ \| g_n(f)-f\|^p_{L_p}=\| (\tau_f^{-1} \circ g_n \circ \tau_f)(0)\|^p_{L_p} = \| \Psi(\tau_f^{-1} \circ g_n \circ \tau_f)(0)\|^q_{L_q}. \]

As $\Psi (g_n) \to \text {id}$, we also have $\Psi (\tau _f^{-1} \circ g_n \circ \tau _f)= \Psi (\tau _f)^{-1} \circ \Psi (g_n) \circ \Psi (\tau _f) \to \text {id}$. This yields

\[ \lim_n \| g_n(f)-f\|^p_{L_p}=\lim_n \| \Psi(\tau_f^{-1} \circ g_n \circ \tau_f)(0)\|^q_{L_q} =0. \]

As this holds for all $f \in L_p$, we conclude that $g_n \to \text {id}$ as we wanted.

Question 4.5 Is Theorem 4.3 still true when $q=p$? Can we at least realize $\psi$ with an affine isometric action whose linear part comes from a measure-preserving action of $G$? One can show that if a measurable function $\varphi : \mathbf {R} \rightarrow \mathbf {R}$ satisfies

\[ \int_\mathbf{R} \int_0^\infty |\varphi(x+\lambda^{-1/p})-\varphi(x)|^p \,{d} \lambda \,{d} x < +\infty \]

for some $p \geq 1$, then $\varphi$ is almost surely constant. Therefore, our method for the proof of Theorem 4.3 cannot work when $q=p$.

Question 4.6 Take $0 < p,q < \infty$. Is it true that $\mathord {\text { Isom}}(L_p)$ embeds as a closed subgroup of $\mathord {\text { Isom}}(L_q)$ if and only if $p \leq \max (2,q)$?

5. Harmonic cocycles and state-preserving actions

Let $E$ be a uniformly convex Banach space and let $\pi$ be a continuous representation of a locally compact group $G$ on $E$. Then we can decompose $E$ as a $\pi$-invariant direct sum $E=E_\pi \oplus E^\pi$ where $E^\pi$ is the subspace of $\pi$-invariant vectors and $E_\pi$ is its natural complement (defined as the orthogonal of $(E^*)^{\pi ^*}$ where $\pi ^*$ is the dual representation of $\pi$ on $E^*$), see [BFGM07]. We say that $\pi$ has spectral gap if $\pi |_{E_\pi }$ has no almost invariant vectors. By [DN19, Theorem 1.1], this is equivalent to the existence of a symmetric compactly supported probability measure $\mu$ on $G$ such that $\|\pi (\mu )|_{E_\pi }\| < 1$.

Lemma 5.1 Let $G$ be a locally compact group and let $\pi$ be a representation of $G$ on a uniformly convex Banach space $E$ that has spectral gap. Let $\mu$ be a symmetric compactly supported probability measure $\mu$ on $G$ such that $\| \pi (\mu )|_{E_\pi } \| < 1$. Then every cohomology class in $H^1(G,\pi,E)$ admits a unique $\mu$-harmonic representant.

Proof. By decomposing $E=E_\pi \oplus E^\pi$, we can reduce the problem to the case where $\pi$ is either trivial or has no invariant vectors.

Assume first that $\pi$ is trivial. Then $B^1(G,\pi,E)=0$ and an element of $Z^1(G,\pi,E)$ is just a group homomorphism from $G$ to $E$. Thus, because $\mu$ is symmetric, every element of $Z^1(G,\pi,E)$ is $\mu$-harmonic.

Now, assume that $\pi$ has no invariant vectors, i.e. $E=E_\pi$. Take $c \in Z^1(G,\pi,E)$. Let $\alpha$ be the affine isometric action of $G$ on $E$ associated to $c$. Then the affine map $\alpha (\mu )=\int _{g \in G} \alpha _g \,{d} \mu (g)$ is $k$-Lipschitz with $k=\|\pi (\mu )\| <1$. Therefore, $\alpha (\mu )$ has a fixed point $\xi \in E$ for some $\xi \in E$. Let $c'(g)=c(g)+\pi (g)\xi -\xi$. Then we get $\int _G c'(g) \,{d} \mu (g) =\alpha (\mu ) \xi -\xi =0$. We conclude that $c'$ is a $\mu$-harmonic representant of the cohomology class of $c$. For the uniqueness part, observe that if we have a coboundary $b(g)=\pi (g)\xi -\xi$ that is $\mu$-harmonic, then $\pi (\mu )\xi =\xi$, hence $\xi =0$ because $\| \pi (\mu ) \| < 1$.

Lemma 5.2 Let $G$ be a compactly generated locally compact group and let $\pi _1,\pi _2$ be two representations of $G$ on two strictly convex Banach spaces $E_1$ and $E_2$. Suppose moreover that $E_1$ is uniformly convex and that $\pi _1$ has spectral gap. Then every injective continuous $G$-equivariant linear map $\psi : E_1 \rightarrow E_2$ induces an injective map $\psi _* : H^1(G,\pi _1,E_1) \rightarrow H^1(G,\pi _2,E_2)$.

Proof. As $\pi _1$ has spectral gap and $E_1$ is uniformly convex, we can find a symmetric compactly supported probability measure $\mu$ on $G$ such that $\| \pi _1(\mu )_{E_{\pi _1}} \| < 1$. As $G$ is compactly generated, we can assume that the support of $\mu$ generates $G$.

Take $\omega \in H^1(G,\pi _1,E_1)$. By Lemma 5.1 $\omega$ admits a $\mu$-harmonic representant $c \in Z^1(G,\pi _1,E_1)$. Then $\psi _*\omega \in H^1(G,\pi _1,E_1)$ is represented by the cocycle $c' : g \mapsto \psi (c(g))$. Note that $c'$ is still $\mu$-harmonic. Suppose that $\psi _*\omega =0$. This means that $c'$ is a coboundary, i.e. $c'(g)=\pi _2(g) \xi -\xi$ for some $\xi \in E_2$ and all $g \in G$. As $c'$ is $\mu$-harmonic, we have $\int _G \pi _2(g)\xi \,{d} \mu (g) = \xi$. As $E_2$ is strictly convex, this implies that $\pi _2(g)\xi =\xi$ for all $g$ in the support of $\mu$, hence for all $g \in G$. We conclude that $c'=0$, hence $c=0$ because $\psi$ is injective.

Remark 5.3 The previous lemma applies, for example, when $\sigma : G \curvearrowright (X,\mu )$ is a continuous probability measure-preserving action of a compactly generated locally compact group, $(\pi _i,E_i) =(\sigma ^{p_i,\mu }, L^{p_i}(X,\mu ))$ for $\infty >p_1 \geq p_2>1$ and $\psi$ is the inclusion map $L^{p_1} \to L^{p_2}$. The lemma shows that

\[ \forall 1< p_1< p_2<\infty,\quad H^1(G,\sigma^{p_2,\mu})=0 \implies H^1(G,\sigma^{p_1,\mu})=0. \]

Indeed, the assumption that $H^1(G,\sigma ^{p_2,\mu }) =0$ implies that $\sigma ^{p_2,\mu }$ has spectral gap, which implies that $\sigma ^{p_1,\mu }$ has spectral gap, see [BFGM07]. In particular, if $G$ is a locally compact property (T) group, $H^1(G,\sigma ^{p,\mu })=0$ for every $p\in [1,\infty )$. This was already known when $G$ is discrete [LO21], or $G$ admits a finite Kazhdan set [CK20].

More generally, the lemma applies to the actions on noncommutative $L_p$ spaces associated to state-preserving actions on von Neumann algebras, and also for actions on Schatten $p$-classes. We start with the latter as it is more elementary.

For a Hilbert space $H$, we denote by $S_p(H)$ the Schatten $p$-class, that is, the space of operators on $H$ such that $\|T\|_p:= (\mathrm {Tr}(|T|^p))^{ {1}/{p}}<\infty$. We say that a group has $FS_p$ if for every $H$ and every continuous isometric representation $\pi : G \curvearrowright S_p(H))$, $H^1(G,\pi,S_p(H))=0$.

Theorem 5.4 If $G$ is a $\sigma$-compact locally compact group, then the set of $1< p<\infty$ such that $G$ has $FS_p$ is empty if $G$ does not have property (T), and is an interval containing $(1,2]$ otherwise.

Proof. Assume that $G$ does not have property (T). By Guichardet's theorem, there is a unitary representation $\pi$ on a Hilbert space $H$ and an unbounded cocycle $b \in Z^1(G,\pi )$. If $\xi \in H^*$ is a unit vector, then the formula $g \cdot T = \pi (g) T + b(g) \otimes \xi$ defines an unbounded action by isometries on $S_p(H)$ for every $1 \leq p \leq \infty$. Thus, $G$ does not have $FS_p$.

If $G$ has property (T), then $G$ is compactly generated, and has $FS_2$ by Delorme's theorem ($S_2(H)$ is a Hilbert space). We have to prove that, if $1< p< q<\infty$ are such that $G$ has $FS_q$, then $G$ has $FS_p$. We can assume that $p \neq 2$. Let $\pi \colon G \to O(S_p(H))$ be an orthogonal representation. By [Yea81], $\pi _g$ is of the form $\pi _g(T) = W_g J_g(T)$ for a unitary $W_g$ and a Jordan automorphism $J_g$ of $B(H)$. In particular, the same formula gives rise to an isometric representation on $S_q(H)$ and the inclusion $S_p(H) \subset S_q(G)$ is equivariant. Moreover, both representations have spectral gap [Yea81], and the Schatten spaces are uniformly convex when $1< p<\infty$. We conclude by Lemma 5.2 that $H^1(G,S_p(H)) \to H^1(G,S_q(H))=0$ is injective and $H^1(G,S_p(H))=0$.

To state our result about state-preserving actions, we need to recall Haagerup's general definition of noncommutative $L_p$ spaces [Haa79]. We follow the approach in [Mar19, § 2.1]. If $M$ is a von Neumann algebra, the core of $M$ is the unique (up to unique isomorphism) tuple $(c(M),\tau,\theta,\iota )$ of a von Neumann algebra $c(M)$, a normal faithful semifinite trace $\tau$ on $c(M)$, a continuous homomorphism $\theta :\mathbf {R} \to \mathrm {Aut}(M)$, and a normal injective $*$-homomorphism $\iota : M \to c(M)$ satisfying $\tau \circ \theta _s = e^{-s}\tau$ and $\{x \in c(M) \mid \forall s \in \mathbf {R}, \theta _s (x)=x\} = \iota (M)$. In the following, we identify $M$ with its image in $c(M)$ and write $x$ instead of $\iota (x)$. For example, if $\phi$ is a normal faithful weight on $M$, the core can be realized as $c(M) = M \rtimes _{\sigma ^\phi } \mathbf {R}$, the crossed product by the modular automorphism group of $\phi$. In that case, $\theta$ is given by the dual action and $\iota$ the natural inclusion. For details on the construction and the existence of a trace $\tau$ as required, see [Tak03]. Then $L_p(M)$ is defined as the space of $\tau$-measurable operators affiliated to $c(M)$ such that $\theta _s(h)= e^{-s/p} h$ for all $s \in \mathbf {R}$. By [Haa79, Theorems 1.2 and 1.3], for every normal state $\varphi$ on $M$, there is a unique element of $L_1(M)$, that we also denote $\varphi$, satisfying

\[ \varphi \bigg(\int_{\mathbf{R}} \theta_t(x) \,\textit{dt}\bigg) = \tau(\varphi x) \]

for every nonnegative $x \in c(M)$. Moreover, this map extends by linearity to an isomorphism $M_* \to L_1(M)$. This allows us to define, for $1\leq p<\infty$ and $h \in L_p(M)$, $\|h\|_p := \| |h|^p \|_{M_*}^{ {1}/{p}}$. This turns $L_p(M)$ into a Banach space that is uniformly convex if $1< p<\infty$, and the $\|\cdot \|_p$ norms satisfy Hölder's inequality.

By the uniqueness of the core, any continuous action by automorphisms of $G$ on $M$ gives rise to a continuous action by isometries on $L_p(M)$, that we call the $p$-Koopman representation.

Theorem 5.5 Let $G$ be a locally compact group with property (T). Let $\sigma : G \rightarrow \mathord {\text { Aut}}(M)$ be an action on a von Neumann algebra $M$ that preserves some faithful normal state $\varphi$ on $M$. Take $p \geq 2$ and let $\pi ^p : G \rightarrow O(L_p(M))$ be the $p$-Koopman representation associated to $\sigma$. Then $H^1(G,\pi ^p,L_p(M))=0$.

Proof. First, $\pi _p$ has spectral gap by [Oli12].

Define a continuous linear map $\psi : L_p(M) \rightarrow L_2(M)$ by the formula

\[ \psi(x\varphi^{1/p}) = x\varphi^{1/2} \]

for all $x \in M$. This map is well-defined because $M\varphi ^{1/p}$ is dense in $L_p(M)$ and for all $x \in M$, we have

\[ \| x \varphi^{1/2}\|_2=\| x \varphi^{1/p} \cdot \varphi^{1/q}\|_2 \leq \| x \varphi^{1/p}\|_p \cdot \| \varphi^{1/q}\|_q= \| x \varphi^{1/p}\|_p, \]

where $ {1}/{p}+ {1}/{q}=\frac {1}{2}$. Observe that $\pi ^p(g)(x\varphi ^{1/p})=\sigma (g)(x)\varphi ^{1/p}$ for all $x \in M$, because $\sigma$ preserves $\varphi$, and we have the same formula for $p=2$. This implies that $\psi$ is $G$-equivariant with respect to the Koopman representations of $\sigma$. Thus, it induces an injective map from $H^1(G,\pi ^p,L_p(M))$ into $H^1(G,\pi ^2,L_2(M))$ by Lemma 5.2. As $G$ has property (T), we know that $H^1(G,\pi ^2,L_2(M))=0$. We conclude that $H^1(G,\pi ^p,L_p(M))=0$.

6. Stability properties of the constants $p_G$ and $p'_G$

We start with some elementary stability properties.

Proposition 6.1 Let $G$ be a locally compact group and let $H< G$ be a closed subgroup. Then $p'_H \leq p'_G$. If $H \triangleleft G$ is a closed normal subgroup, then $p_{G/H} \geq p_G$.

Proof. Any proper action of $G$ on an $L_p$ space restricts to a proper action of $H$, so $p'_H \leq p'_G$. If $H$ is normal, any action of $G/H$ with unbounded orbits on an $L_p$ space can be seen as an action of $G$, so $p_{G/H} \geq p_G$.

Proposition 6.2 Let $G_1,G_2$ be two locally compact groups. Then

\[ p_{G_1 \times G_2} =\min(p_{G_1},p_{G_2}) \quad \text{and} \quad p'_{G_1 \times G_2} =\max(p'_{G_1},p'_{G_2}). \]

Proof. The inequalities $p_{G_1 \times G_2} \leq \min (p_{G_1},p_{G_2})$ and $p'_{G_1 \times G_2} \geq \max (p'_{G_1},p'_{G_2})$ follow from Proposition 6.1.

For the inequality $p_{G_1 \times G_2} \geq \min (p_{G_1},p_{G_2})$, observe that if $G_1 \times G_2 \curvearrowright L_p$ has unbounded orbits, then its restriction to either $G_1$ or $G_2$ also has unbounded orbits.

For the inequality $p'_{G_1 \times G_2} \leq \max (p'_{G_1},p'_{G_2})$, observe that given two isometric actions $G_1 \curvearrowright L_p(\Omega _1)$ and $G_1 \curvearrowright L_p(\Omega _2)$, one can construct the action of $G_1 \times G_2$ on $L_p(\Omega _1 \cup \Omega _2)$, which is proper whenever both actions were proper.

Proposition 6.3 Let $G$ be a locally compact group and let $K \triangleleft G$ be a normal compact subgroup. Then

\[ p_{G/K} = p_G \quad \text{and} \quad p'_{G/K} = p'_G. \]

Proof. The only thing to note is that the space of $K$-invariant vectors for an isometric representation of $G$ on an $L_p$-space is isometric to an $L_p$-space. This either follows from the general form of isometries of $L_p$-space, or from the classical result [Tza69] that the range of a norm $1$ projection in $B(L_p)$ is isometric to an $L_p$-space.

We now investigate the stability of the constants $p_G$ and $p'_G$ under measure equivalence. For this we need to recall some definitions.

Let $G$ be a locally compact group $G$ with left Haar measure $m_G$. A measure-preserving action of $G$ on $(X,\mu )$ is called principal if there is a measure-preserving conjugacy between $G \curvearrowright (X,\mu )$ and an action of the form $G \curvearrowright (G \times \Omega, m_G \otimes \nu )$ where $G$ acts by translation of the left coordinate and $(\Omega, \nu )$ is a measure space with finite measure. In that case, we can identify $\Omega$ with $X/G$ and, thus, equip $X/G$ with the finite measure space structure coming from this identification. The finite measure on $X/G$ coming from this identification is denoted $\mu /G$.

Let $G_1$ and $G_2$ be two locally compact groups. The groups $G_1,G_2$ are said to be measure equivalent if there is a measure equivalence coupling, that is a measure space $(X,\mu )$ equipped with two commuting measure-preserving principal actions of $G_1$ and $G_2$. Observe that we then obtain a measure-preserving action of $G_2$ on $(X/G_1,\mu /{G_1})$ and of $G_1$ on $(X/G_2,\mu /{G_2})$. We use the notation $\mu _1=\mu /G_1$ and $\mu _2=\mu /G_2$.

Let $p_1 : X \rightarrow G_1$ be a $G_1$-equivariant map (it always exists because the action of $G_1$ is principal). Then for every $g_2 \in G$, the map

\[ X \ni \omega \mapsto p_1(g_2 \omega)^{-1}p_1(\omega) \in G_1 \]

is $G_1$-invariant. Thus, one can define a cocycle $c_1 : G_2 \times X/G_1 \rightarrow G_1$ by the formula

\[ c_1(g_2,\omega_1)=p_1(g_2 \omega)^{-1}p_1(\omega), \]

where $\omega \in X$ is any representant of $\omega _1 \in X/G_1$. Observe that the cocycle $c_1$ depends on the section $p_1$.

Now, suppose that $G_1$ is compactly generated. Take $p > 0$. We say that the measure equivalence coupling $(X,\mu )$ is $L_p$-integrable over $G_1$ if there exists a $G_1$-equivariant map $p_1 : X \rightarrow G_1$ such that the associated cocycle $c_1$ defined previously satisfies the following $L_p$-integrability condition:

\[ \int_{X/G_1} |c_1(g_2,\omega_1)|_{G_1}^p \,{d} \mu_1(\omega_1)<\infty\ \forall g_2 \in G_2, \]

where $| \cdot |_{G_1}$ is the word length with respect to any compact generating set of $G_1$ (the integrability condition does not depend on the choice).

We say that two compactly generated groups $G_1$ and $G_2$ are $L_p$-measure equivalent if there exists a measure equivalence coupling that is $L_p$-integrable over both $G_1$ and $G_2$. Thanks to the construction in [Fur99, Theorem 3.3], we know that $L_p$-measure equivalence is more general than $L_p$-orbit equivalence.

More details on measure equivalence of locally compact groups and $L_p$-measure equivalence can be found in [BFS13, § 1.2] and the references therein.

Theorem 6.4 Let $G_1$ and $G_2$ be two locally compact groups. Let $1 \leq p < \infty$. Suppose that $G_1$ is compactly generated and that there exists a measure equivalence coupling between $G_1$ and $G_2$ that is $L_p$-integrable over $G_1$.

1. (i) If $G_1$ admits an affine isometric action without fixed points on some $L_p$-space, then so does $G_2$.

2. (ii) If $G_1$ admits a proper affine isometric action on some $L_p$-space, then so does $G_2$.

Proof. We use the standard tool of induction of Banach-space actions as in [BFGM07, § 8.b]. Let $(X,\mu )$ be measure equivalence coupling that is $L_p$-integrable over $G_1$. Let $p_1$ and $c_1$ be as in the definition.

Let $\alpha \colon G_1 \curvearrowright E$ be an action by affine isometries of $G_1$ on a Banach space $E$. Let $L_0(X,\mu,E)^{G_1}$ be the set of all $G_1$-equivariant Bochner-measurable maps from $X$ to $E$. Note that $L_0(X,\mu,E)^{G_1}$ is only an affine subspace of $L_0(X,\mu,E)$ (it does not contain $0$). Observe that we have a natural affine action $\beta : G_2 \curvearrowright L_0(X,\mu, E)^{G_1}$ given by $\beta _{g_2}(f)(x)=f(g_2^{-1}x)$ for all $x \in X$. If $f,h \in L_0(X,\mu,E)^{G_1}$, then the map $\| f-h\|_E : X \rightarrow \mathbf {R}_+$ is $G_1$-invariant and, thus, we can define

\[ \| f-h\|_{p,G_1} = \bigg( \int_{X/G_1} \|f-h\|_E^p \,{d}\mu_1 \bigg)^{1/p}. \]

We clearly have $\| \beta _{g_2}(f)-\beta _{g_2}(h) \| = \| f-h\|$ for all $g_2 \in G_2$ (because the action of $G_2$ on $X/G_1$ preserves the measure).

Take $x \in E$ and define $f_0 \in L_0(X,\mu,E)^{G_1}$ by the formula $f_0(\omega )=p_1(\omega ) \cdot x$. Now, define an affine subspace

\[ F=\{ f \in L_0(X,\mu,E)^{G_1} \mid \| f-f_0\|_{p,G_1} < \infty\}. \]

Note that the space $F$ is isometric to $L_p( X/G_1,\mu _1, E)$. In particular, if $E$ is an $L_p$-space, then $F$ is also an $L_p$-space.

Now, a key observation is that $\beta _{g_2}(f_0) \in F$ for all $g_2 \in G_2$. Indeed, we have

\[ \| \beta_{g_2}(f_0) - f_0 \|_{p,G_1}=\bigg( \int_{X/G_1} \| c_1(g_2,\omega_1) \cdot x -x\|_E^p \,{d} \mu_1(\omega_1) \bigg)^{1/p} \]

and this integral is finite because of the $L_p$-integrability of $c_1$ and the fact that the function $g_1 \mapsto \| g_1 \cdot x-x\|_E$ grows sublinearly: there exists a constant $C > 0$ such that $\| g_1 \cdot x- x\|_E \leq C|g_1|_{G_1}$ for all $g_1 \in G_1$.

As $\beta _{g_2}(f_0) \in F$ for all $g_2 \in G_2$, it follows that $F$ is globally invariant under the action $\beta$. We conclude that the action $\beta : G_2 \curvearrowright L_0(X,\mu,E)^{G_1}$ restricts to an affine isometric action, still denoted $\beta$, of $G_2$ on $F$. We call the affine isometric action $\beta : G_2 \curvearrowright F$ the induced action of $\alpha$ (note that it depends on the choice of $p_1$).

To prove item (i), it is enough to check that if $\beta$ has a fixed point then $\alpha$ also has a fixed point. Let $f \in F$ be a fixed point for $\beta$. This means that $f : X \rightarrow E$ is a $G_1$-equivariant measurable map that is also $G_2$-invariant. Thus, we can view $f$ as a $G_1$-equivariant map from $X/G_2$ to $E$. As $X/G_2$ admits a $G_1$-invariant probability measure $\mu _2$, we can push it forward by $f$ to obtain a $G_1$-invariant probability measure on $E$. We conclude by [BFGM07, Lemma 2.14] that $\alpha$ has a fixed point.

To prove item (ii), it is enough to check that if $\alpha$ is proper, then $\beta$ is also proper. We have

\[ \| \beta_{g_2}(f_0) - f_0 \|_{p,G_1} = \bigg(\int_{X/G_1} \| c_1(g_2,\omega_1) \cdot x-x \|_E^p \,{d}\mu_1(\omega_1)\bigg)^{{1}/{p}}. \]

Take $R > 0$. As $\alpha$ is proper, we can choose a compact subset $K_1 \subset G_1$ such that $\| g_1 x-x\| \geq R$ for all $g_1 \in G_1 \setminus K_1$. Observe that

\begin{align*} & \mu_1 \{ \omega_1 \in X/G_1 \mid c(g_2, \omega_1) \in K_1 \} \\ &\quad =\frac{1}{m_{G_1}(K_1)} \mu \{ \omega \in X \mid p_1(\omega) \in K_1 \text{ and } p_1(g_2 \omega)^{-1}p_1(\omega) \in K_1 \} \\ &\quad \leq \frac{1}{m_{G_1}(K_1)} \mu \{ \omega \in X \mid p_1(\omega) \in K_1 \text{ and } p_1(g_2 \omega) \in K_1^{-1} K_1 \}. \end{align*}

As $\mu (p_1^{-1}(K_1 \cup K_1^{-1} K_1))<+\infty$, there exists a compact subset $K_2 \subset G_2$ such that $\mu (p_1^{-1}(K_1 \cup K_1^{-1}K_1) \setminus p_2^{-1}(K_2) ) \leq \frac {1}{4} m_{G_1}(K_1)$. Take $g_2 \in G_2 \setminus K_2 K_2^{-1}$. Then, we cannot have $p_2(\omega ) \in K_2$ $p_2(g_2 \omega ) \in K_2$ at the same time. Therefore, we obtain

\[ \mu \{ \omega \in X \mid p_1(\omega) \in K_1 \text{ and } p_1(g_2 \omega) \in K_1^{-1} K_1 \} \leq 2 \mu(p_1^{-1}(K_1 \cup K_1^{-1}K_1) \setminus p_2^{-1}(K_2) ), \]

which yields

\[ \mu_1 \{ \omega_1 \in X/G_1 \mid c(g_2, \omega_1) \in K_1 \} \leq \tfrac{1}{2}. \]

By the choice of $K_1$, this means that

\[ \mu_1 \{ \omega_1 \in X/G_1 \mid \| c(g_2, \omega_1) \cdot x- x\|_E \geq R\} \geq \tfrac{1}{2}, \]

hence

\[ \| \beta_{g_2}(f_0)-f_0 \|_{p,G_1} \geq 2^{-1/p} R. \]

This holds for all $g_2 \in G_2$ outside of the compact subset $K_2 K_2^{-1}$. We conclude that $\beta$ is proper.

When $G$ is a locally compact group and $\Gamma < G$ is a lattice, then $(G,m_G)$ is measure equivalence coupling between $G$ and $\Gamma$. Therefore, Theorem 6.4 covers, in particular, the following corollary which is already implicit in [BFGM07].

Corollary 6.5 [BFGM07]

Let $G$ be a locally compact compactly generated group and let $\Gamma < G$ be a lattice. Then $p_{\Gamma } \leq p_G$ and $p'_{\Gamma } \leq p'_G$. If $\Gamma$ is $L_p$-integrable for some $p \geq p_\Gamma$ (respectively, $p \geq p'_\Gamma$), then $p_G=p_{\Gamma }$ (respectively, $p'_G=p'_{\Gamma }$).