Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Cascini, Paolo
2021.
New directions in the Minimal Model Program.
Bollettino dell'Unione Matematica Italiana,
Vol. 14,
Issue. 1,
p.
179.
Cascini, Paolo
and
Spicer, Calum
2021.
MMP for co-rank one foliations on threefolds.
Inventiones mathematicae,
Vol. 225,
Issue. 2,
p.
603.
Druel, Stéphane
2021.
Codimension 1 foliations with numerically trivial canonical class on singular spaces.
Duke Mathematical Journal,
Vol. 170,
Issue. 1,
Figueredo, João Paulo
2022.
Del Pezzo foliations with log canonical singularities.
Journal of Pure and Applied Algebra,
Vol. 226,
Issue. 5,
p.
106926.
Spicer, Calum
and
Svaldi, Roberto
2022.
Effective generation for foliated surfaces: Results and applications.
Journal für die reine und angewandte Mathematik (Crelles Journal),
Vol. 0,
Issue. 0,
Druel, Stéphane
and
Ou, Wenhao
2022.
Codimension One Foliations with Numerically Trivial Canonical Class on Singular Spaces II.
International Mathematics Research Notices,
Vol. 2022,
Issue. 20,
p.
15574.
Wang, Juanyong
2022.
Structure of projective varieties with nef anticanonical divisor: the case of log terminal singularities.
Mathematische Annalen,
Vol. 384,
Issue. 1-2,
p.
1.
Figueredo, João Paulo
2022.
Codimension One Regular Foliations on Rationally Connected Threefolds.
Bulletin of the Brazilian Mathematical Society, New Series,
Vol. 53,
Issue. 4,
p.
1131.
Golota, Aleksei
2023.
Toward classification of codimension 1 foliations on threefolds of general type.
Mathematische Nachrichten,
Vol. 296,
Issue. 11,
p.
5012.
Etesse, Antoine
2023.
Geometric Generalized Wronskians: Applications in Intermediate Hyperbolicity and Foliation Theory.
International Mathematics Research Notices,
Vol. 2023,
Issue. 10,
p.
8251.
Wang, Weikun
2023.
Toric foliated minimal model program.
Journal of Algebra,
Vol. 632,
Issue. ,
p.
70.
Liu, Jihao
Luo, Yujie
and
Meng, Fanjun
2023.
On global ACC for foliated threefolds.
Transactions of the American Mathematical Society,
Cascini, Paolo
and
Spicer, Calum
2024.
Foliation adjunction.
Mathematische Annalen,
Mendson, Wodson
and
Pereira, Jorge Vitório
2024.
CODIMENSION ONE FOLIATIONS IN POSITIVE CHARACTERISTIC.
Journal of the Institute of Mathematics of Jussieu,
Vol. 23,
Issue. 4,
p.
1705.
Liu, Jihao
Meng, Fanjun
and
Xie, Lingyao
2024.
Complements, index theorem, and minimal log discrepancies of foliated surface singularities.
European Journal of Mathematics,
Vol. 10,
Issue. 1,
Liu, Jihao
Meng, Fanjun
and
Xie, Lingyao
2024.
Uniform rational polytopes of foliated threefolds and the global ACC.
Journal of the London Mathematical Society,
Vol. 109,
Issue. 6,
Bernasconi, Fabio
2024.
Counterexamples to the MMP for 1-foliations in positive characteristic.
ANNALI DELL'UNIVERSITA' DI FERRARA,
Vol. 70,
Issue. 3,
p.
631.
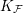 $K_{{\mathcal{F}}}$ for rank 2 foliations on threefolds. We also make progress toward realizing a minimal model program (MMP) for rank 2 foliations on threefolds.
$K_{{\mathcal{F}}}$ for rank 2 foliations on threefolds. We also make progress toward realizing a minimal model program (MMP) for rank 2 foliations on threefolds.