No CrossRef data available.
Published online by Cambridge University Press: 25 April 2023
For a fixed infinite graph 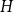 $H$, we study the largest density of a monochromatic subgraph isomorphic to
$H$, we study the largest density of a monochromatic subgraph isomorphic to  $H$ that can be found in every two-colouring of the edges of
$H$ that can be found in every two-colouring of the edges of 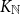 $K_{\mathbb{N}}$. This is called the Ramsey upper density of
$K_{\mathbb{N}}$. This is called the Ramsey upper density of  $H$ and was introduced by Erdős and Galvin in a restricted setting, and by DeBiasio and McKenney in general. Recently [4], the Ramsey upper density of the infinite path was determined. Here, we find the value of this density for all locally finite graphs
$H$ and was introduced by Erdős and Galvin in a restricted setting, and by DeBiasio and McKenney in general. Recently [4], the Ramsey upper density of the infinite path was determined. Here, we find the value of this density for all locally finite graphs  $H$ up to a factor of 2, answering a question of DeBiasio and McKenney. We also find the exact density for a wide class of bipartite graphs, including all locally finite forests. Our approach relates this problem to the solution of an optimisation problem for continuous functions. We show that, under certain conditions, the density depends only on the chromatic number of
$H$ up to a factor of 2, answering a question of DeBiasio and McKenney. We also find the exact density for a wide class of bipartite graphs, including all locally finite forests. Our approach relates this problem to the solution of an optimisation problem for continuous functions. We show that, under certain conditions, the density depends only on the chromatic number of 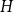 $H$, the number of components of
$H$, the number of components of 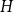 $H$ and the expansion ratio
$H$ and the expansion ratio 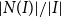 $|N(I)|/|I|$ of the independent sets of
$|N(I)|/|I|$ of the independent sets of  $H$.
$H$.
Funded from the European Research Council (ERC) under the European Union’s Horizon 2020 research and innovation programme (grant agreement No 648509). This publication reflects only its author’s view; the European Research Council Executive Agency is not responsible for any use that may be made of the information it contains. Also supported by the MUNI Award in Science and Humanities of the Grant Agency of Masaryk University.
Former affiliation: Freie Universität Berlin