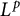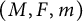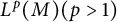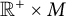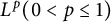1 Introduction
Finsler geometry is a Riemannian geometry without quadratic restriction. It is a natural generalization of Riemannian geometry. Studies show that there are similarities but essential differences between Riemannian and Finsler geometry [Reference Ohta and SturmOS1, Reference Ohta and SturmOS2, Reference ShenSh, Reference XiaXia1, Reference XiaXia4, Reference Zhang and XiaZX]. One of the challenges is the nonlinearity of the Laplacian and the associated heat equation. Some questions related to nonlinear equations would be of interest in itself from the analytic viewpoint.
A Finsler manifold
![]() $(M, F)$
means a smooth manifold M equipped with a Finsler metric (or Finsler structure)
$(M, F)$
means a smooth manifold M equipped with a Finsler metric (or Finsler structure)
![]() $F: TM\rightarrow [0, +\infty )$
such that
$F: TM\rightarrow [0, +\infty )$
such that
![]() $F_x=F|_{T_xM}$
is a Minkowski norm on
$F_x=F|_{T_xM}$
is a Minkowski norm on
![]() $T_xM$
at each point
$T_xM$
at each point
![]() $x\in M$
, that is to say, F is smooth on
$x\in M$
, that is to say, F is smooth on
![]() $TM\kern1pt{\backslash}\kern1pt \{0\}$
and
$TM\kern1pt{\backslash}\kern1pt \{0\}$
and
![]() $F_x(y)=0$
if and only if
$F_x(y)=0$
if and only if
![]() $y=0$
, F satisfies
$y=0$
, F satisfies
![]() $F(x, \lambda y)=\lambda F(x,y)$
for all
$F(x, \lambda y)=\lambda F(x,y)$
for all
![]() $\lambda>0$
, and the matrix
$\lambda>0$
, and the matrix
![]() $\left (g_{ij}(x, y)\right ):=\left (\frac 12(F^2)_{y^iy^j}\right )$
is positive definite for any nonzero
$\left (g_{ij}(x, y)\right ):=\left (\frac 12(F^2)_{y^iy^j}\right )$
is positive definite for any nonzero
![]() $y\in T_x M$
. Such a pair
$y\in T_x M$
. Such a pair
![]() $(M, F)$
is called a Finsler manifold. Given a smooth measure m, the triple
$(M, F)$
is called a Finsler manifold. Given a smooth measure m, the triple
![]() $(M, F, m)$
is called a Finsler measure space. In particular, if F is only a function of
$(M, F, m)$
is called a Finsler measure space. In particular, if F is only a function of
![]() $x\in M$
independing of y, then it is Riemannian. A Finsler measure space is not a metric space in usual sense because F may be nonreversible, i.e.,
$x\in M$
independing of y, then it is Riemannian. A Finsler measure space is not a metric space in usual sense because F may be nonreversible, i.e.,
![]() $F(x, y)\neq F(x, -y)$
may happen. This nonreversibility causes the asymmetry of the associated distance function. For any Finsler metric F, its dual
$F(x, y)\neq F(x, -y)$
may happen. This nonreversibility causes the asymmetry of the associated distance function. For any Finsler metric F, its dual
![]() $F^*(x, \xi )=\sup _{F_x(y)=1}\xi (y)$
is also a Finsler metric on M, where
$F^*(x, \xi )=\sup _{F_x(y)=1}\xi (y)$
is also a Finsler metric on M, where
![]() $\xi \in T_{x}^{*}(M)$
.
$\xi \in T_{x}^{*}(M)$
.
In general, the space of functions
![]() $u\in L^p(M)$
with
$u\in L^p(M)$
with
![]() $\int _M [F^*(du)]^pdm<\infty $
with respect to the measure m is not a linear space over
$\int _M [F^*(du)]^pdm<\infty $
with respect to the measure m is not a linear space over
![]() $\mathbb {R}$
because of the nonreversibility of F [Reference Kristály and RudasKR]. We define
$\mathbb {R}$
because of the nonreversibility of F [Reference Kristály and RudasKR]. We define
for
![]() $p\geq 1$
. Thus,
$p\geq 1$
. Thus,
![]() $ W^{1, p}(M)$
is a Banach space with respect to the norm
$ W^{1, p}(M)$
is a Banach space with respect to the norm
It is a Sobolev space, which is the completion of the space
![]() $C^\infty (M)$
with respect to the Sobolev norm
$C^\infty (M)$
with respect to the Sobolev norm
![]() $\|\cdot \|_{1, p}$
. Further, we may define
$\|\cdot \|_{1, p}$
. Further, we may define
![]() $W_0^{1, p}(M)$
as the completion of the space
$W_0^{1, p}(M)$
as the completion of the space
![]() $C_0^\infty (M)$
with respect to the norm
$C_0^\infty (M)$
with respect to the norm
![]() $\|\cdot \|_{1, p}$
, and hence
$\|\cdot \|_{1, p}$
, and hence
![]() $(W_0^{1, p}(M), \|u\|_{1, p})$
is also a Sobolev space. We denote
$(W_0^{1, p}(M), \|u\|_{1, p})$
is also a Sobolev space. We denote
![]() $H^1(M):=W^{1,2}(M)$
and
$H^1(M):=W^{1,2}(M)$
and
![]() $H^1_0(M): =W^{1, 2}_0(M)$
for simplicity. Likewise,
$H^1_0(M): =W^{1, 2}_0(M)$
for simplicity. Likewise,
![]() $H^{-1}(M)$
means the dual Banach space of
$H^{-1}(M)$
means the dual Banach space of
![]() $H_0^1(M)$
.
$H_0^1(M)$
.
For any
![]() $T>0$
(T maybe the infinity), a function
$T>0$
(T maybe the infinity), a function
![]() $u\in [0, T]\times M$
is called a global (super, sub)solution to the heat equation
$u\in [0, T]\times M$
is called a global (super, sub)solution to the heat equation
![]() $\partial _tu=\mathbf \Delta u$
if it satisfies
$\partial _tu=\mathbf \Delta u$
if it satisfies
![]() $\mathrm {(i)}$
$\mathrm {(i)}$
![]() $u\in L^2\left ([0, T], H_0^1(M)\right )\cap H^1\left ([0, T], H^{-1}(M)\right )$
and
$u\in L^2\left ([0, T], H_0^1(M)\right )\cap H^1\left ([0, T], H^{-1}(M)\right )$
and
![]() $\mathrm {(ii)}$
for almost every
$\mathrm {(ii)}$
for almost every
![]() $t\in (0, T)$
and
$t\in (0, T)$
and
![]() $0\leq \phi \in C_0^\infty (M)$
(or, equivalently, for every
$0\leq \phi \in C_0^\infty (M)$
(or, equivalently, for every
![]() $\phi \in H_0^1(M)$
), it holds that
$\phi \in H_0^1(M)$
), it holds that
The existence and regularity of the global solution to the heat equation have been studied in [Reference Ohta and SturmOS1] (see Section 2). Moreover, global solutions are constructed as gradient curves of the energy functional
![]() $\mathcal E(u)=\int _M F^2(\nabla u)dm$
on
$\mathcal E(u)=\int _M F^2(\nabla u)dm$
on
![]() $u\in H^1_{loc}(M)$
in the Hilbert space
$u\in H^1_{loc}(M)$
in the Hilbert space
![]() $L^2(M)$
[Reference Ohta and SturmOS1]. Comparing with the Riemannian case [Reference Schoen and YauSY], there are less results on the heat equation in Finsler geometry. An important progress is that Ohta and Sturm gave Li-Yau's gradient estimate for the positive solutions to the heat equation on a closed Finsler manifold with weighted Ricci curvature bounded below by establishing the Bochner–Weitzenböck formula in [Reference Ohta and SturmOS2]. Recently, the author of the present paper gave the local and global Li-Yau's type gradient estimates on complete Finsler measure spaces with weighted Ricci curvature bounded below in [Reference XiaXia2] and [Reference XiaXia3] by Moser's iteration and a linearized heat semigroup approach. In this paper, we will focus on the uniqueness of nonnegative
$L^2(M)$
[Reference Ohta and SturmOS1]. Comparing with the Riemannian case [Reference Schoen and YauSY], there are less results on the heat equation in Finsler geometry. An important progress is that Ohta and Sturm gave Li-Yau's gradient estimate for the positive solutions to the heat equation on a closed Finsler manifold with weighted Ricci curvature bounded below by establishing the Bochner–Weitzenböck formula in [Reference Ohta and SturmOS2]. Recently, the author of the present paper gave the local and global Li-Yau's type gradient estimates on complete Finsler measure spaces with weighted Ricci curvature bounded below in [Reference XiaXia2] and [Reference XiaXia3] by Moser's iteration and a linearized heat semigroup approach. In this paper, we will focus on the uniqueness of nonnegative
![]() $L^p(p>0)$
subsolutions to the heat equation on forward complete Finsler measure spaces.
$L^p(p>0)$
subsolutions to the heat equation on forward complete Finsler measure spaces.
For the case when
![]() $p>1$
, the uniqueness of
$p>1$
, the uniqueness of
![]() $L^p$
nonnegative subsolutions to the heat equation is determined by the initial data on a complete Finsler manifold. More precisely, we have the following theorem.
$L^p$
nonnegative subsolutions to the heat equation is determined by the initial data on a complete Finsler manifold. More precisely, we have the following theorem.
Theorem 1.1 Let
![]() $(M, F, m)$
be a forward or backward complete Finsler measure space. If
$(M, F, m)$
be a forward or backward complete Finsler measure space. If
![]() $u(t, x)$
is a nonnegative global subsolution to the heat equation on
$u(t, x)$
is a nonnegative global subsolution to the heat equation on
![]() $[0, \infty ) \times M$
with
$[0, \infty ) \times M$
with
![]() $u(t, x)\in L^p(M)$
and
$u(t, x)\in L^p(M)$
and
![]() $\lim \limits _{t\rightarrow 0}\int _Mu^p(t, x)dm=0$
for all
$\lim \limits _{t\rightarrow 0}\int _Mu^p(t, x)dm=0$
for all
![]() $t>0$
and
$t>0$
and
![]() $p>1$
, then
$p>1$
, then
![]() $u(t, x)\equiv 0$
on
$u(t, x)\equiv 0$
on
![]() $[0, \infty ) \times M$
.
$[0, \infty ) \times M$
.
For the case when
![]() $0<p\leq 1$
, we have the following theorem.
$0<p\leq 1$
, we have the following theorem.
Theorem 1.2 Let
![]() $(M, F, m)$
be an n-dimensional forward or backward complete and noncompact Finsler measure space with finite reversibility
$(M, F, m)$
be an n-dimensional forward or backward complete and noncompact Finsler measure space with finite reversibility
![]() $\Lambda $
. If Ric
$\Lambda $
. If Ric
![]() $_N\geq 0$
for some
$_N\geq 0$
for some
![]() $N\in [n, \infty )$
, then every nonnegative
$N\in [n, \infty )$
, then every nonnegative
![]() $L^p(\mathbb R\times M)(0<p\leq 2)$
subsolution to the heat equation on
$L^p(\mathbb R\times M)(0<p\leq 2)$
subsolution to the heat equation on
![]() $\mathbb R\times M$
is identically zero.
$\mathbb R\times M$
is identically zero.
When
![]() $(M, F=\sqrt {g}, dV_g)$
is Riemannian and
$(M, F=\sqrt {g}, dV_g)$
is Riemannian and
![]() $N=n$
, Theorem 1.1 is reduced to the result in [Reference LiLi]. Since the Finsler Laplacian is nonlinear, we use some nonlinear approaches to prove Theorem 1.2. In fact, we shall establish the local
$N=n$
, Theorem 1.1 is reduced to the result in [Reference LiLi]. Since the Finsler Laplacian is nonlinear, we use some nonlinear approaches to prove Theorem 1.2. In fact, we shall establish the local
![]() $L^p$
mean value inequality for the heat equation by means of Moser’s iteration to prove this. In particular, if the (super, sub)solution u to the heat equation is independent of t, then u is a Finsler (super, sub)harmonic function. In this case, some Liouville-type theorems have been established in [Reference XiaXc, Reference XiaXia1, Reference XiaXia4, Reference Zhang and XiaZX].
$L^p$
mean value inequality for the heat equation by means of Moser’s iteration to prove this. In particular, if the (super, sub)solution u to the heat equation is independent of t, then u is a Finsler (super, sub)harmonic function. In this case, some Liouville-type theorems have been established in [Reference XiaXc, Reference XiaXia1, Reference XiaXia4, Reference Zhang and XiaZX].
2 Preliminaries
In this section, we briefly review some basic concepts in Finsler geometry. For more details, we refer to [Reference ShenSh].
2.1 Finsler metrics and curvatures
Let
![]() $(M, F)$
be an n-dimensional Finsler manifold, and let
$(M, F)$
be an n-dimensional Finsler manifold, and let
![]() $TM$
be the tangent bundle of M. We define the reverse metric
$TM$
be the tangent bundle of M. We define the reverse metric
![]() $\overleftarrow {F}$
of F by
$\overleftarrow {F}$
of F by
![]() $\overleftarrow {F}(x,y):=F(x,-y)$
for all
$\overleftarrow {F}(x,y):=F(x,-y)$
for all
![]() $(x, y)\in TM$
. It is easy to see that
$(x, y)\in TM$
. It is easy to see that
![]() $\overleftarrow F$
is also a Finsler metric on M. A Finsler metric F on M is said to be reversible if
$\overleftarrow F$
is also a Finsler metric on M. A Finsler metric F on M is said to be reversible if
![]() $\overleftarrow {F}(x,y)=F(x, y)$
for all
$\overleftarrow {F}(x,y)=F(x, y)$
for all
![]() $y\in TM$
. Otherwise, we say F is nonreversible. In this case, we define the reversibility
$y\in TM$
. Otherwise, we say F is nonreversible. In this case, we define the reversibility
![]() $\Lambda =\Lambda (M, F)$
of F by
$\Lambda =\Lambda (M, F)$
of F by
 $$ \begin{align*}\Lambda:= \sup\limits_{(x, y)\in TM\kern1pt{\backslash}\kern1pt\{0\}}\frac{F(x, -y)}{F(x, y)}.\end{align*} $$
$$ \begin{align*}\Lambda:= \sup\limits_{(x, y)\in TM\kern1pt{\backslash}\kern1pt\{0\}}\frac{F(x, -y)}{F(x, y)}.\end{align*} $$
Obviously,
![]() $\Lambda \in [1, \infty ]$
and F is reversible if and only if
$\Lambda \in [1, \infty ]$
and F is reversible if and only if
![]() $\Lambda =1$
.
$\Lambda =1$
.
Given a nonvanishing smooth vector field V, one introduces a weighted Riemannian metric
![]() $g_V$
on M given by
$g_V$
on M given by
Thus, we have
![]() $F^2(V)=g_V(V, V)$
.
$F^2(V)=g_V(V, V)$
.
For
![]() $x_1, x_2\in M$
, the distance from
$x_1, x_2\in M$
, the distance from
![]() $x_1$
to
$x_1$
to
![]() $x_2$
is defined by
$x_2$
is defined by
![]() $d_F(x_1, x_2):= \inf _{\gamma }\int _0^1F(\dot {\gamma }(t))dt$
, where the infimum is taken over all
$d_F(x_1, x_2):= \inf _{\gamma }\int _0^1F(\dot {\gamma }(t))dt$
, where the infimum is taken over all
![]() $C^1$
curves
$C^1$
curves
![]() $\gamma : [0,1]\rightarrow M$
such that
$\gamma : [0,1]\rightarrow M$
such that
![]() $\gamma (0)=x_1$
and
$\gamma (0)=x_1$
and
![]() $\gamma (1)=x_2$
. Note that
$\gamma (1)=x_2$
. Note that
![]() $d_F(x_1, x_2)\neq d_F(x_2, x_1)$
unless F is reversible.
$d_F(x_1, x_2)\neq d_F(x_2, x_1)$
unless F is reversible.
![]() $d_F(x, \cdot )$
is differentiable almost everywhere on M and
$d_F(x, \cdot )$
is differentiable almost everywhere on M and
![]() $F(\nabla d_F)=1$
at points where
$F(\nabla d_F)=1$
at points where
![]() $d_F$
is differentiable. If F has finite reversibility
$d_F$
is differentiable. If F has finite reversibility
![]() $\Lambda $
, then
$\Lambda $
, then
Now we define the forward and backward geodesic balls of radius R with center at
![]() $x\in M$
by
$x\in M$
by
A
![]() $C^1$
curve
$C^1$
curve
![]() $\eta : [0, \ell ]\rightarrow M$
is called a geodesic if it has constant speed (i.e.,
$\eta : [0, \ell ]\rightarrow M$
is called a geodesic if it has constant speed (i.e.,
![]() $F(\eta , \dot \eta )$
is constant) and if it is locally minimizing. Such a geodesic is in fact a
$F(\eta , \dot \eta )$
is constant) and if it is locally minimizing. Such a geodesic is in fact a
![]() $C^\infty $
curve. A Finsler manifold
$C^\infty $
curve. A Finsler manifold
![]() $(M, F)$
is said to be forward complete (or backward complete) if each geodesic defined on
$(M, F)$
is said to be forward complete (or backward complete) if each geodesic defined on
![]() $[0, \ell )$
(or
$[0, \ell )$
(or
![]() $(-\ell , 0]$
) can be extended to a geodesic defined on
$(-\ell , 0]$
) can be extended to a geodesic defined on
![]() $[0, \infty )$
(or
$[0, \infty )$
(or
![]() $(-\infty , 0]$
).
$(-\infty , 0]$
).
![]() $(M, F)$
is backward complete if and only if
$(M, F)$
is backward complete if and only if
![]() $(M, \overleftarrow F)$
is forward complete. We say that
$(M, \overleftarrow F)$
is forward complete. We say that
![]() $(M, F)$
is complete if it is both forward complete and backward complete [Reference ShenSh].
$(M, F)$
is complete if it is both forward complete and backward complete [Reference ShenSh].
Given a smooth measure m, write
![]() $dm=\sigma (x)dx$
. For any
$dm=\sigma (x)dx$
. For any
![]() $v\in T_{x}M\kern1pt{\backslash}\kern1pt \{0\}$
, let
$v\in T_{x}M\kern1pt{\backslash}\kern1pt \{0\}$
, let
![]() $\eta (t)$
be a geodesic with
$\eta (t)$
be a geodesic with
![]() $\eta (0)=x$
and
$\eta (0)=x$
and
![]() $\dot {\eta }(0)=v$
. The distortion of F is defined by
$\dot {\eta }(0)=v$
. The distortion of F is defined by
![]() $ \tau (x, v):=\log \left \{ \frac {\sqrt {\mathrm{det}(g_{ij}(x, v))}}{\sigma (x)}\right \},$
and the S-curvature S is defined as the rate of change of the distortion
$ \tau (x, v):=\log \left \{ \frac {\sqrt {\mathrm{det}(g_{ij}(x, v))}}{\sigma (x)}\right \},$
and the S-curvature S is defined as the rate of change of the distortion
![]() $\tau $
along
$\tau $
along
![]() $\eta (t)$
, i.e.,
$\eta (t)$
, i.e.,
S-curvature disappears on a Riemannian manifold
![]() $(M, g)$
.
$(M, g)$
.
Next we recall the definition of the weighted Ricci curvature on a Finsler manifold, which was introduced by S. Ohta.
Definition 2.1 [Reference Ohta FinslerOh] Given a unit vector
![]() $v\in T_{x}M$
, let
$v\in T_{x}M$
, let
![]() $\eta :(-\varepsilon ,\varepsilon )\rightarrow M$
be the geodesic with
$\eta :(-\varepsilon ,\varepsilon )\rightarrow M$
be the geodesic with
![]() $\eta (0)=x$
and
$\eta (0)=x$
and
![]() $\dot \eta (0)=v$
. We set
$\dot \eta (0)=v$
. We set
![]() $dm =e^{-\Psi (\eta (t))} \mathrm{vol}_{\dot \eta }$
along
$dm =e^{-\Psi (\eta (t))} \mathrm{vol}_{\dot \eta }$
along
![]() $\eta $
, where
$\eta $
, where
![]() $ {vol}_{\dot \eta }$
is the volume form of
$ {vol}_{\dot \eta }$
is the volume form of
![]() $g_{\dot \eta }$
. Define the weighted Ricci curvature involving a parameter
$g_{\dot \eta }$
. Define the weighted Ricci curvature involving a parameter
![]() $N\in (n,\infty )$
by
$N\in (n,\infty )$
by
 $$ \begin{align*}\mathrm{Ric}_N(v):=\mathrm{Ric}(v)+{(\Psi\circ \eta)^{"}(0)}-\frac{(\Psi\circ \eta)^{\prime}(0)^{2}}{(N-n)},\end{align*} $$
$$ \begin{align*}\mathrm{Ric}_N(v):=\mathrm{Ric}(v)+{(\Psi\circ \eta)^{"}(0)}-\frac{(\Psi\circ \eta)^{\prime}(0)^{2}}{(N-n)},\end{align*} $$
where Ric is the Ricci curvature of F. Also, define Ric
![]() $_\infty (v):= \mathrm{Ric}(v)+{(\Psi \circ \eta )^{"}(0)}$
and
$_\infty (v):= \mathrm{Ric}(v)+{(\Psi \circ \eta )^{"}(0)}$
and
 $$ \begin{align*} \mathrm{Ric}_n(v):=\lim \limits_{N\rightarrow n}\mathrm{Ric}_N=\left\{\begin{array}{ll} \mathrm{Ric}(v)+{(\Psi\circ \eta)^{"}(0)}, &\mathrm{if}\ (\Psi\circ \eta)^{'}(0)=0, \\ -\infty, &\mathrm{otherwise}\end{array} \right. \end{align*} $$
$$ \begin{align*} \mathrm{Ric}_n(v):=\lim \limits_{N\rightarrow n}\mathrm{Ric}_N=\left\{\begin{array}{ll} \mathrm{Ric}(v)+{(\Psi\circ \eta)^{"}(0)}, &\mathrm{if}\ (\Psi\circ \eta)^{'}(0)=0, \\ -\infty, &\mathrm{otherwise}\end{array} \right. \end{align*} $$
as limits. Finally, for any
![]() $\lambda \geq 0$
and
$\lambda \geq 0$
and
![]() $N\in [n, \infty ]$
, define
$N\in [n, \infty ]$
, define
![]() $ \mathrm{Ric}_N(\lambda v):=\lambda ^2 \mathrm{Ric}_N(v)$
.
$ \mathrm{Ric}_N(\lambda v):=\lambda ^2 \mathrm{Ric}_N(v)$
.
We say that
![]() $ \mathrm{Ric}_N\geq K$
for some
$ \mathrm{Ric}_N\geq K$
for some
![]() $K\in {\mathbb R}$
if
$K\in {\mathbb R}$
if
![]() $ \mathrm{Ric}_N(v)\geq KF^2(v)$
for all
$ \mathrm{Ric}_N(v)\geq KF^2(v)$
for all
![]() $v\in TM$
. We remark that
$v\in TM$
. We remark that
![]() $(\Psi \circ \eta )^{'}(0)=S(x, v)$
and
$(\Psi \circ \eta )^{'}(0)=S(x, v)$
and
![]() $(\Psi \circ \eta )^{"}(0)$
is exactly the change rate of the S-curvature along the geodesic
$(\Psi \circ \eta )^{"}(0)$
is exactly the change rate of the S-curvature along the geodesic
![]() $\eta (t)$
.
$\eta (t)$
.
2.2 Gradient and nonlinear Laplacian
Let
![]() $\mathcal L: T^*M \rightarrow TM$
be the Legendre transform associated with F and its dual norm
$\mathcal L: T^*M \rightarrow TM$
be the Legendre transform associated with F and its dual norm
![]() $F^*$
, that is, a transformation
$F^*$
, that is, a transformation
![]() $\mathcal L$
from
$\mathcal L$
from
![]() $T^*M$
to
$T^*M$
to
![]() $TM$
, defined locally by
$TM$
, defined locally by
![]() $\mathcal L(x, \xi )=J^i(x, \xi )\frac {\partial }{\partial x^i}$
,
$\mathcal L(x, \xi )=J^i(x, \xi )\frac {\partial }{\partial x^i}$
,
![]() $J^i(x, \xi ):=\frac 12[F^{*2}(x, \xi )]_{\xi _i}(x, \xi )$
, is sending
$J^i(x, \xi ):=\frac 12[F^{*2}(x, \xi )]_{\xi _i}(x, \xi )$
, is sending
![]() $\xi \in T^*M$
to a unique element
$\xi \in T^*M$
to a unique element
![]() $y\in T_xM$
such that
$y\in T_xM$
such that
![]() $F(x, y)=F^*(x, \xi )$
and
$F(x, y)=F^*(x, \xi )$
and
![]() $\xi (y)=F^2(y)$
[Reference ShenSh]. It is a diffeomorphism from
$\xi (y)=F^2(y)$
[Reference ShenSh]. It is a diffeomorphism from
![]() $T^*M\kern1pt{\backslash}\kern1pt \{0\}$
to
$T^*M\kern1pt{\backslash}\kern1pt \{0\}$
to
![]() $TM\kern1pt{\backslash}\kern1pt \{0\}$
preserving the norm.
$TM\kern1pt{\backslash}\kern1pt \{0\}$
preserving the norm.
For a smooth function
![]() $u :M \rightarrow \mathbb R$
, the gradient vector
$u :M \rightarrow \mathbb R$
, the gradient vector
![]() $\nabla u(x)$
of u is defined by
$\nabla u(x)$
of u is defined by
![]() $\nabla u :=\mathcal L(du)\in T_xM$
. Obviously,
$\nabla u :=\mathcal L(du)\in T_xM$
. Obviously,
![]() $\nabla u=0$
if
$\nabla u=0$
if
![]() $du=0$
. In a local coordinate system, we reexpress
$du=0$
. In a local coordinate system, we reexpress
![]() $\nabla u$
as
$\nabla u$
as
 $$ \begin{align*} \nabla u:=\left\{\begin{array}{l} g^{ij}(\nabla u)\frac{\partial u}{\partial x^i}\frac{\partial}{\partial x^j},\ \ \ \ x\in M_u,\\ 0,\ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ x\in M\kern1pt{\backslash}\kern1pt M_u, \end{array} \right. \end{align*} $$
$$ \begin{align*} \nabla u:=\left\{\begin{array}{l} g^{ij}(\nabla u)\frac{\partial u}{\partial x^i}\frac{\partial}{\partial x^j},\ \ \ \ x\in M_u,\\ 0,\ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ x\in M\kern1pt{\backslash}\kern1pt M_u, \end{array} \right. \end{align*} $$
where
![]() $M_u=\{x\in M|du(x)\neq 0\}$
. In general,
$M_u=\{x\in M|du(x)\neq 0\}$
. In general,
![]() $\nabla u$
is only continuous on M, but smooth on
$\nabla u$
is only continuous on M, but smooth on
![]() $M_u$
.
$M_u$
.
Recall that
![]() $dm=\sigma (x)dx$
. The Finsler Laplacian
$dm=\sigma (x)dx$
. The Finsler Laplacian
![]() $\Delta $
of a function u on M is formally defined by
$\Delta $
of a function u on M is formally defined by
![]() $\Delta u=\mbox {div}(\nabla u)$
. It should be understood in the sense that
$\Delta u=\mbox {div}(\nabla u)$
. It should be understood in the sense that
for any
![]() $u\in H^1_{\mathrm {{loc}}}(M)$
and
$u\in H^1_{\mathrm {{loc}}}(M)$
and
![]() $\varphi \in C^\infty _0(M)$
[Reference ShenSh]. Obviously,
$\varphi \in C^\infty _0(M)$
[Reference ShenSh]. Obviously,
![]() $\Delta $
is a nonlinear divergence-type operator. Locally,
$\Delta $
is a nonlinear divergence-type operator. Locally,
We say that a function u on
![]() $[0, T]\times M$
is a global (super, sub)solution to the heat equation
$[0, T]\times M$
is a global (super, sub)solution to the heat equation
![]() $\partial _tu=\Delta u$
if it satisfies (1.2). When
$\partial _tu=\Delta u$
if it satisfies (1.2). When
![]() $\Lambda <\infty $
, the global solutions enjoy the
$\Lambda <\infty $
, the global solutions enjoy the
![]() $H^2_{loc}$
-regularity in x as well as the
$H^2_{loc}$
-regularity in x as well as the
![]() $C^{1, \alpha }$
-regularity in both t and x on
$C^{1, \alpha }$
-regularity in both t and x on
![]() $(0, \infty )\times M$
. Moreover,
$(0, \infty )\times M$
. Moreover,
![]() $\partial _tu$
lies in
$\partial _tu$
lies in
![]() $H^1_{loc}(M)$
[Reference Ohta and SturmOS1]. We also may define the local solutions to the heat equation as follows.
$H^1_{loc}(M)$
[Reference Ohta and SturmOS1]. We also may define the local solutions to the heat equation as follows.
Given an open subset
![]() $\Omega \subset M$
and an open interval
$\Omega \subset M$
and an open interval
![]() $I\subset \mathbb R$
, we say that a function u on
$I\subset \mathbb R$
, we say that a function u on
![]() $I\times \Omega $
is a local (super, sub)solution to the heat equation
$I\times \Omega $
is a local (super, sub)solution to the heat equation
![]() $\partial _t u=\mathbf \Delta u$
on
$\partial _t u=\mathbf \Delta u$
on
![]() $I\times \Omega $
if
$I\times \Omega $
if
![]() $u\in L^2_{loc}(I\times \Omega )$
with
$u\in L^2_{loc}(I\times \Omega )$
with
![]() $F^*(du)\in L^2_{loc}(I\times \Omega )$
, and for any
$F^*(du)\in L^2_{loc}(I\times \Omega )$
, and for any
![]() $\phi \in C_0^{\infty }(I\times \Omega )$
(or, equivalently, for any
$\phi \in C_0^{\infty }(I\times \Omega )$
(or, equivalently, for any
![]() $\phi \in H_0^1(I\times \Omega )$
),
$\phi \in H_0^1(I\times \Omega )$
),
Every continuous local solution to the heat equation
![]() $\partial _tu=\mathbf \Delta u$
on
$\partial _tu=\mathbf \Delta u$
on
![]() $I\times \Omega $
is
$I\times \Omega $
is
![]() $C^{1, \beta }$
in t and x [Reference Ohta and SturmOS1].
$C^{1, \beta }$
in t and x [Reference Ohta and SturmOS1].
3 Mean value inequality
In this section, we establish the local
![]() $L^p(p>0)$
mean value inequalities for nonnegative subsolutions to the heat equation on Finsler measure spaces
$L^p(p>0)$
mean value inequalities for nonnegative subsolutions to the heat equation on Finsler measure spaces
![]() $(M, F, m)$
. Let us first recall the local Sobolev inequality, which was due to Xia [Reference XiaXc]. It is worth mentioning that it suffices to assume the finite reversibility instead of the uniform convexity and uniform smoothness for the Finsler metrics from the proof of Theorem 3.3 in [Reference XiaXc]. For the sake of simplicity, we shall denote by
$(M, F, m)$
. Let us first recall the local Sobolev inequality, which was due to Xia [Reference XiaXc]. It is worth mentioning that it suffices to assume the finite reversibility instead of the uniform convexity and uniform smoothness for the Finsler metrics from the proof of Theorem 3.3 in [Reference XiaXc]. For the sake of simplicity, we shall denote by
![]() $B_R:=B^+_R(x_0)$
the forward geodesic ball of radius R centered at some point
$B_R:=B^+_R(x_0)$
the forward geodesic ball of radius R centered at some point
![]() $x_0\in M$
.
$x_0\in M$
.
Lemma 3.1 [Reference XiaXc] Let
![]() $(M, F, m)$
be a forward complete Finsler measure space with finite reversibility
$(M, F, m)$
be a forward complete Finsler measure space with finite reversibility
![]() $\Lambda $
satisfying Ric
$\Lambda $
satisfying Ric
![]() $_N\geq -K$
for some
$_N\geq -K$
for some
![]() $K>0$
. Then there exist constants
$K>0$
. Then there exist constants
![]() $\nu =\nu (N)>2$
and
$\nu =\nu (N)>2$
and
![]() $c_0=c_0(N, \Lambda )$
depending on N and
$c_0=c_0(N, \Lambda )$
depending on N and
![]() $\Lambda $
, such that
$\Lambda $
, such that
 $$ \begin{align} \left(\int_{B_R}|u|^{\frac {2\nu}{\nu-2}}dm\right)^{\frac {\nu-2}{\nu}}\leq e^{c_0(1+\sqrt{K}R)}R^2m(B_R)^{-\frac 2\nu}\int_{B_R}\{F^{*2}(x, du)+R^{-2}u^2\}dm \end{align} $$
$$ \begin{align} \left(\int_{B_R}|u|^{\frac {2\nu}{\nu-2}}dm\right)^{\frac {\nu-2}{\nu}}\leq e^{c_0(1+\sqrt{K}R)}R^2m(B_R)^{-\frac 2\nu}\int_{B_R}\{F^{*2}(x, du)+R^{-2}u^2\}dm \end{align} $$
for any forward geodesic ball
![]() $B_R$
and
$B_R$
and
![]() $u\in W^{1, 2}_{loc}(M)$
.
$u\in W^{1, 2}_{loc}(M)$
.
With this, we can prove the local
![]() $L^2$
mean value inequality for nonnegative subsolutions to the heat equation.
$L^2$
mean value inequality for nonnegative subsolutions to the heat equation.
Proposition 3.1 Let
![]() $(M, F, m)$
be an n-dimensional forward complete Finsler measure space with finite reversibility
$(M, F, m)$
be an n-dimensional forward complete Finsler measure space with finite reversibility
![]() $\Lambda $
. Fix
$\Lambda $
. Fix
![]() $R>0$
, and assume that (3.1) holds. If u is a nonnegative local subsolution of the heat equation in the cylinder
$R>0$
, and assume that (3.1) holds. If u is a nonnegative local subsolution of the heat equation in the cylinder
![]() $Q: =(s-r^2, s+\varepsilon )\times B^+_r(x_0)$
for any
$Q: =(s-r^2, s+\varepsilon )\times B^+_r(x_0)$
for any
![]() $s\in \mathbb R$
and
$s\in \mathbb R$
and
![]() $0<r<R$
, where
$0<r<R$
, where
![]() $\varepsilon $
is a positive constant, then there are constants
$\varepsilon $
is a positive constant, then there are constants
![]() $\nu>2$
, and
$\nu>2$
, and
![]() $c=c(N, \nu , \Lambda )>0$
such that for any
$c=c(N, \nu , \Lambda )>0$
such that for any
![]() $0<\delta <\delta '\leq 1$
, we have
$0<\delta <\delta '\leq 1$
, we have
 $$ \begin{align} \sup\limits_{Q_{\delta}}u^2\leq \frac{e^{c(1+r\sqrt{K})}}{(\delta'-\delta)^{2+\nu}r^{2} m(B_r^+(x_0))}\int_{Q_{\delta'}} u^2dmdt, \end{align} $$
$$ \begin{align} \sup\limits_{Q_{\delta}}u^2\leq \frac{e^{c(1+r\sqrt{K})}}{(\delta'-\delta)^{2+\nu}r^{2} m(B_r^+(x_0))}\int_{Q_{\delta'}} u^2dmdt, \end{align} $$
where
![]() $Q_{\delta }:=(s-\delta r^2, s]\times B^+_{\delta r}(x_0)$
and
$Q_{\delta }:=(s-\delta r^2, s]\times B^+_{\delta r}(x_0)$
and
![]() $Q_{\delta '}:=(s-\delta ' r^2, s]\times B^+_{\delta ' r}(x_0)$
. In particular, when Ric
$Q_{\delta '}:=(s-\delta ' r^2, s]\times B^+_{\delta ' r}(x_0)$
. In particular, when Ric
![]() $_N\geq -K$
for some
$_N\geq -K$
for some
![]() $N\in [n, \infty )$
and
$N\in [n, \infty )$
and
![]() $K>0$
, (3.2) holds.
$K>0$
, (3.2) holds.
Proof For the sake of simplicity, we denote
![]() $B_{\bullet }: =B^+_{\bullet }(x_0)$
in the following. Since u is a nonnegative local subsolution to the heat equation in Q, we have
$B_{\bullet }: =B^+_{\bullet }(x_0)$
in the following. Since u is a nonnegative local subsolution to the heat equation in Q, we have
for any
![]() $t\in (s-\delta r^2, s]$
and nonnegative function
$t\in (s-\delta r^2, s]$
and nonnegative function
![]() $\phi \in W^{1, 2}_0(Q)$
. Let
$\phi \in W^{1, 2}_0(Q)$
. Let
![]() $\lambda =\lambda (t)\in C_0^\infty (\mathbb R)$
and
$\lambda =\lambda (t)\in C_0^\infty (\mathbb R)$
and
![]() $\varphi =\varphi (x)\in C_0^\infty (B_r)$
be cutoff functions. Replacing
$\varphi =\varphi (x)\in C_0^\infty (B_r)$
be cutoff functions. Replacing
![]() $\phi $
with
$\phi $
with
![]() $u\varphi ^2\lambda ^2$
in (3.3) and using the Cauchy–Schwarz inequality yield
$u\varphi ^2\lambda ^2$
in (3.3) and using the Cauchy–Schwarz inequality yield
 $$ \begin{align*} &\int_{s-r^2}^{t} \int_{B_r} \lambda^2\varphi^2 F^2(\nabla u)dmdt+\int_{s-r^2}^{t}\int_{B_r} \lambda^2\varphi^2u\partial_tu dm dt \\ & \quad \leq -2\int_{s-r^2}^{t}\int_{B_r}\lambda^2\varphi ud\varphi(\nabla u)dm dt \leq 2\int_{s-r^2}^{t}\int_{B_r}\lambda^2\varphi uF^*(-d\varphi)F(\nabla u)dm dt\nonumber \\ & \quad \leq \frac 13\int_{s-r^2}^{t}\int_{B_r}\lambda^2\varphi^2F^{*2}(du)dmdt+3\int_{s-r^2}^{t}\int_{B_r}\lambda^2 u^2F^{*2}(-d\varphi)dm dt, \end{align*} $$
$$ \begin{align*} &\int_{s-r^2}^{t} \int_{B_r} \lambda^2\varphi^2 F^2(\nabla u)dmdt+\int_{s-r^2}^{t}\int_{B_r} \lambda^2\varphi^2u\partial_tu dm dt \\ & \quad \leq -2\int_{s-r^2}^{t}\int_{B_r}\lambda^2\varphi ud\varphi(\nabla u)dm dt \leq 2\int_{s-r^2}^{t}\int_{B_r}\lambda^2\varphi uF^*(-d\varphi)F(\nabla u)dm dt\nonumber \\ & \quad \leq \frac 13\int_{s-r^2}^{t}\int_{B_r}\lambda^2\varphi^2F^{*2}(du)dmdt+3\int_{s-r^2}^{t}\int_{B_r}\lambda^2 u^2F^{*2}(-d\varphi)dm dt, \end{align*} $$
where we used
![]() $F(\nabla u)=F^*(du)$
. Thus,
$F(\nabla u)=F^*(du)$
. Thus,
 $$ \begin{align*} &\int_{s-r^2}^{t}\int_{B_r}\lambda^2\varphi^2\partial_t(u^2)dm dt+\frac 43\int_{s-r^2}^{ t}\int_{B_r}\lambda^2\varphi^2F^{*2}(du)dm dt\\& \quad \leq 6\int_{s-r^2}^{t}\int_{B_r}\lambda^2u^2F^{*2}(-d\varphi)dm dt. \end{align*} $$
$$ \begin{align*} &\int_{s-r^2}^{t}\int_{B_r}\lambda^2\varphi^2\partial_t(u^2)dm dt+\frac 43\int_{s-r^2}^{ t}\int_{B_r}\lambda^2\varphi^2F^{*2}(du)dm dt\\& \quad \leq 6\int_{s-r^2}^{t}\int_{B_r}\lambda^2u^2F^{*2}(-d\varphi)dm dt. \end{align*} $$
Note that
![]() $F^*(d(\varphi u))\leq uF^*(d\varphi )+\varphi F^*(du)$
. Hence,
$F^*(d(\varphi u))\leq uF^*(d\varphi )+\varphi F^*(du)$
. Hence,
 $$ \begin{align} &\int_{s-r^2}^{t}\int_{B_r} \frac{\partial}{\partial t}(\lambda\varphi u)^2dmdt+\int_{s-r^2}^{t}\int_{B_r}\lambda^2F^{*2}(d(\varphi u))dm dt\nonumber \\& \quad \leq 2\int_{s-r^2}^{t}\int_{B_r}\lambda\lambda'\varphi^2 u^2dmdt+ \int_{s-r^2}^{t}\int_{B_r}\lambda^2\varphi^2\partial_t(u^2)dmdt \nonumber \\& \qquad +\frac 43 \int_{s-r^2}^{t}\int_{B_r}\lambda^2\varphi^2F^{*2}(du) u^2dmdt+ 4 \int_{s-r^2}^{t}\int_{B_r}\lambda^2 F^{*2}(d\varphi)dm dt \nonumber \\& \quad \leq 2\int_{s-r^2}^{t}\int_{B_r}\lambda u^2\left[\lambda'\varphi^2+5\Lambda^2\lambda F^{*2}(d\varphi)\right]dm dt. \end{align} $$
$$ \begin{align} &\int_{s-r^2}^{t}\int_{B_r} \frac{\partial}{\partial t}(\lambda\varphi u)^2dmdt+\int_{s-r^2}^{t}\int_{B_r}\lambda^2F^{*2}(d(\varphi u))dm dt\nonumber \\& \quad \leq 2\int_{s-r^2}^{t}\int_{B_r}\lambda\lambda'\varphi^2 u^2dmdt+ \int_{s-r^2}^{t}\int_{B_r}\lambda^2\varphi^2\partial_t(u^2)dmdt \nonumber \\& \qquad +\frac 43 \int_{s-r^2}^{t}\int_{B_r}\lambda^2\varphi^2F^{*2}(du) u^2dmdt+ 4 \int_{s-r^2}^{t}\int_{B_r}\lambda^2 F^{*2}(d\varphi)dm dt \nonumber \\& \quad \leq 2\int_{s-r^2}^{t}\int_{B_r}\lambda u^2\left[\lambda'\varphi^2+5\Lambda^2\lambda F^{*2}(d\varphi)\right]dm dt. \end{align} $$
Now we choose
![]() $\lambda (t)$
on
$\lambda (t)$
on
![]() $\mathbb R$
with
$\mathbb R$
with
![]() $0\leq \lambda \leq 1$
,
$0\leq \lambda \leq 1$
,
![]() $\lambda =0$
in
$\lambda =0$
in
![]() $(-\infty , s-\delta ' r^2)$
,
$(-\infty , s-\delta ' r^2)$
,
![]() $\lambda =1$
in
$\lambda =1$
in
![]() $(s-\delta r^2, +\infty )$
and
$(s-\delta r^2, +\infty )$
and
![]() $|\lambda '(t)|\leq \frac 1{[(\delta '-\delta )r]^2}$
, and
$|\lambda '(t)|\leq \frac 1{[(\delta '-\delta )r]^2}$
, and
![]() $\varphi =\varphi (x)$
on
$\varphi =\varphi (x)$
on
![]() $B_r$
defined by
$B_r$
defined by
 $$ \begin{align*} \varphi(x):=\left\{\begin{array}{lll} 1, & \mathrm{{on}}\ B_{\delta r}, \\ \frac{\delta'r-d_F(x_0, x)}{(\delta'-\delta)r}, & \mathrm{{on}}\ B_{\delta' r}\kern1pt{\backslash}\kern1pt B_{\delta r},\\ 0, & \mathrm{{on}}\ B_{\delta'r}. \end{array} \right. \end{align*} $$
$$ \begin{align*} \varphi(x):=\left\{\begin{array}{lll} 1, & \mathrm{{on}}\ B_{\delta r}, \\ \frac{\delta'r-d_F(x_0, x)}{(\delta'-\delta)r}, & \mathrm{{on}}\ B_{\delta' r}\kern1pt{\backslash}\kern1pt B_{\delta r},\\ 0, & \mathrm{{on}}\ B_{\delta'r}. \end{array} \right. \end{align*} $$
Then
![]() $F^*( - d\varphi )\leq \frac 1{(\delta '-\delta )r}$
and
$F^*( - d\varphi )\leq \frac 1{(\delta '-\delta )r}$
and
![]() $F^*(d\varphi )\leq \Lambda F^*(-d\varphi )\leq \frac {\Lambda }{(\delta '-\delta )r}$
a.e. on
$F^*(d\varphi )\leq \Lambda F^*(-d\varphi )\leq \frac {\Lambda }{(\delta '-\delta )r}$
a.e. on
![]() $B_{\delta ' r}$
. Denote
$B_{\delta ' r}$
. Denote
![]() $I_\delta : =(s-\delta r^2, s]$
and
$I_\delta : =(s-\delta r^2, s]$
and
![]() $I_{\delta '}: =(s-\delta 'r^2, s]$
. Taking the supremum with respect to t on both sides of (3.5) yields
$I_{\delta '}: =(s-\delta 'r^2, s]$
. Taking the supremum with respect to t on both sides of (3.5) yields
 $$ \begin{align} \sup\limits_{t\in I_\delta} \left\{\int_{B_r}(\varphi u)^2dm \right\}+\int_{I_{\delta}\times B_r} F^{*2}(d(\varphi u))dmdt\leq \frac {12\Lambda^2}{(\delta'-\delta)^2r^2}\int_{Q_{\delta'}} u^2dm dt. \end{align} $$
$$ \begin{align} \sup\limits_{t\in I_\delta} \left\{\int_{B_r}(\varphi u)^2dm \right\}+\int_{I_{\delta}\times B_r} F^{*2}(d(\varphi u))dmdt\leq \frac {12\Lambda^2}{(\delta'-\delta)^2r^2}\int_{Q_{\delta'}} u^2dm dt. \end{align} $$
On the other hand, by Hölder's inequality and Soblev's inequality (3.1), we have
 $$ \begin{align} \int_{B_r}(u\varphi)^{2(1+\frac 2\nu)}dm &\leq \left(\int_{B_r}(u\varphi)^{\frac{2\nu}{\nu-2}}dm\right)^{\frac {\nu-2}{\nu}}\cdot \left(\int_{B_r} (u\varphi)^2dm\right)^{\frac 2\nu} \nonumber \\&\leq \left(A\int_{B_r}[F^{*2}(d(u\varphi))+r^{-2}(u\varphi)^2]dm\right) \cdot \left(\int_{B_r} (u\varphi)^2dm\right)^{\frac 2\nu} \end{align} $$
$$ \begin{align} \int_{B_r}(u\varphi)^{2(1+\frac 2\nu)}dm &\leq \left(\int_{B_r}(u\varphi)^{\frac{2\nu}{\nu-2}}dm\right)^{\frac {\nu-2}{\nu}}\cdot \left(\int_{B_r} (u\varphi)^2dm\right)^{\frac 2\nu} \nonumber \\&\leq \left(A\int_{B_r}[F^{*2}(d(u\varphi))+r^{-2}(u\varphi)^2]dm\right) \cdot \left(\int_{B_r} (u\varphi)^2dm\right)^{\frac 2\nu} \end{align} $$
for some
![]() $\nu>2$
, where
$\nu>2$
, where
![]() $A:=e^{c_0(1+r\sqrt {K})}r^2m(B_r)^{-2/\nu }$
, where
$A:=e^{c_0(1+r\sqrt {K})}r^2m(B_r)^{-2/\nu }$
, where
![]() $c_0=c_0(N, \Lambda )$
is a positive constant. From (3.5) and (3.6), one obtains
$c_0=c_0(N, \Lambda )$
is a positive constant. From (3.5) and (3.6), one obtains
 $$ \begin{align*} \int_{Q_{\delta }} u^{2(1+\frac 2\nu)}dm dt &=\int_{Q_{\delta }} (u\varphi)^{2(1+\frac 2\nu)}dm dt\nonumber \\& \leq \left(A\int_{I_\delta\times B_r}[F^{*2}(d(u\varphi))+r^{-2}(u\varphi)^2]dm dt\right) \left(\sup\limits_{t\in I_{\delta}}\int_{B_r} (u\varphi)^2dm\right)^{2/\nu} \nonumber \\& \leq A\left(\frac {12\Lambda^2}{((\delta'-\delta)r)^2}\int_{Q_{\delta'}} u^2dm dt\right)^{1+\frac 2\nu}. \end{align*} $$
$$ \begin{align*} \int_{Q_{\delta }} u^{2(1+\frac 2\nu)}dm dt &=\int_{Q_{\delta }} (u\varphi)^{2(1+\frac 2\nu)}dm dt\nonumber \\& \leq \left(A\int_{I_\delta\times B_r}[F^{*2}(d(u\varphi))+r^{-2}(u\varphi)^2]dm dt\right) \left(\sup\limits_{t\in I_{\delta}}\int_{B_r} (u\varphi)^2dm\right)^{2/\nu} \nonumber \\& \leq A\left(\frac {12\Lambda^2}{((\delta'-\delta)r)^2}\int_{Q_{\delta'}} u^2dm dt\right)^{1+\frac 2\nu}. \end{align*} $$
For any
![]() $\tau \geq 1$
, it is easy to see that
$\tau \geq 1$
, it is easy to see that
![]() $u^\tau $
is also a nonnegative subsolution of the heat equation. Let
$u^\tau $
is also a nonnegative subsolution of the heat equation. Let
![]() $\chi : =1+\frac 2\nu $
. The above inequality implies that
$\chi : =1+\frac 2\nu $
. The above inequality implies that
 $$ \begin{align} \int_{Q_{\delta }} u^{2\chi\tau}dm dt\leq A\left(\frac {12\Lambda^2}{((\delta'-\delta)r)^2}\int_{Q_{\delta'}} u^{2\tau}dm dt\right)^{\chi}. \end{align} $$
$$ \begin{align} \int_{Q_{\delta }} u^{2\chi\tau}dm dt\leq A\left(\frac {12\Lambda^2}{((\delta'-\delta)r)^2}\int_{Q_{\delta'}} u^{2\tau}dm dt\right)^{\chi}. \end{align} $$
For any
![]() $0<\delta <\delta '\leq 1$
, let
$0<\delta <\delta '\leq 1$
, let
![]() $\delta _0=\delta ', \delta _{i+1}=\delta _i-\frac {\delta '-\delta }{2^{i+1}}$
, and
$\delta _0=\delta ', \delta _{i+1}=\delta _i-\frac {\delta '-\delta }{2^{i+1}}$
, and
![]() $\tau =\chi ^i>1$
on
$\tau =\chi ^i>1$
on
![]() $B_{\delta _ir}$
for
$B_{\delta _ir}$
for
![]() $i=0, 1, \ldots $
. Applying (3.7) for
$i=0, 1, \ldots $
. Applying (3.7) for
![]() $\delta '=\delta _i$
,
$\delta '=\delta _i$
,
![]() $\delta =\delta _{i+1}$
, and
$\delta =\delta _{i+1}$
, and
![]() $\tau =\chi ^i$
, we get
$\tau =\chi ^i$
, we get
 $$ \begin{align*} \int_{Q_{\delta_{i+1}}}u^{2\chi^{i+1}}dm dt\leq A\left(\frac {(12\Lambda^2)4^{i+1}}{((\delta'-\delta)r)^2}\int_{Q_{\delta_i}}u^{2\chi^i}dm dt\right)^\chi. \end{align*} $$
$$ \begin{align*} \int_{Q_{\delta_{i+1}}}u^{2\chi^{i+1}}dm dt\leq A\left(\frac {(12\Lambda^2)4^{i+1}}{((\delta'-\delta)r)^2}\int_{Q_{\delta_i}}u^{2\chi^i}dm dt\right)^\chi. \end{align*} $$
By iteration, one obtains that
 $$ \begin{align} \left(\int_{Q_{\delta_{i+1}}}u^{2\chi^{i+1}}dm dt\right)^{\frac 1{\chi^{i+1}}}\leq C^{\sum j\chi^{1-j}}(A)^{\sum \chi^{-j}}\left[(\delta'-\delta)r\right]^{-2\sum \chi^{1-j}}\cdot \int_{Q_{\delta'}}u^2dm dt, \end{align} $$
$$ \begin{align} \left(\int_{Q_{\delta_{i+1}}}u^{2\chi^{i+1}}dm dt\right)^{\frac 1{\chi^{i+1}}}\leq C^{\sum j\chi^{1-j}}(A)^{\sum \chi^{-j}}\left[(\delta'-\delta)r\right]^{-2\sum \chi^{1-j}}\cdot \int_{Q_{\delta'}}u^2dm dt, \end{align} $$
in which
![]() $\sum $
denotes the summation on j from
$\sum $
denotes the summation on j from
![]() $1$
to
$1$
to
![]() $i+1$
and C is a positive constant depending on
$i+1$
and C is a positive constant depending on
![]() $\Lambda $
. Since
$\Lambda $
. Since
![]() $\sum _{j=1}^\infty \chi ^{1-j}=1+\frac {\nu } 2$
,
$\sum _{j=1}^\infty \chi ^{1-j}=1+\frac {\nu } 2$
,
![]() $\sum _{j=1}^\infty \chi ^{-j}=\frac {\nu } 2$
and
$\sum _{j=1}^\infty \chi ^{-j}=\frac {\nu } 2$
and
![]() $\sum _{j=1}^\infty j\chi ^{1-j}$
converges, (3.8) implies (3.2) by taking
$\sum _{j=1}^\infty j\chi ^{1-j}$
converges, (3.8) implies (3.2) by taking
![]() $i\rightarrow \infty $
. This finishes the proof.
$i\rightarrow \infty $
. This finishes the proof.
Based on Proposition 3.1, we have the following local
![]() $L^p(0<p\leq 2)$
mean value inequality.
$L^p(0<p\leq 2)$
mean value inequality.
Theorem 3.1 Let
![]() $(M, F, m)$
be an n-dimensional forward complete Finsler measure space with finite reversibility
$(M, F, m)$
be an n-dimensional forward complete Finsler measure space with finite reversibility
![]() $\Lambda $
. Fix
$\Lambda $
. Fix
![]() $R>0$
, and assume that (3.1) holds. If u is a nonnegative
$R>0$
, and assume that (3.1) holds. If u is a nonnegative
![]() $L^p$
subsolution of the heat equation in the cylinder
$L^p$
subsolution of the heat equation in the cylinder
![]() $Q=(s-r^2, s+\varepsilon )\times B^+_r(x_0)$
for any
$Q=(s-r^2, s+\varepsilon )\times B^+_r(x_0)$
for any
![]() $s\in \mathbb R$
and
$s\in \mathbb R$
and
![]() $0<r<R$
, where
$0<r<R$
, where
![]() $\varepsilon $
is a positive constant, then there are constants
$\varepsilon $
is a positive constant, then there are constants
![]() $\nu>2$
and
$\nu>2$
and
![]() $c=c(N, p, \nu , \Lambda )>0$
such that
$c=c(N, p, \nu , \Lambda )>0$
such that
 $$ \begin{align} \sup\limits_{Q_{\delta }}u^p\leq \frac{e^{c(1+r\sqrt{K})}}{(\delta'-\delta)^{2+\nu}r^2 m(B_r)}\int_{Q_{\delta'}} u^pdmdt \end{align} $$
$$ \begin{align} \sup\limits_{Q_{\delta }}u^p\leq \frac{e^{c(1+r\sqrt{K})}}{(\delta'-\delta)^{2+\nu}r^2 m(B_r)}\int_{Q_{\delta'}} u^pdmdt \end{align} $$
for any
![]() $0<p\leq 2$
and
$0<p\leq 2$
and
![]() $0<\delta <\delta '\leq 1$
, where
$0<\delta <\delta '\leq 1$
, where
![]() $Q_{\delta }$
and
$Q_{\delta }$
and
![]() $Q_{\delta '}$
are as in Proposition 3.1. In particular, when Ric
$Q_{\delta '}$
are as in Proposition 3.1. In particular, when Ric
![]() $_N\geq -K(K>0)$
for some
$_N\geq -K(K>0)$
for some
![]() $N\in [n, \infty )$
, (3.9) holds.
$N\in [n, \infty )$
, (3.9) holds.
Proof The case when
![]() $p=2$
has been proved in Proposition 3.1. It suffices to prove (3.9) for any
$p=2$
has been proved in Proposition 3.1. It suffices to prove (3.9) for any
![]() $0<p<2$
.
$0<p<2$
.
We denote
![]() $B_{\bullet }:=B_{\bullet }^+(x_0)$
as in the proof of Proposition 3.1. For any
$B_{\bullet }:=B_{\bullet }^+(x_0)$
as in the proof of Proposition 3.1. For any
![]() $0<\delta <\delta"\leq\delta'\leq 1$
, it follows from Proposition 3.1 that there are positive constants
$0<\delta <\delta"\leq\delta'\leq 1$
, it follows from Proposition 3.1 that there are positive constants
![]() $\nu =\nu (N)>2$
and
$\nu =\nu (N)>2$
and
![]() $c=c(N, \nu , \Lambda )$
such that
$c=c(N, \nu , \Lambda )$
such that
 $$\begin{align*} \sup\limits_{Q_{\delta }}u^2 &\leq \frac{e^{c(1+r\sqrt{K})}}{ (\delta"-\delta)^{2+\nu}r^2m(B_r)}\int_{Q_{\delta"}}u^2dmdt.\end{align*}$$
$$\begin{align*} \sup\limits_{Q_{\delta }}u^2 &\leq \frac{e^{c(1+r\sqrt{K})}}{ (\delta"-\delta)^{2+\nu}r^2m(B_r)}\int_{Q_{\delta"}}u^2dmdt.\end{align*}$$
On the other hand,
 $$\begin{align*} \int_{Q_{\delta"}}u^2dmdt\leq\sup_{Q_{\delta"}}u^{2-p}\int_{Q_{\delta"}}u^pdmdt\leq \left(\sup_{Q_{\delta"}}u^2\right)^{1-p/2}\int_{Q_{\delta'}}u^pdmdt\end{align*}$$
$$\begin{align*} \int_{Q_{\delta"}}u^2dmdt\leq\sup_{Q_{\delta"}}u^{2-p}\int_{Q_{\delta"}}u^pdmdt\leq \left(\sup_{Q_{\delta"}}u^2\right)^{1-p/2}\int_{Q_{\delta'}}u^pdmdt\end{align*}$$
Thus,
 $$\begin{align} \sup\limits_{Q_{\delta }}u^2\leq \frac{e^{ c(1+r\sqrt{K})}}{(\delta"-\delta)^{2+\nu}r^2m(B_r)}\left(\sup\limits_{Q_{\delta"}}u^2\right)^{1-p/2}\int_{Q_{\delta'}}u^pdmdt.\end{align}$$
$$\begin{align} \sup\limits_{Q_{\delta }}u^2\leq \frac{e^{ c(1+r\sqrt{K})}}{(\delta"-\delta)^{2+\nu}r^2m(B_r)}\left(\sup\limits_{Q_{\delta"}}u^2\right)^{1-p/2}\int_{Q_{\delta'}}u^pdmdt.\end{align}$$
Let
![]() $\mu =1-p/2>0$
and
$\mu =1-p/2>0$
and
 $$ \begin{align*}\mathcal M(\delta):=\sup\limits_{Q_{\delta}}u^2, \ \ \ \ \ \ \mathcal R=\frac{e^{ c(1+r\sqrt{K})}}{r^{2}m(B_{r})}\int_{Q_{\delta'}}u^pdmdt.\end{align*} $$
$$ \begin{align*}\mathcal M(\delta):=\sup\limits_{Q_{\delta}}u^2, \ \ \ \ \ \ \mathcal R=\frac{e^{ c(1+r\sqrt{K})}}{r^{2}m(B_{r})}\int_{Q_{\delta'}}u^pdmdt.\end{align*} $$
Let
![]() $\delta _0=\delta $
and
$\delta _0=\delta $
and
![]() $\delta _{i}=\delta _{i-1}+\frac {\delta '-\delta }{2^i}$
for
$\delta _{i}=\delta _{i-1}+\frac {\delta '-\delta }{2^i}$
for
![]() $i= 1, 2, \ldots $
. Applying (3.10) for
$i= 1, 2, \ldots $
. Applying (3.10) for
![]() $\delta =\delta _{i-1}$
and
$\delta =\delta _{i-1}$
and
![]() $\delta"=\delta_i$
yields
$\delta"=\delta_i$
yields
By iterating, we get
in which
![]() $\sum $
denotes the summation on i from
$\sum $
denotes the summation on i from
![]() $1$
to j. Obviously,
$1$
to j. Obviously,
![]() $\lim \limits _{j\rightarrow \infty }\delta _j=\delta '$
and
$\lim \limits _{j\rightarrow \infty }\delta _j=\delta '$
and
![]() $\lim \limits _{j\rightarrow \infty }\mu ^j=0$
. Moreover,
$\lim \limits _{j\rightarrow \infty }\mu ^j=0$
. Moreover,
![]() $\sum _{i=1}^\infty \mu ^{i-1}=2/p$
and
$\sum _{i=1}^\infty \mu ^{i-1}=2/p$
and
![]() $\sum _{i=1}^\infty i\mu ^{i-1}$
converges. Letting
$\sum _{i=1}^\infty i\mu ^{i-1}$
converges. Letting
![]() $j\rightarrow \infty $
on both sides of (3.11), there is a positive constant
$j\rightarrow \infty $
on both sides of (3.11), there is a positive constant
![]() $c=c(N, \nu , p)$
such that
$c=c(N, \nu , p)$
such that
The proof is finished.
4 Proofs of Theorems 1.1 and 1.2
Proof of Theorem 1.1
Let
![]() $B_R: =B^+_R(x_0)$
, and let
$B_R: =B^+_R(x_0)$
, and let
![]() $\psi (x)$
be a cutoff function defined by
$\psi (x)$
be a cutoff function defined by
 $$ \begin{align*} \psi(x)=\left\{\begin{array}{lll} 1, & \mbox{on}\ B_R, \\ \frac{2R-d_F(x_0, x)}R, & \mbox{on} \ B_{2R}\kern1pt{\backslash}\kern1pt B_R, \\ 0, & \mbox{on} \ M\kern1pt{\backslash}\kern1pt B_{2R}. \end{array} \right. \end{align*} $$
$$ \begin{align*} \psi(x)=\left\{\begin{array}{lll} 1, & \mbox{on}\ B_R, \\ \frac{2R-d_F(x_0, x)}R, & \mbox{on} \ B_{2R}\kern1pt{\backslash}\kern1pt B_R, \\ 0, & \mbox{on} \ M\kern1pt{\backslash}\kern1pt B_{2R}. \end{array} \right. \end{align*} $$
Obviously,
![]() $0\leq \psi \leq 1$
and
$0\leq \psi \leq 1$
and
![]() $F^*(-d\psi )\leq 1/R$
a.e., on
$F^*(-d\psi )\leq 1/R$
a.e., on
![]() $B_{2R}$
. Since
$B_{2R}$
. Since
![]() $\lim \limits _{t\rightarrow 0}\int _Mu^p(t, x)dm=0$
, we have
$\lim \limits _{t\rightarrow 0}\int _Mu^p(t, x)dm=0$
, we have
which means that
![]() $\lim \limits _{t\rightarrow 0}\int _M\psi ^2u^pdm=0$
. Choosing
$\lim \limits _{t\rightarrow 0}\int _M\psi ^2u^pdm=0$
. Choosing
![]() $\phi :=\psi ^2 u^{p-1}$
as a test function in (1.2) yields
$\phi :=\psi ^2 u^{p-1}$
as a test function in (1.2) yields
 $$ \begin{align*} -\int_0^T\int_Md(\psi^2u^{p-1})(\nabla u)dm dt &\geq \int_0^T\int_M \psi^2u^{p-1}\frac{\partial u}{\partial t}dm dt\\&\geq \frac 1p\int_0^T\frac{\partial}{\partial t}\left(\int_M\psi^2u^pdm\right)dt \\ &=\frac 1p\int_M\psi^2u^p(T, x)dm \end{align*} $$
$$ \begin{align*} -\int_0^T\int_Md(\psi^2u^{p-1})(\nabla u)dm dt &\geq \int_0^T\int_M \psi^2u^{p-1}\frac{\partial u}{\partial t}dm dt\\&\geq \frac 1p\int_0^T\frac{\partial}{\partial t}\left(\int_M\psi^2u^pdm\right)dt \\ &=\frac 1p\int_M\psi^2u^p(T, x)dm \end{align*} $$
by the assumption. On the other hand, the LHS of the above inequality is equal to
 $$ \begin{align*} & -2\int_0^T\int_M \psi u^{p-1}d\psi(\nabla u)dm dt-(p-1)\int_0^T\int_M\psi^2u^{p-2}F^2(\nabla u)dmdt \\ &\leq \frac {2}{p-1}\int_0^T\int_M u^pF^{*2}(-d\psi)dm dt-\frac{2(p-1)}{p^2}\int_0^T\int_M\psi^2 F^2(\nabla u^{p/2})dmdt, \end{align*} $$
$$ \begin{align*} & -2\int_0^T\int_M \psi u^{p-1}d\psi(\nabla u)dm dt-(p-1)\int_0^T\int_M\psi^2u^{p-2}F^2(\nabla u)dmdt \\ &\leq \frac {2}{p-1}\int_0^T\int_M u^pF^{*2}(-d\psi)dm dt-\frac{2(p-1)}{p^2}\int_0^T\int_M\psi^2 F^2(\nabla u^{p/2})dmdt, \end{align*} $$
where we used the inequality
![]() $-d\psi (\nabla u)\leq F^*(-d\psi )F(\nabla u)$
. Therefore,
$-d\psi (\nabla u)\leq F^*(-d\psi )F(\nabla u)$
. Therefore,
 $$ \begin{align*} \int_M\psi^2u^p(T, x)dm+\frac{2(p-1)}{p}\int_0^T\int_M\psi^2 F^2(\nabla u^{p/2})dm dt\leq \frac{2p}{(p-1)R^2}\int_0^T\int_Mu^pdm dt. \end{align*} $$
$$ \begin{align*} \int_M\psi^2u^p(T, x)dm+\frac{2(p-1)}{p}\int_0^T\int_M\psi^2 F^2(\nabla u^{p/2})dm dt\leq \frac{2p}{(p-1)R^2}\int_0^T\int_Mu^pdm dt. \end{align*} $$
Letting
![]() $R\rightarrow \infty $
, one obtains
$R\rightarrow \infty $
, one obtains
which implies that
![]() $u(T, x)=0$
and
$u(T, x)=0$
and
![]() $\nabla u^{p/2}=0$
. Hence,
$\nabla u^{p/2}=0$
. Hence,
![]() $u(t, x)\equiv 0$
.
$u(t, x)\equiv 0$
.
Proof of Theorem 1.2
By Theorem 3.1 and the assumption, we have
 $$ \begin{align} u(t, x_0)\leq \sup\limits_{Q_{\delta}}u^p &\leq \frac{c}{(\delta'-\delta)^{2+\nu}r^2 m(B_r)}\int_{Q_{\delta'}} u^pdmdt \nonumber \\&=\frac{c}{(\delta'-\delta)^{2+\nu}r^2 m(B_r)}\int_{\mathbb R\times M} u^p dm dt \end{align} $$
$$ \begin{align} u(t, x_0)\leq \sup\limits_{Q_{\delta}}u^p &\leq \frac{c}{(\delta'-\delta)^{2+\nu}r^2 m(B_r)}\int_{Q_{\delta'}} u^pdmdt \nonumber \\&=\frac{c}{(\delta'-\delta)^{2+\nu}r^2 m(B_r)}\int_{\mathbb R\times M} u^p dm dt \end{align} $$
for any
![]() $t\in (s-\delta r^2, s]$
and
$t\in (s-\delta r^2, s]$
and
![]() $0<\delta <\delta '\leq 1$
. Since Ric
$0<\delta <\delta '\leq 1$
. Since Ric
![]() $_N\geq 0$
and
$_N\geq 0$
and
![]() $\Lambda <\infty $
,
$\Lambda <\infty $
,
![]() $m(B_r)\geq Cr$
by Theorem 5.4 in [Reference WuWu], where C is a positive constant depending on N and
$m(B_r)\geq Cr$
by Theorem 5.4 in [Reference WuWu], where C is a positive constant depending on N and
![]() $\Lambda $
. Note that
$\Lambda $
. Note that
![]() $0\leq u(t,x)\in L^p(\mathbb R\times M)$
by the assumption. Letting
$0\leq u(t,x)\in L^p(\mathbb R\times M)$
by the assumption. Letting
![]() $\delta \rightarrow 0$
and then
$\delta \rightarrow 0$
and then
![]() $r\rightarrow \infty $
on both sides of (4.1) yields
$r\rightarrow \infty $
on both sides of (4.1) yields
![]() $u(s, x_0)\equiv 0$
. Since
$u(s, x_0)\equiv 0$
. Since
![]() $s\in \mathbb R$
and
$s\in \mathbb R$
and
![]() $x_0\in M$
are arbitrary, we have
$x_0\in M$
are arbitrary, we have
![]() $u(t, x)\equiv 0$
on
$u(t, x)\equiv 0$
on
![]() $\mathbb R\times M$
.
$\mathbb R\times M$
.