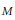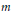Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Li, Ming
and
Zhou, JiaZu
2010.
An isoperimetric deficit upper bound of the convex domain in a surface of constant curvature.
Science China Mathematics,
Vol. 53,
Issue. 8,
p.
1941.
Jiazu, Zhou
2011.
On the Willmore's Theorem for convex hypersurfaces.
Acta Mathematica Scientia,
Vol. 31,
Issue. 2,
p.
361.
Zhou, Jiazu
Xia, Yunwei
and
Zeng, Chunna
2011.
SOME NEW BONNESEN-STYLE INEQUALITIES.
Journal of the Korean Mathematical Society,
Vol. 48,
Issue. 2,
p.
421.
Zhou, Jia Zu
Du, Yan Hua
and
Cheng, Fei
2012.
Some Bonnesen-style inequalities for higher dimensions.
Acta Mathematica Sinica, English Series,
Vol. 28,
Issue. 12,
p.
2561.
Xia, YunWei
Xu, WenXue
Zhou, JiaZu
and
Zhu, BaoCheng
2013.
Reverse Bonnesen style inequalities in a surface $$\mathbb{X}_\varepsilon ^2$$ of constant curvature.
Science China Mathematics,
Vol. 56,
Issue. 6,
p.
1145.
Xu, Wenxue
Zhou, Jiazu
and
Zhu, Baocheng
2013.
On containment measure and the mixed isoperimetric inequality.
Journal of Inequalities and Applications,
Vol. 2013,
Issue. 1,
Jiang, De Shuo
Zhou, Jia Zu
and
Chen, Fang Wei
2014.
A kinematic formula for integral invariant of degree 4 in real space form.
Acta Mathematica Sinica, English Series,
Vol. 30,
Issue. 8,
p.
1465.
Li, Ming
and
Zhou, Jiazu
2016.
Kinematic formulas of total mean curvatures for hypersurfaces.
Chinese Annals of Mathematics, Series B,
Vol. 37,
Issue. 1,
p.
137.
Zhu, Baocheng
and
Xu, Wenxue
2018.
Reverse Bonnesen-style inequalities on surfaces of constant curvature.
International Journal of Mathematics,
Vol. 29,
Issue. 06,
p.
1850040.