No CrossRef data available.
Article contents
On semidirectly closed pseudovarieties of finite semigroups and monoids
Published online by Cambridge University Press: 02 August 2021
Abstract
For every pseudovariety 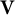 $\mathbf {V}$
of finite monoids, let
$\mathbf {V}$
of finite monoids, let 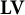 $\mathbf {LV}$
denote the pseudovariety of all finite semigroups all of whose local submonoids belong to
$\mathbf {LV}$
denote the pseudovariety of all finite semigroups all of whose local submonoids belong to  $\mathbf {V}$
. In this paper, it is shown that, for every nontrivial semidirectly closed pseudovariety
$\mathbf {V}$
. In this paper, it is shown that, for every nontrivial semidirectly closed pseudovariety  $\mathbf {V}$
of finite monoids, the pseudovariety
$\mathbf {V}$
of finite monoids, the pseudovariety 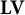 $\mathbf {LV}$
of finite semigroups is also semidirectly closed if, and only if, the given pseudovariety
$\mathbf {LV}$
of finite semigroups is also semidirectly closed if, and only if, the given pseudovariety 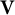 $\mathbf {V}$
is local in the sense of Tilson. This finding resolves a long-standing open problem posed in the second volume of the classic monograph by Eilenberg.
$\mathbf {V}$
is local in the sense of Tilson. This finding resolves a long-standing open problem posed in the second volume of the classic monograph by Eilenberg.
Keywords
MSC classification
- Type
- Article
- Information
- Copyright
- © Canadian Mathematical Society 2021
Footnotes
This research has been supported by the Grant Agency of the Czech Republic under the project GA19-12790S.



