Hostname: page-component-cd9895bd7-dzt6s Total loading time: 0 Render date: 2024-12-27T12:22:08.619Z Has data issue: false hasContentIssue false
  • English
  • Français

On metabelian 2-class field towers over 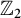$\mathbb Z_2$-extensions of real quadratic fields

Part of: Algebraic number theory: global fields

Published online by Cambridge University Press:  06 October 2021

Yasushi Mizusawa Open the ORCID record for Yasushi Mizusawa [Opens in a new window]
Yasushi Mizusawa*
Affiliation:
Department of Mathematics, Nagoya Institute of Technology, Gokiso-cho, Showa-ku, Nagoya, 466-8555, Japan
*
e-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

We give a family of real quadratic fields such that the 2-class field towers over their cyclotomic 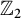$\mathbb Z_2$ -extensions have metabelian Galois groups of abelian invariants 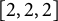$[2,2,2]$ . We also consider the boundedness of the Galois groups in relation to Greenberg’s conjecture, and calculate their class-2 quotients with an explicit example.

Keywords

p-class field towerℤp-extensionGreenberg’s conjecture

MSC classification

Primary: 11R23: Iwasawa theory
Secondary: 11R11: Quadratic extensions 11R32: Galois theory 11R37: Class field theory
Type
Article
Information
Canadian Mathematical Bulletin , Volume 65 , Issue 3 , September 2022 , pp. 795 - 805
Check for updates
Copyright
© Canadian Mathematical Society 2021

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Azizi, A. and Mouhib, A., Sur le rang du 2-groupe de classes de $Q(\sqrt{m},\sqrt{d})$ où $\ m= 2\ {}$ ou un premier $p \equiv 1\ (mod\ 4)$ . Trans. Amer. Math. Soc. 353(2001), no. 7, 2741–2752.CrossRefGoogle Scholar
Azizi, A., Rezzougui, M., and Zekhnini, A., On the maximal unramified pro- $2$ -extension of certain cyclotomic $~{\mathbb{Z}}_2$ -extensions . Period. Math. Hungar. 83(2021), no. 1, 54–66.CrossRefGoogle Scholar
Benjamin, E., Lemmermeyer, F., and Snyder, C., Real quadratic fields with abelian $2$ -class field tower . J. Number Theory 73(1998), no. 2, 182–194.CrossRefGoogle Scholar
Benjamin, E. and Snyder, C., Real quadratic number fields with $2$ -class group of type $\left(2,2\right)$ . Math. Scand. 76(1995), no. 2, 161–178.CrossRefGoogle Scholar
Fukuda, T., Remarks on $\textbf{Z}_p$ -extensions of number fields . Proc. Japan Acad. Ser. A 70(1994), 264–266.CrossRefGoogle Scholar
Fukuda, T. and Komatsu, K., On the Iwasawa $\lambda$ -invariant of the cyclotomic $\textbf{Z}_2$ -extension of a real quadratic field . Tokyo J. Math. 28(2005), no. 1, 259–264.CrossRefGoogle Scholar
Gamble, G., Nickel, W., O’Brien, E. and Horn, M., ANUPQ, ANU p-Quotient – a GAP package, Version 3.2.1. 2019. https://gap-packages.github.io/anupq/ Google Scholar
The GAP Group, GAP – Groups, Algorithms, and Programming, Version 4.11.1. 2021. https://www.gap-system.org Google Scholar
Greenberg, R., On the Iwasawa invariants of totally real number fields . Amer. J. Math. 98(1976), no. 1, 263–284.CrossRefGoogle Scholar
Iwasawa, K., A note on class numbers of algebraic number fields . Abh. Math. Sem. Univ. Hamburg 20(1956), 257–258.CrossRefGoogle Scholar
Kisilevsky, H., Number fields with class number congruent to 4 mod 8 and Hilbert’s theorem 94 . J. Number Theory 8(1976), no. 3, 271–279.CrossRefGoogle Scholar
Kumakawa, N., On the Iwasawa $\lambda$ -invariant of the cyclotomic $~{\mathbb{Z}}_2$ -extension of $\,\mathbb{Q}(\sqrt{pq})$ and the $2$ -part of the class number of $\,\mathbb{Q}(\sqrt{pq},\sqrt{2+\sqrt{2}})$ . Int. J. Number Theory 17(2021), no. 4, 931–958.CrossRefGoogle Scholar
Lemmermeyer, F., The ambiguous class number formula revisited . J. Ramanujan Math. Soc. 28(2013), no. 4, 415–421.Google Scholar
Mizusawa, Y., On the maximal unramified pro- $2$ -extension of $~{\mathbb{Z}}_2$ -extensions of certain real quadratic fields II . Acta Arith. 119(2005), no. 1, 93–107.CrossRefGoogle Scholar
Mizusawa, Y., On unramified Galois $2$ -groups over ${\mathbb{Z}}_2$ -extensions of real quadratic fields . Proc. Amer. Math. Soc. 138(2010), no. 9, 3095–3103.CrossRefGoogle Scholar
Mizusawa, Y., A note on semidihedral $2$ -class field towers and ${\mathbb{Z}}_2$ -extensions . Ann. Math. Qué. 38(2014), no. 1, 73–79.CrossRefGoogle Scholar
Mizusawa, Y., Tame pro- $2$ Galois groups and the basic ${\mathbb{Z}}_2$ -extension . Trans. Amer. Math. Soc. 370(2018), no. 4, 2423–2461.CrossRefGoogle Scholar
Mouhib, A., Sur la $2$ -extension maximale non ramifié de la $\textbf{Z}_2$ -extension cyclotomique de certains corps quadratiques . An. Şt. Univ. Ovidius Constanţa 22(2014), no. 1, 207–214.Google Scholar
Mouhib, A. and Movahhedi, A., On the $p$ -class tower of a $\textbf{Z}_p$ -extension . Tokyo J. Math. 31(2008), no. 2, 321–332.CrossRefGoogle Scholar
Nishino, Y., On the Iwasawa invariants of the cyclotomic $\textbf{Z}_2$ -extensions of certain real quadratic fields . Tokyo J. Math. 29(2006), no. 1, 239–245.CrossRefGoogle Scholar
Ozaki, M., Non-abelian Iwasawa theory of ${\mathbb{Z}}_p$ -extensions . J. Reine Angew. Math. 602(2007), 59–94.Google Scholar
Ozaki, M. and Taya, H., On the Iwasawa ${\lambda}_2$ -invariants of certain families of real quadratic fields . Manuscripta Math. 94(1997), no. 4, 437–444.CrossRefGoogle Scholar
The PARI Group, PARI/GP version 2.13.1, Univ. Bordeaux. 2021. http://pari.math.u-bordeaux.fr/ Google Scholar
Salle, L., Sur les pro- $p$ -extensions à ramification restreinte au-dessus de la ${\mathbb{Z}}_p$ -extension cyclotomique d’un corps de nombres . J. Théor. Nombres Bordeaux 20(2008), no. 2, 485–523.CrossRefGoogle Scholar