Hostname: page-component-cd9895bd7-dk4vv Total loading time: 0 Render date: 2024-12-26T20:14:53.395Z Has data issue: false hasContentIssue false
  • English
  • Français

On Classification of Certain C*-Algebras

Published online by Cambridge University Press:  20 November 2018

George Elliott  and
Igor Fulman
George Elliott
Affiliation:
Department of Mathematics, University of Toronto, Toronto, Ontario, M5S 3G3, email: [email protected]
Igor Fulman
Affiliation:
Department of Mathematics and Statistics, University of Calgary 2500 University, Drive NW Calgary, Alberta T2N 1N4, email: [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

We consider 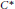${{C}^{*}}$ -algebras which are inductive limits of finite direct sums of copies of 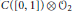$C([0,\,1])\otimes {{\mathcal{O}}_{2}}$ . For such algebras, the lattice of closed two-sided ideals is proved to be a complete invariant.

Keywords

46L0546L35
Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 43 , Issue 3 , 01 September 2000 , pp. 320 - 329
Copyright
Copyright © Canadian Mathematical Society 2000

References

[1] Dixmier, J., Les C*-Algèbres et leurs Représentations. Gauthier-Villars, Paris, 1964.Google Scholar
[2] Elliott, G. A., On the classification of C*-algebras of real rank zero. J.ReineAngew.Math. 443 (1993), 179–219.Google Scholar
[3] Mortensen, J., Classification of certain non-simple C*-algebras. Preprints of Odense Universitet, Denmark, 9, 1996.Google Scholar