Hostname: page-component-cd9895bd7-dk4vv Total loading time: 0 Render date: 2024-12-29T09:27:27.179Z Has data issue: false hasContentIssue false
  • English
  • Français

Norm One Idempotent cb-Multipliers with Applications to the Fourier Algebra in the cb-Multiplier Norm

Published online by Cambridge University Press:  20 November 2018

Brian E. Forrest  and
Volker Runde
Brian E. Forrest
Affiliation:
Department of Pure Mathematics, University of Waterloo, Waterloo, ON, N2L 3G1e-mail: [email protected]
Volker Runde
Affiliation:
Department of Mathematical and Statistical Sciences, University of Alberta, Edmonton, AB, T6G 2G1e-mail: [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

For a locally compact group $G$, let 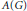$A(G)$ be its Fourier algebra, let ${{M}_{cb}}A(G)$ denote the completely bounded multipliers of 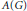$A(G)$, and let 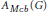${{A}_{Mcb}}\,(G)$ stand for the closure of 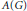$A(G)$ in ${{M}_{cb}}A(G)$. We characterize the norm one idempotents in ${{M}_{cb}}A(G)$: the indicator function of a set 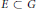$E\,\subset \,G$ is a norm one idempotent in ${{M}_{cb}}A(G)$ if and only if $E$ is a coset of an open subgroup of $G$. As applications, we describe the closed ideals of 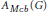${{A}_{Mcb}}\,(G)$ with an approximate identity bounded by 1, and we characterize those $G$ for which 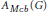${{A}_{Mcb}}\,(G)$ is 1-amenable in the sense of B. E. Johnson. (We can even slightly relax the norm bounds.)

Keywords

43A2220E0543A3046J1046J4046L0747L25amenabilitybounded approximate identitycb-multiplier normFourier algebranorm one idempotent
Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 54 , Issue 4 , 01 December 2011 , pp. 654 - 662
Copyright
Copyright © Canadian Mathematical Society 2011

References

[1] Bożejko, M., Remark on Herz-Schur multipliers on free groups. Math. Ann. 258(1981/82), no. 1, 11–15. doi:10.1007/BF01450343Google Scholar
[2] Bożejko, M. and Fendler, G., Herz–Schur multipliers and completely bounded multipliers of the Fourier algebra of a locally compact group. Boll. Un. Mat. Ital. A (6) 3(1984), no. 2, 297–302.Google Scholar
[3] Brannan, M., Forrest, B. E., and Zwarich, C., Multipliers and complemented ideals in the Fourier algebra. Preprint.Google Scholar
[4] Cohen, P. J., On a conjecture of Littlewood and idempotent measures.. Amer. J. Math. 82(1960), 191–212. doi:10.2307/2372731Google Scholar
[5] Cowling, M. and Haagerup, U., Completely bounded multipliers of the Fourier algebra of a simple Lie group of real rank one. Invent. Math. 96(1989), no. 3, 507–549. doi:10.1007/BF01393695Google Scholar
[6] de Cannière, J. and Haagerup, U., Multipliers of the Fourier algebras of some simple Lie groups and their discrete subgroups. Amer. J. Math. 107(1985), no. 2, 455–500. doi:10.2307/2374423Google Scholar
[7] Effros, E. G. and Ruan, Z.-J., Operator Spaces. London Mathematical Society Monographs 23, The Clarendon Press, New York, 2000.Google Scholar
[8] Eymard, P., L’algèbre de Fourier d’un groupe localement compact. Bull. Soc. Math. France 92(1964), 181–236.Google Scholar
[9] Forrest, B. E., Amenability and bounded approximate identities in ideals of A(G). Illinois J. Math. 34(1990), no. 1, 1–25.Google Scholar
[10] Forrest, B. E., Completely bounded multipliers and ideals in A(G) vanishing on closed subgroups. In: Banach Algebras and Their Applications. Contemp. Math. American Mathematical Society, Providence, RI, 2004, pp. 89–94.Google Scholar
[11] Forrest, B. E. and Wood, P. J., Cohomology and the operator space structure of the Fourier algebra and its second dual. Indiana Univ. Math. J. 50(2001), no. 3, 1217–1240.Google Scholar
[12] Forrest, B. E., Kaniuth, E., Lau, A. T.-M., and Spronk, N., Ideals with bounded approximate identities in Fourier algebras. J. Funct. Anal. 203(2003), no. 1, 286–304. doi:10.1016/S0022-1236(02)00121-0Google Scholar
[13] Forrest, B. E. and Runde, V., Amenability and weak amenability of the Fourier algebra. Math. Z. 250(2005), no. 4, 731–744. doi:10.1007/s00209-005-0772-2Google Scholar
[14] Forrest, B. E., Runde, V., and Spronk, N., Nico Operator amenability of the Fourier algebra in the cb-multiplier norm. Canad. J. Math. 59(2007), no. 5, 966–980. doi:10.4153/CJM-2007-041-9Google Scholar
[15] Gilbert, J. E., Lp-convolution opeators and tensor products of Banach spaces, I, II, and III. Unpublished manuscripts.Google Scholar
[16] Host, B., Le théorème des idempotents dans B(G) . Bull. Soc. Math. France 114(1986), no. 2, 215–223.Google Scholar
[17] Ilie, M. and Spronk, N., Completely bounded homomorphisms of the Fourier algebras. J. Funct. Anal. 225(2005), no. 2, 480–499. doi:10.1016/j.jfa.2004.11.011Google Scholar
[18] Johnson, B. E., Cohomology in Banach Algebras. Memoirs of the American Mathematical Society 127, American Mathematical Society, Providence, RI, 1972.Google Scholar
[19] Johnson, B. E., Approximate diagonals and cohomology of certain annihilator Banach algebras.. Amer. J. Math. 94(1972), 685–698. doi:10.2307/2373751Google Scholar
[20] Johnson, B. E., Non-amenability of the Fourier algebra of a compact group. J. London Math. Soc. 50(1994), no. 2, 361–374.Google Scholar
[21] Jolissaint, P., A characterization of completely bounded multipliers of Fourier algebras. Colloq. Math. 63(1992), no. 2, 311–313.Google Scholar
[22] Katavolos, A. and Paulsen, V. I., On the ranges of bimodule projections. Canad. Math. Bull. 48(2005), no. 1, 97–111. doi:10.4153/CMB-2005-009-4Google Scholar
[23] Leinert, M., Abschätzung von Normen gewisser Matrizen und eine Anwendung. Math. Ann. 240(1979), no. 1, 13–19. doi:10.1007/BF01428295Google Scholar
[24] Leptin, H., Sur l’algèbre de Fourier d’un groupe localement compact. C. R. Acad. Sci. Paris Sér. A-B 266(1968), A1180–A1182.Google Scholar
[25] Livshits, L., A note on 0–1 Schur multipliers. Linear Algebra Appl. 222(1995), 15–22. doi:10.1016/0024-3795(93)00268-5Google Scholar
[26] Losert, V., Properties of the Fourier algebra that are equivalent to amenability. Proc. Amer. Math. Soc. 92(1984), no. 3, 347–354.Google Scholar
[27] Pisier, G., Similarity Problems and Completely Bounded Maps. Lecture Notes in Mathematics 1618, Springer-Verlag, Berlin, 1996.Google Scholar
[28] Ruan, Z.-J., The operator amenability of A(G) . Amer. J. Math. 117(1995), no. 6, 1449–1474. doi:10.2307/2375026Google Scholar
[29] Runde, V., Lectures on Amenability. Lecture Notes in Mathematics 1774, Springer-Verlag, Berlin, 2002.Google Scholar
[30] Runde, V., The amenability constant of the Fourier algebra. Proc. Amer. Math. Soc. 134(2006), no. 5, 1473–1481. doi:10.1090/S0002-9939-05-08164-5Google Scholar
[31] Spronk, N., Measurable Schur multipliers and completely bounded multipliers of the Fourier algebras.. Proc. London Math. Soc. 89(2004), 161–192. doi:10.1112/S0024611504014650Google Scholar