Article contents
Multivariate Rankin–Selberg Integrals on GL4 and GU(2, 2)
Published online by Cambridge University Press: 20 November 2018
Abstract
Inspired by a construction by Bump, Friedberg, and Ginzburg of a two-variable integral representation on 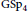 $\text{GS}{{\text{p}}_{4}}$ for the product of the standard and spin
$\text{GS}{{\text{p}}_{4}}$ for the product of the standard and spin  $L$-functions, we give two similar multivariate integral representations. The first is a three-variable Rankin-Selberg integral for cusp forms on
$L$-functions, we give two similar multivariate integral representations. The first is a three-variable Rankin-Selberg integral for cusp forms on  $\text{PG}{{\text{L}}_{4}}$ representing the product of the
$\text{PG}{{\text{L}}_{4}}$ representing the product of the  $L$-functions attached to the three fundamental representations of the Langlands
$L$-functions attached to the three fundamental representations of the Langlands  $L$-group
$L$-group 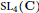 $\text{S}{{\text{L}}_{\text{4}}}\left( \text{C} \right)$. The second integral, which is closely related, is a two-variable Rankin-Selberg integral for cusp forms on
$\text{S}{{\text{L}}_{\text{4}}}\left( \text{C} \right)$. The second integral, which is closely related, is a two-variable Rankin-Selberg integral for cusp forms on 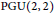 $\text{PGU}\left( 2,\,2 \right)$ representing the product of the degree
$\text{PGU}\left( 2,\,2 \right)$ representing the product of the degree  $8$ standard
$8$ standard  $L$-function and the degree
$L$-function and the degree  $6$ exterior square
$6$ exterior square  $L$-function.
$L$-function.
Keywords
- Type
- Research Article
- Information
- Copyright
- Copyright © Canadian Mathematical Society 2018
References
- 2
- Cited by


