Hostname: page-component-cd9895bd7-8ctnn Total loading time: 0 Render date: 2024-12-27T07:38:43.789Z Has data issue: false hasContentIssue false
  • English
  • Français

Large Sets not Containing Images of a Given Sequence

Published online by Cambridge University Press:  20 November 2018

Péter Komjáth
Péter Komjáth*
Affiliation:
R. Eötvös UniversityDepartment of Algebra and Number Theory Budapest 8, P.O.B. 323 H-1445 , Hungary
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

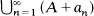In the first part we construct a subset H of positive measure in the unit interval and a zero-sequence {an} so that H contains no homothetic copy of {an}. In Theorem 2 we prove that if ε > 0 and a zero-sequence {an} are given then there exists a set A of measure less than ε so that covers the interval. An application of this result is Theorem 3: for any sequence {an} and ε > 0 there is a set H of measure 1 - ε such that for no N and c is {an + c}n ≥ N contained by H.

Keywords

primary: 28A05secondary: 54G2051M25real sequencesmeasurable sets
Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 26 , Issue 1 , 01 March 1983 , pp. 41 - 43
Copyright
Copyright © Canadian Mathematical Society 1983

References

1. Borwein, D. and S. Z. Ditor, Translates of sequences in sets of positive measure, Canadian Mathematical Bulletin 21/4 (1978), 497-498.Google Scholar
2. Erdős, P., Set theoretic, measure theoretic, combinatorial, and number theoretic problems concerning point sets in Euclidean space, Real Analysis Exchange 4 (1978-79), 113-138.Google Scholar