Article contents
Jordan *-Derivations of Finite-DimensionalSemiprime Algebras
Published online by Cambridge University Press: 20 November 2018
Abstract
In this paper, we characterize Jordan  $*$-derivations of a 2-torsion free, finite-dimensional semiprime algebra
$*$-derivations of a 2-torsion free, finite-dimensional semiprime algebra  $R$ with involution
$R$ with involution  $*$. To be precise, we prove the following. Let
$*$. To be precise, we prove the following. Let  $\delta :\,R\,\to \,R$ be a Jordan
$\delta :\,R\,\to \,R$ be a Jordan  $*$-derivation. Then there exists a
$*$-derivation. Then there exists a  $*$-algebra decomposition
$*$-algebra decomposition  $R\,=\,U\,\oplus \,V$ such that both
$R\,=\,U\,\oplus \,V$ such that both 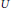 $U$ and
$U$ and 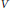 $V$ are invariant under
$V$ are invariant under  $\delta $. Moreover,
$\delta $. Moreover,  $*$ is the identity map of
$*$ is the identity map of 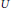 $U$ and
$U$ and 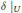 $\delta {{|}_{U}}$ is a derivation, and the Jordan
$\delta {{|}_{U}}$ is a derivation, and the Jordan  $*$-derivation
$*$-derivation  $\delta {{|}_{V}}$ is inner. We also prove the following. Let
$\delta {{|}_{V}}$ is inner. We also prove the following. Let  $R$ be a noncommutative, centrally closed prime algebra with involution
$R$ be a noncommutative, centrally closed prime algebra with involution  $*$, char
$*$, char 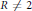 $R\,\ne \,2$, and let
$R\,\ne \,2$, and let  $\delta $ be a nonzero Jordan
$\delta $ be a nonzero Jordan  $*$-derivation of
$*$-derivation of  $R$. If
$R$. If  $\delta $ is an elementary operator of
$\delta $ is an elementary operator of  $R$, then
$R$, then 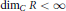 ${{\dim}_{C}}\,R\,<\,\infty $ and
${{\dim}_{C}}\,R\,<\,\infty $ and  $\delta $ is inner.
$\delta $ is inner.
- Type
- Research Article
- Information
- Copyright
- Copyright © Canadian Mathematical Society 2014
References
- 9
- Cited by


