Published online by Cambridge University Press: 20 November 2018
In this paper we describe six pencils of 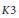 $K3$-surfaces which have large Picard number
$K3$-surfaces which have large Picard number 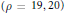 $\left( \rho =19,20 \right)$ and each contains precisely five special fibers: four have
$\left( \rho =19,20 \right)$ and each contains precisely five special fibers: four have 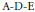 $\text{A-D-E}$ singularities and one is non-reduced. In particular, we characterize these surfaces as cyclic coverings of some
$\text{A-D-E}$ singularities and one is non-reduced. In particular, we characterize these surfaces as cyclic coverings of some 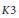 $K3$-surfaces described in a recent paper by Barth and the author. In many cases, using 3-divisible sets, resp., 2-divisible sets, of rational curves and lattice theory, we describe explicitly the Picard lattices.
$K3$-surfaces described in a recent paper by Barth and the author. In many cases, using 3-divisible sets, resp., 2-divisible sets, of rational curves and lattice theory, we describe explicitly the Picard lattices.