Endomorphisms That Are the Sum of a Unit and a Root of a Fixed Polynomial

Abstract

If 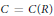$C=C\left( R \right)$ denotes the center of a ring $R$ and $g\left( x \right)$ is a polynomial in 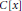$C\left[ x \right]$, Camillo and Simón called a ring $g\left( x \right)$-clean if every element is the sum of a unit and a root of $g\left( x \right)$. If $V$ is a vector space of countable dimension over a division ring 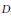$D$, they showed that 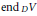$\text{en}{{\text{d}}_{\,D}}V$ is $g\left( x \right)$-clean provided that $g\left( x \right)$ has two roots in 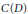$C\left( D \right)$. If 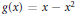$g\left( x \right)=x-{{x}^{2}}$ this shows that 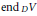$\text{en}{{\text{d}}_{\,D}}V$ is clean, a result of Nicholson and Varadarajan. In this paper we remove the countable condition, and in fact prove that 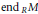$\text{en}{{\text{d}}_{\,R}}M$ is $g\left( x \right)$-clean for any semisimple module 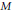$M$ over an arbitrary ring 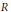$R$ provided that 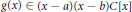$g\left( x \right)\in \left( x-a \right)\left( x-b \right)C\left[ x \right] $ where 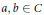$a,b\in C$ and both 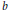$b$ and 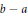$b-a$ are units in $R$.