Hostname: page-component-cd9895bd7-lnqnp Total loading time: 0 Render date: 2024-12-26T21:29:07.126Z Has data issue: false hasContentIssue false
  • English
  • Français

C*-Algebras of Infinite Graphs and Cuntz-Krieger Algebras

Published online by Cambridge University Press:  20 November 2018

Berndt Brenken
Berndt Brenken*
Affiliation:
Department of Mathematics & Statistics University of Calgary Calgary, Alberta T2N 1N4
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

The Cuntz-Krieger algebra 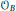${{\mathcal{O}}_{B}}$ is defined for an arbitrary, possibly infinite and infinite valued, matrix 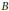$B$. A graph 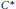${{C}^{*}}$-algebra ${{G}^{*}}\left( E \right)$ is introduced for an arbitrary directed graph $E$, and is shown to coincide with a previously defined graph algebra ${{C}^{*}}\left( E \right)$ if each source of $E$ emits only finitely many edges. Each graph algebra ${{G}^{*}}\left( E \right)$ is isomorphic to the Cuntz-Krieger algebra 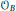${{\mathcal{O}}_{B}}$ where 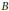$B$ is the vertex matrix of $E$.

Keywords

46LXX05C50
Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 45 , Issue 3 , 01 September 2002 , pp. 321 - 336
Copyright
Copyright © Canadian Mathematical Society 2002

References

[1] an Huef, A. and Raeburn, I., The ideal structure of Cuntz-Krieger algebras. Ergodic Theory Dynamical Systems 17 (1997), 17–1997.Google Scholar
[2] Brenken, B., Cuntz-Krieger algebras and endomorphisms of finite direct sums of type I factors. Trans. Amer.Math. Soc., to appear.Google Scholar
[3] Cuntz, J. and Krieger, W., A class of C*-algebras and topological Markov chains. Invent.Math. 56 (1980), 56–1980.Google Scholar
[4] Exel, R. and Laca, M., Cuntz-Krieger algebras for infinite matrices. J. Reine Angew. Math, to appear.Google Scholar
[5] Fowler, N., Laca, M., and Raeburn, I., The C*-algebras of infinite graphs. Preprint, 1998.Google Scholar
[6] Kumjian, A., Pask, D. and Raeburn, I., Cuntz-Krieger algebras of directed graphs. Pacific J. Math. (1) 184 (1998), 184–1998.Google Scholar
[7] Kumjian, A., Pask, D., Raeburn, I., and Renault, J., Graphs, Groupoids, and Cuntz-Krieger algebras. J. Funct. Anal. 144 (1997), 144–1997.Google Scholar
[8] Lind, D. and Marcus, B., An Introduction to Symbolic Dynamics and Coding. Cambridge University Press, 1995.Google Scholar
[9] Rordam, M., Classification of Cuntz-Krieger Algebras. K-theory 9 (1995), 9–1995.Google Scholar