Article contents
Approximation and the Topology of Rationally Convex Sets
Published online by Cambridge University Press: 20 November 2018
Abstract
Considering a mapping 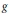 $g$ holomorphic on a neighbourhood of a rationally convex set
$g$ holomorphic on a neighbourhood of a rationally convex set 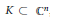 $K\subset {{\mathbb{C}}^{n}}$, and range into the complex projective space
$K\subset {{\mathbb{C}}^{n}}$, and range into the complex projective space  $\mathbb{C}{{\mathbb{P}}^{m}}$, the main objective of this paper is to show that we can uniformly approximate
$\mathbb{C}{{\mathbb{P}}^{m}}$, the main objective of this paper is to show that we can uniformly approximate 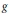 $g$ on
$g$ on 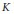 $K$ by rational mappings defined from
$K$ by rational mappings defined from 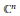 ${{\mathbb{C}}^{n}}$ into
${{\mathbb{C}}^{n}}$ into  $\mathbb{C}{{\mathbb{P}}^{m}}$. We only need to ask that the second Čech cohomology group
$\mathbb{C}{{\mathbb{P}}^{m}}$. We only need to ask that the second Čech cohomology group 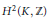 ${{\overset{\scriptscriptstyle\smile}{H}}^{2}}\left( K,\mathbb{Z} \right)$ vanishes.
${{\overset{\scriptscriptstyle\smile}{H}}^{2}}\left( K,\mathbb{Z} \right)$ vanishes.
- Type
- Research Article
- Information
- Copyright
- Copyright © Canadian Mathematical Society 2006
References
- 1
- Cited by


