No CrossRef data available.
Article contents
Analytic order-isomorphisms of countable dense subsets of the unit circle
Published online by Cambridge University Press: 14 July 2021
Abstract
For functions in 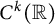 $C^k(\mathbb {R})$
which commute with a translation, we prove a theorem on approximation by entire functions which commute with the same translation, with a requirement that the values of the entire function and its derivatives on a specified countable set belong to specified dense sets. Using this theorem, we show that if A and B are countable dense subsets of the unit circle
$C^k(\mathbb {R})$
which commute with a translation, we prove a theorem on approximation by entire functions which commute with the same translation, with a requirement that the values of the entire function and its derivatives on a specified countable set belong to specified dense sets. Using this theorem, we show that if A and B are countable dense subsets of the unit circle 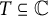 $T\subseteq \mathbb {C}$
with
$T\subseteq \mathbb {C}$
with 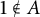 $1\notin A$
,
$1\notin A$
, 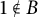 $1\notin B$
, then there is an analytic function
$1\notin B$
, then there is an analytic function 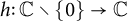 $h\colon \mathbb {C}\setminus \{0\}\to \mathbb {C}$
that restricts to an order isomorphism of the arc
$h\colon \mathbb {C}\setminus \{0\}\to \mathbb {C}$
that restricts to an order isomorphism of the arc 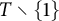 $T\setminus \{1\}$
onto itself and satisfies
$T\setminus \{1\}$
onto itself and satisfies  $h(A)=B$
and
$h(A)=B$
and 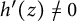 $h'(z)\not =0$
when
$h'(z)\not =0$
when  $z\in T$
. This answers a question of P. M. Gauthier.
$z\in T$
. This answers a question of P. M. Gauthier.
MSC classification
- Type
- Article
- Information
- Copyright
- © Canadian Mathematical Society 2021
Footnotes
Research supported by NSERC.



