Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Bellow, A.
and
Losert, V.
1985.
The weighted pointwise ergodic theorem and the individual ergodic theorem along subsequences.
Transactions of the American Mathematical Society,
Vol. 288,
Issue. 1,
p.
307.
Assani, Idris
1991.
Almost Everywhere Convergence II.
p.
9.
Jones, Roger L.
and
Olsen, James
1992.
Subsequence pointwise ergodic theorems for operators inL p.
Israel Journal of Mathematics,
Vol. 77,
Issue. 1-2,
p.
33.
Assani, I.
1992.
The return times and the Wiener—Wintner property for mean-bounded positive operators in Lp.
Ergodic Theory and Dynamical Systems,
Vol. 12,
Issue. 1,
p.
1.
Jones, Roger L.
and
Olsen, James
1994.
Multiparameter Weighted Ergodic Theorems.
Canadian Journal of Mathematics,
Vol. 46,
Issue. 2,
p.
343.
Lin, Michael
and
Olsen, James
1996.
Convergence in Ergodic Theory and Probability.
p.
277.
Çömez, Doğan
Lin, Michael
and
Olsen, James
1998.
Weighted ergodic theorems for mean ergodic 𝐿₁-contractions.
Transactions of the American Mathematical Society,
Vol. 350,
Issue. 1,
p.
101.
Lin, Michael
and
Tempelman, Arkady
1999.
Averaging sequences and modulated ergodic theorems for weakly almost periodic group representations.
Journal d'Analyse Mathématique,
Vol. 77,
Issue. 1,
p.
237.
Каримов, Абдусалом Кадиралиевич
Karimov, Abdusalom Kadiralievich
Мухамедов, Фаррух Максутович
and
Mukhamedov, Farrukh Maksutovich
2003.
Индивидуальная эргодическая теорема относительно равномерной последовательности и принцип Банаха в йордановых алгебрах.
Математический сборник,
Vol. 194,
Issue. 2,
p.
73.
Demeter, Ciprian
2004.
The Best Constants Associated with some Weak Maximal Inequalities in Ergodic Theory.
Canadian Journal of Mathematics,
Vol. 56,
Issue. 3,
p.
449.
Ganiev, Inomjon
and
Mukhamedov, Farrukh
2013.
Weighted ergodic theorems for Banach-Kantorovich lattice $$L_p \left( {\hat \nabla ,\hat \mu } \right)$$.
Lobachevskii Journal of Mathematics,
Vol. 34,
Issue. 1,
p.
1.
Ganiev, Inomjon
and
Mukhamedov, Farrukh
2015.
Weighted Ergodic Theorem for Contractions of Orlicz–Kantorovich lattice $$L_{M}(\widehat{\nabla },\widehat{\mu }$$ L M ( ∇ ^ , μ ^ ).
Bulletin of the Malaysian Mathematical Sciences Society,
Vol. 38,
Issue. 1,
p.
387.
Bianchi, Luigi Amedeo
Blömker, Dirk
and
Wacker, Philipp
2017.
Pattern Size in Gaussian Fields from Spinodal Decomposition.
SIAM Journal on Applied Mathematics,
Vol. 77,
Issue. 4,
p.
1292.
Hong, Guixiang
and
Sun, Mu
2018.
Noncommutative multi-parameter Wiener–Wintner type ergodic theorem.
Journal of Functional Analysis,
Vol. 275,
Issue. 5,
p.
1100.
Cuny, Christophe
Eisner, Tanja
and
Farkas, Bálint
2019.
Wiener's lemma along primes and other subsequences.
Advances in Mathematics,
Vol. 347,
Issue. ,
p.
340.
Chilin, Vladimir
and
Litvinov, Semyon
2020.
Noncommutative weighted individual ergodic theorems with continuous time.
Infinite Dimensional Analysis, Quantum Probability and Related Topics,
Vol. 23,
Issue. 02,
p.
2050013.
Sun, Mu
and
Zhang, Yinmei
2024.
Noncommutative Multi-Parameter Subsequential Wiener–Wintner-Type Ergodic Theorem.
Axioms,
Vol. 13,
Issue. 9,
p.
595.
 , μ) be a probability space, T a linear operator on ℒp(X,
, μ) be a probability space, T a linear operator on ℒp(X, 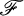 , μ), for some p, 1 ≦ p ≦ ∞. Let an be a sequence of complex numbers, n = 0, 1, …, which we shall often refer to as weights. We shall say that the weighted pointwise ergodic theorem holds for T on ℒp, if, for every ƒ in ℒp,
, μ), for some p, 1 ≦ p ≦ ∞. Let an be a sequence of complex numbers, n = 0, 1, …, which we shall often refer to as weights. We shall say that the weighted pointwise ergodic theorem holds for T on ℒp, if, for every ƒ in ℒp,



