Une propriété de domination convexe pour les orbites sturmiennes

Abstract

Let $\mathbf{x}\,=\,\left( {{x}_{0}},\,{{x}_{1}},.\,.\,. \right)$ be a 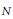$N$-periodic sequence of integers 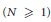$\left( N\,\ge \,1 \right)$, and 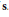$\mathbf{s}$ a sturmian sequence with the same barycenter (and also 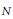$N$-periodic, consequently). It is shown that, for affine functions 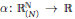$\alpha :\,\mathbb{R}_{(N)}^{\mathbb{N}}\,\to \,\mathbb{R}$ which are increasing relatively to some order 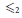${{\le }_{2}}$ on 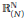$\mathbb{R}_{(N)}^{\mathbb{R}}$ (the space of all 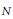$N$-periodic sequences), the average of $\left| \alpha \right|$ on the orbit of 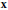$\mathbf{x}$ is greater than its average on the orbit of 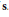$\mathbf{s}$.

Résumé

Soit $\mathbf{x}\,=\,\left( {{x}_{0}},\,{{x}_{1}},.\,.\,. \right)$ une suite 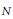$N$-périodique d'entiers 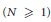$\left( N\,\ge \,1 \right)$, et 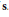$\mathbf{s}$ une suite sturmienne de même barycentre (et donc également 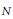$N$-périodique). On montre que, pour les fonctions affines 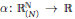$\alpha :\,\mathbb{R}_{(N)}^{\mathbb{N}}\,\to \,\mathbb{R}$ qui sont croissantes relativement à un certain ordre 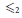${{\le }_{2}}$ sur 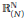$\mathbb{R}_{(N)}^{\mathbb{R}}$ (l'espace de toutes les suites 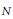$N$-périodiques), la moyenne de $\left| \alpha \right|$ sur l'orbite de 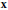$\mathbf{x}$ est plus grande que sa moyenne sur l'orbite de 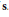$\mathbf{s}$.