Hostname: page-component-6bf8c574d5-7jkgd Total loading time: 0 Render date: 2025-02-23T11:13:26.689Z Has data issue: false hasContentIssue false
  • English
  • Français

On the Restriction to ${{\mathcal{D}}^{*}}\,\times \,{{\mathcal{D}}^{*}}$ of Representations of 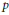$p$-adic $G{{L}_{2}}(\mathcal{D})$

Published online by Cambridge University Press:  20 November 2018

A. Raghuram
A. Raghuram*
Affiliation:
Department of Mathematics, Oklahoma State University, Stillwater, OK 74078, U.S.A. email: [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let $\mathcal{D}$ be a division algebra over a nonarchimedean local field. Given an irreducible representation $\pi $ of $G{{L}_{2}}(\mathcal{D})$, we describe its restriction to the diagonal subgroup ${{\mathcal{D}}^{*}}\,\times \,{{\mathcal{D}}^{*}}$. The description is in terms of the structure of the twisted Jacquet module of the representation $\pi $. The proof involves Kirillov theory that we have developed earlier in joint work with Dipendra Prasad. The main result on restriction also shows that $\pi $ is ${{\mathcal{D}}^{*}}\,\times \,{{\mathcal{D}}^{*}}$-distinguished if and only if $\pi $ admits a Shalika model. We further prove that if $\mathcal{D}$ is a quaternion division algebra then the twisted Jacquet module is multiplicity-free by proving an appropriate theorem on invariant distributions; this then proves a multiplicity-one theorem on the restriction to ${{\mathcal{D}}^{*}}\,\times \,{{\mathcal{D}}^{*}}$ in the quaternionic case.

Keywords

22E5022E3511S37
Type
Research Article
Information
Canadian Journal of Mathematics , Volume 59 , Issue 5 , 01 October 2007 , pp. 1050 - 1068
Copyright
Copyright © Canadian Mathematical Society 2007

References

[1] Badulescu, A. I., Correspondance de Jacquet-Langlands pour les corps locaux de caractéristique non nulle. Ann. Sci. École Norm. Sup. 35(2002), no. 5, 695–747.Google Scholar
[2] Baruch, E. M. and Rallis, S., A uniqueness theorem of Fourier Jacobi models for representations of Sp(4). J. London Math. Soc. 62(2000), no. 1, 183–197.Google Scholar
[3] Bernstein, J., P-invariant distributions on GL(n) and the classification of unitary representations of GL(N), (non-Archimedean case). In: Lie Group Representations. II. Lecture Notes in Math. 1041, Springer-Verlag, 1984, pp. 141–184.Google Scholar
[4] Bernšteĭn, I. N. and Zelevinskiĭ, A. V., Representation theory of GL(n, F) where F is a local non-Archimedean field . Uspehi Mat. Nauk, 31(1976), no. 3, 1–76.Google Scholar
[5] Bump, D., Automorphic forms and representations. Cambridge Studies in Advanced Mathematics 55, Cambridge University Press, Cambridge, 1997.Google Scholar
[6] Carayol, H., Représentations cuspidales du groupe linéaire. Ann. Sci. École Norm.Sup. 17(1984), no. 2, 191–225.Google Scholar
[7] Deligne, P., Kazhdan, D. and Vignéras, M.-F., Représentations des algèbres centrales simples p-adiques, In: Representations of reductive groups over a local field, Hermann, Paris, 1984, pp. 33–118.Google Scholar
[8] Jacquet, H. and Langlands, R., Automorphic Forms on GL2. Lecture Notes in Math. 114, Springer-Verlag, Berlin, 1970.Google Scholar
[9] Jacquet, H. and Rallis, S., Uniqueness of linear periods. Compositio Math. 102(1996), no. 1, 65–123.Google Scholar
[10] Kudla, S. S., The local Langlands correspondence: The non-Archimedean case. In Motives. Proc. Sympos. Pure Math. 55, American Mathematical Society, Providence, RI, 1994, pp. 365–391.Google Scholar
[11] Mœglin, C. and Waldspurger, J.-L., Modèles de Whittaker dégénérés pour des groupes p-adiques. Math. Z. 196(1987), no. 3, 427–452.Google Scholar
[12] Prasad, D., Some remarks on representations of a division algebra and of the Galois group of a local field. J. Number Theory, 74(1999), no. 1, 73–97.Google Scholar
[13] Prasad, D., The space of degenerate Whittaker models for GL(4) over p-adic fields. In: Cohomology of Arithmetic Groups, L-Functions and Automorphic Forms. Tata Inst. Fund. Res. Stud. Math. 15, Tata Inst. Fund. Res., Bombay, 2001, pp. 103–115.Google Scholar
[14] Prasad, D., The space of degenerate Whittaker models for general linear groups over finite and p-adic fields. http://www.math.tifr.res.in/_dprasad/tifr.pdf. Google Scholar
[15] Prasad, D. and Raghuram, A., Kirillov theory for. GL2( $ $ where $ $ is a division algebra over a non-Archimedean local field. Duke Math. J. 104(2000), no. 1, 19–44.Google Scholar
[16] Raghuram, A., Some Topics in Algebraic Groups : Representation Theory of GL2 ( $ $ ) Where $ $ Is a Division Algebra over a Non-Archimedean Local Field. Ph.D. Thesis, TIFR, University of Mumbai, 1999.Google Scholar
[17] Raghuram, A., On representations of p-adic GL2( $ $ ). Pacific J. Math. 206(2002), no. 2, 451–464.Google Scholar
[18] Raghuram, A., A Künneth theorem for p-adic groups, To appear, Canad. Math. Bull.Google Scholar
[19] Rogawski, J., Representations of GL(n) and division algebras over a p-adic field. Duke Math. J. 50(1983), no. 1, 161–196.Google Scholar
[20] Tadić, M., Induced representations of GL(n, A) for p-adic division algebras A. J. Reine Angew. Math. 405(1990), 48–77.Google Scholar
[21] Waldspurger, J.-L., Sur les valeurs de certaines fonctions L automorphes en leur centre de symétrie. Compositio Math. 54(1985), no. 2, 173–242.Google Scholar