Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Drinfel’d, G. I.
1972.
Algebra and Geometry.
p.
173.


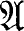 the group of unimodular affine transformations which operates on it. It is known that the sets of linear
the group of unimodular affine transformations which operates on it. It is known that the sets of linear