On the Convergence of a Class of Nearly Alternating Series

Abstract

Let $C$ be the class of convex sequences of real numbers. The quadratic irrational numbers can be partitioned into two types as follows. If $\alpha$ is of the first type and $\left( {{c}_{k}} \right)\,\in C$, then 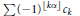$\sum{{{(-1)}^{\left\lfloor k\alpha \right\rfloor }}}{{c}_{k}}$ converges if and only if 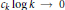${{c}_{k}}\log k\to 0$. If $\alpha$ is of the second type and $\left( {{c}_{k}} \right)\,\in C$, then 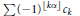$\sum{{{(-1)}^{\left\lfloor k\alpha \right\rfloor }}}{{c}_{k}}$ converges if and only if 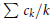$\sum{{{c}_{k}}/k}$ converges. An example of a quadratic irrational of the first type is 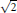$\sqrt{2}$, and an example of the second type is 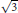$\sqrt{3}$. The analysis of this problem relies heavily on the representation of $\alpha$ as a simple continued fraction and on properties of the sequences of partial sums 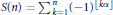$S\left( n \right)\,=\,{{\sum\nolimits_{k=1}^{n}{\left( -1 \right)}}^{\left\lfloor k\alpha \right\rfloor }}$ and double partial sums 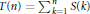$T\left( n \right)\,=\,\sum\nolimits_{k=1}^{n}{\,S\left( k \right)}$.