Hostname: page-component-78c5997874-m6dg7 Total loading time: 0 Render date: 2024-11-10T06:55:28.331Z Has data issue: false hasContentIssue false
  • English
  • Français

A Note on Continued Fractions

Published online by Cambridge University Press:  20 November 2018

A. Oppenheim
A. Oppenheim*
Affiliation:
University of Malaya
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Any real number y leads to a continued fraction of the type

(1)

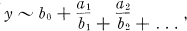
where ai, bi are integers which satisfy the inequalities

(2)

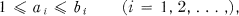
by means of the algorithm

(3)

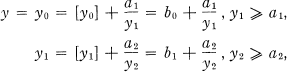
the a's being assigned positive integers. The process terminates for rational y; the last denominator bk satisfying bk ≥ ak + 1. For irrational y, the process does not terminate. For a preassigned set of numerators ai ≥ 1, this C.F. development of y is unique; its value being y.

Bankier and Leighton (1) call such fractions (1), which satisfy (2), proper continued fractions. Among other questions, they studied the problem of expanding quadratic surds in periodic continued fractions. They state that “it is well-known that not only does every periodic regular continued fraction represent a quadratic irrational, but the regular continued fraction expansion of a quadratic irrational is periodic.

Type
Research Article
Information
Canadian Journal of Mathematics , Volume 12 , 1960 , pp. 303 - 308
Copyright
Copyright © Canadian Mathematical Society 1960

References

1. Bankier, J.D. and Leighton, W., Numerical continued fractions, Amer. J. Math., 64 (1942), 653–668.Google Scholar