Hostname: page-component-586b7cd67f-g8jcs Total loading time: 0 Render date: 2024-11-27T22:15:41.636Z Has data issue: false hasContentIssue false
  • English
  • Français

Infinitely Many Solutions for the Prescribed Boundary Mean Curvature Problem in 𝔹N

Published online by Cambridge University Press:  20 November 2018

Liping Wang  and
Chunyi Zhao
Liping Wang
Affiliation:
Department of Mathematics, East China Normal University, Shanghai, 200241, China, e-mail: [email protected], [email protected]
Chunyi Zhao
Affiliation:
Department of Mathematics, East China Normal University, Shanghai, 200241, China, e-mail: [email protected], [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

We consider the prescribed boundary mean curvature problem in 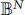${{\mathbb{B}}^{N}}$ with the Euclidean metric

$$\{_{\frac{\partial u}{\partial v}+\frac{N-2}{2}u=\frac{N-2}{2}\tilde{K}\left( x \right){{u}^{{{2}^{\#-1}}}}\,\,\,\,\,\,\text{on}{{\mathbb{S}}^{N-1}},}^{-\Delta u=0,\,\,\,\,\,\,u>0\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\text{in}{{\mathbb{B}}^{N}},}$$

where 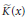$\tilde{K}\left( x \right)$ is positive and rotationally symmetric on ${{\mathbb{S}}^{N-1}},{{2}^{\#}}=\frac{2\left( N-1 \right)}{N-2}$. We show that if 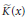$\tilde{K}\left( x \right)$ has a local maximum point, then this problem has infinitely many positive solutions that are not rotationally symmetric on 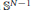${{\mathbb{S}}^{N-1}}$.

Keywords

35J2535J6535J67infinitely many solutionsprescribed boundary mean curvaturevariational reduction
Type
Research Article
Information
Canadian Journal of Mathematics , Volume 65 , Issue 4 , 01 August 2013 , pp. 927 - 960
Copyright
Copyright © Canadian Mathematical Society 2013

References

[1] Abdelhedi, W., Chtioui, H., and Ould Ahmedou, M., A Morse theoretical approach for boundary mean curvature problem on B4 , J. Funct. Anal. 254(2008), no. 5, 1307–1341. http://dx.doi.org/10.1016/j.jfa.2007.11.016 Google Scholar
[2] Almaraz, S., An existence theorem of conformal scalar-flat metrics on manifolds with boundary. Pacific J. Math. 248(2010), no. 1, 1–22. http://dx.doi.org/10.2140/pjm.2010.248.1 Google Scholar
[3] Ambrosetti, A., Li, Y. Y., and Malchiodi, A., On the Yamabe problem and the scalar curvature problem under boundary conditions. Math. Ann. 322(2002), no. 4, 667–699. http://dx.doi.org/10.1007/s002080100267 Google Scholar
[4] Brendle, S. and Chen, S., An existence theorem for the Yamabe problem on manifolds with boundary. arxiv:0908.4327v2. Google Scholar
[5] Cao, D.-M. and Peng, S.-J., Solutions for the prescribing mean curvature equation. Acta Math. Appl. Sin. Engl. Ser. 24(2008), no. 3, 497–510. http://dx.doi.org/10.1007/s10255-008-8051-8 Google Scholar
[6] Chang, S.-Y. A., Xu, X.-W., and Yang, P. C., A perturbation result for prescribing mean curvature. Math. Ann. 310(1998), no. 3, 473–496. http://dx.doi.org/10.1007/s002080050157 Google Scholar
[7] Chen, S.-Y. S., Conformal deformation to scalar flat metrics with constant mean curvature on the boundary in higher dimensions. arxiv:0912.1302v2. Google Scholar
[8] Cherrier, P., Problèmes de Neumann non linéaires sur les variétés Riemanniennes. J. Funct. Anal. 57(1984), no. 2, 154–206. http://dx.doi.org/10.1016/0022-1236(84)90094-6 Google Scholar
[9] zde Moura Almaraz, S., A compactness theorem for scalar-flat metrics on manifolds with boundary. Calc. Var. Partial Differential Equations 41(2011), no. 3–4, 341–386. http://dx.doi.org/10.1007/s00526-010-0365-8 Google Scholar
[10] del Pino, M., Felmer, P., and Musso, M., Two-bubble solutions in the super-critical Bahri-Coron’s problem. Calc. Var. Partial Differential Equations 16(2003), no. 2, 113–145. http://dx.doi.org/10.1007/s005260100142 Google Scholar
[11] Djadli, Z., Malchiodi, A., and Ouold Ahmedou, M., The prescribed boundary mean curvature problems on B4. J. Differential Equations 206(2004), no. 2, 373–398. http://dx.doi.org/10.1016/j.jde.2004.04.016 Google Scholar
[12] Escobar, J. F., Conformal deformation of Riemannian metric to scalar flat metric with constant mean curvature on the boundary. Ann. of Math. 136(1992), no. 1, 1–50. http://dx.doi.org/10.2307/2946545 Google Scholar
[13] Escobar, J. F., The Yamabe problem on manifolds with boundary. J. Differential Geom. 35(1992), no. 1, 21–84.Google Scholar
[14] Escobar, J. F., Conformal metrics with prescribed mean curvature on the boundary. Calc. Var. Partial Differential Equations 4(1996), no. 6, 559–592. http://dx.doi.org/10.1007/BF01261763 Google Scholar
[15] Escobar, J. F. and Garcia, G., Conformal metrics on the ball with zero scalar curvature and prescribed mean curvature on the boundary. J. Funct. Anal. 211(2004), no. 1, 71–152. http://dx.doi.org/10.1016/S0022-1236(03)00175-7 Google Scholar
[16] Felli, V. and Ould Ahmedou, M., Compactness results in conformal deformations of Riemannian metrics on manifolds with boundaries. Math. Z. 244(2003), no. 1, 175–210. http://dx.doi.org/10.1007/s00209-002-0486-7 Google Scholar
[17] Han, Z.-C. and Li, Y.-Y., The Yamabe problem on manifolds with boundary: existence and compactness results. Duke Math. J. 99(1999), no. 3, 489–542. http://dx.doi.org/10.1215/S0012-7094-99-09916-7 Google Scholar
[18] Li, Y.-Y. and Zhu, M.-J., Uniqueness theorems through the method of moving spheres. Duke Math. J. 80(1995), no. 2, 383–417. http://dx.doi.org/10.1215/S0012-7094-95-08016-8 Google Scholar
[19] Marques, F. C., Existence results for the Yamabe problem on manifolds with boundary. Indiana Univ. Math. J. 54(2005), no. 6, 1599–1620. http://dx.doi.org/10.1512/iumj.2005.54.2590 Google Scholar
[20] Marques, F. C., Conformal deformation to scalar-flat metrics with constant mean curvature on the boundary. Comm. Anal. Geom. 15(2007), no. 2, 381–405.Google Scholar
[21] Wei, J.-C. and Yan, S.-S., Infinitely many solutions for the prescribed scalar curvature problem on 𝕊N. J. Funct. Anal. 258(2010), no. 9, 3048–3081. http://dx.doi.org/10.1016/j.jfa.2009.12.008 Google Scholar