Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Yang, Zhilin
2011.
Positive solutions for a system ofp-Laplacian boundary value problems.
Computers & Mathematics with Applications,
Vol. 62,
Issue. 12,
p.
4429.
Yang, Zhilin
and
O’Regan, Donal
2012.
Positive solutions of a focal problem for one-dimensional p-Laplacian equations.
Mathematical and Computer Modelling,
Vol. 55,
Issue. 7-8,
p.
1942.
Tyagi, J.
2013.
Multiple solutions for singular N-Laplace equations with a sign changing nonlinearity.
Communications on Pure & Applied Analysis,
Vol. 12,
Issue. 6,
p.
2381.
Yang, Zhilin
Wang, Xiaomei
and
Li, Hongyu
2020.
Positive solutions for a system of second-order quasilinear boundary value problems.
Nonlinear Analysis,
Vol. 195,
Issue. ,
p.
111749.
Mohamed, Alhussein
Wang, Jingjing
Gao, Chenghua
and
Yao, Xiaobin
2022.
Existence of Positive Solution for Second-Order Singular Semipositone Difference Equation with Nonlinear Boundary Conditions.
Mediterranean Journal of Mathematics,
Vol. 19,
Issue. 6,
Yang, Lijuan
and
Ma, Ruyun
2024.
INFINITELY MANY SOLUTIONS FOR A <i>P</i>-SUPERLINEAR <i>P</i>-LAPLACIAN PROBLEMS.
Journal of Applied Analysis & Computation,
Vol. 14,
Issue. 3,
p.
1778.
 $$\left\{ _{u\left( 0 \right)\,=\,u\left( 1 \right)\,=\,0}^{-{{\left( {{\left| {{u}'} \right|}^{p-2}}{u}' \right)}^{\prime }}\,=\,\lambda f\left( t,\,u \right),\,t\,\in \,\left( 0,\,1 \right),\,p\,>\,1} \right.$$
$$\left\{ _{u\left( 0 \right)\,=\,u\left( 1 \right)\,=\,0}^{-{{\left( {{\left| {{u}'} \right|}^{p-2}}{u}' \right)}^{\prime }}\,=\,\lambda f\left( t,\,u \right),\,t\,\in \,\left( 0,\,1 \right),\,p\,>\,1} \right.$$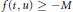 $f(t,u)\ge -M$, (
$f(t,u)\ge -M$, (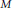 $M$ is a positive constant) for
$M$ is a positive constant) for 


