No CrossRef data available.
Published online by Cambridge University Press: 20 November 2018
Every abstract open Riemann surface can be made "concrete" (in the terminology of (1)) by considering it as a covering surface (in general branched) of the complex plane 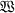 by means of a suitable projection map p. Since this covering map is not unique, it seems natural to single out some such maps by an extremal property. The use of Riemannian metrics compatible with the conformai structure on the given surface
by means of a suitable projection map p. Since this covering map is not unique, it seems natural to single out some such maps by an extremal property. The use of Riemannian metrics compatible with the conformai structure on the given surface  for the study of $1 is well known ; from the point of view of differential geometry it suggests an investigation of the distortion caused by p between such a metric ds^ and the Euclidean metric of
for the study of $1 is well known ; from the point of view of differential geometry it suggests an investigation of the distortion caused by p between such a metric ds^ and the Euclidean metric of 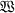 .
.