Hostname: page-component-cd9895bd7-jn8rn Total loading time: 0 Render date: 2024-12-26T16:42:18.549Z Has data issue: false hasContentIssue false
  • English
  • Français

Antichains and Finite Sets that Meet all Maximal Chains

Published online by Cambridge University Press:  20 November 2018

J. Ginsburg ,
I. Rival  and
B. Sands
J. Ginsburg
Affiliation:
University of Winnipeg, Winnipeg, Manitoba
I. Rival
Affiliation:
The University of Calgary, Calgary, Alberta
B. Sands
Affiliation:
The University of Calgary, Calgary, Alberta
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

This paper is inspired by two apparently different ideas. Let P be an ordered set and let M(P) stand for the set of all of its maximal chains. The collection of all sets of the form

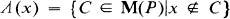
and

where x ∊ P, is a subbase for the open sets of a topology on M(P). (Actually, it is easy to check that the B(x) sets themselves form a subbase.) In other words, as M(P) is a subset of the power set 2|p| of P, we can regard M(P) as a subspace of 2|p| with the usual product topology. M. Bell and J. Ginsburg [1] have shown that the topological space M(P) is compact if and only if, for each x ∊ P, there is a finite subset C(x) of P all of whose elements are noncomparable to x and such that {x} ∪ C(x) meets each maximal chain.

Type
Research Article
Information
Canadian Journal of Mathematics , Volume 38 , Issue 3 , 01 June 1986 , pp. 619 - 632
Copyright
Copyright © Canadian Mathematical Society 1986

References

1. Bell, M. and Ginsburg, J., Compact spaces and spaces of maximal complete subgraphs, Trans. Amer. Math. Soc. 283 (1984), 329–339.Google Scholar
2. Dilworth, R. P., A decomposition theorem for partially ordered sets, Ann. of Math. 57 (1950), 161–166.Google Scholar
3. Erdös, P. and Rado, R., A partition calculus in set theory, Bull. Amer. Math. Soc. 62 (1956), 427–489.Google Scholar
4. Rudin, M. E., Lectures on set-theoretic topology, Publications Amer. Math. Soc. 23 (1975).CrossRefGoogle Scholar
5. Sauer, N. and Woodrow, R. E., Finite cutsets and antichains, Order 1 (1984), 35–46.Google Scholar