No CrossRef data available.
Published online by Cambridge University Press: 07 November 2014
In recent years, an attempt has been made by the present author to measure the probable slope of the marginal productivity functions of labour and capital in various economies. This has been done by computing indexes of capital (C), labour (L), and product (P) for manufacturing in the United States for the years 1899-1922, Massachusetts 1890-1926, New South Wales 1901-1927, and Victoria 1907-1929. The formula used is that P' (representing an index of computed product) = b LkC(1−k). By making the sum of exponents equal to unity, we assumed for purposes of simplicity that production could be described by a homogeneous function of the first degree and that equal proportionate increases in the quantities of labour and capital would cause the same proportionate increase in product. The exponents of L and C were found by the method of least squares, so that the sum of the squares of the deviation of P′ from P would be reduced to a minimum, and the sum of the arithmetic deviations to zero. The exponents for labour and capital in manufacturing which were found by this method were as follows:
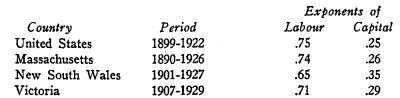
It will be noticed that there is a substantial degree of similarity between the exponents found for these economies.
A mathematical analysis of the above function discloses: (1) that the share of the net product to be received by a factor should, according to marginal productivity theory, be equal to its exponent; (2) that the elasticity of the marginal productivity curve of, and hence, under competitive conditions, the demand curve for, a factor is the reciprocal of the sum of the exponents of other factors. This would mean a “normal” elasticity of demand for labour of between −2.86 and −4.0, and for capital of between −1.33 and −1.54.
1 See Douglas, P. H., The Theory of Wages (New York, 1934), pp. 113–225.Google Scholar The Massachusetts indexes were computed by Professor Charles W. Cobb of Amherst College, and those for New South Wales by Mr. Aaron Director.
2 See an article by Mrs.Handsaker, M. L. and myself, “The Theory of Marginal Productivity as Tested by Data for Manufacturing in Victoria, 1907-1929” (Quarterly Journal of Economics, LI, 11, 1936, and Feb., 1937).Google Scholar Land was omitted in all of these studies because it could not be measured, but in any study of an entire society, it should, of course, be included.
3 This formula was developed for the United States by Professor Charles W. Cobb, to whom I have been indebted for fundamental aid.
4 For proof, see Douglas, , The Theory of Wages, pp. 145–58Google Scholar; Handsaker and Douglas, “The Theory of Marginal Productivity”; Pigou, A. C., The Economics of Welfare (ed. 2, London, 1924), p. 623 Google Scholar; Dalton, H., Some Aspects of the Inequality of Incomes (London, 1920), pp. 186–7.Google Scholar
5 National Bureau of Economic Research, Income in the United States (New York, 1921), vol. II, p. 98.Google Scholar
6 On Quantitative Thinking in Economics (Oxford, 1935), pp. 119–50.Google Scholar
7 Ibid., p. 123.
8 In dealing with an individual industry, the margin is not merely a physical one, but rather one of value in which (1) incremental physical product and (2) unit exchange values or price are both controlling under conditions of perfect competition. Under imperfect competition, marginal revenue replaces price as a determinant.
9 On Quantitative Thinking, p. 125.
10 Cassel, G., The World's Monetary Problems (London, 1921)Google Scholar; and Money and Foreign Exchange After 1914 (New York, 1923).Google Scholar For a trenchant exposure of Professor Cassel's weaknesses on this point, see Terborgh, G. W., “The Purchasing Power Parity Theory” (Journal of Political Economy, vol. XXXIV, 1926, pp. 197–208).CrossRefGoogle Scholar
11 Or by the percentage of the value product represented by the commodity.
12 On Quantitative Thinking, pp. 146-7.
13 See Professor Cassel's statement, “… the total product is a great mass of different commodities and services of the most varying nature and there is no technical possibility of expressing this mass as a measurable arithmetical quantity. Only if the different commodities and services have definite prices may the value of the total product be expressed in terms of money. The mass thus becomes a measurable arithmetical quantity. Thus it is impossible to speak of the marginal productivity of any factor in the great social process of production except when the prices of the different factors are assumed to be known. But in this case the marginal productivity of each factor is simply its own price” (On Quantitative Thinking, p. 125).
14 Of course the actual marginal productivity is itself influenced by various factors such as the slope and location of the incremental product curves, the elasticities of substitution, the respective quantities supplied of the factors, and the nature and slope of their supply functions.
15 Since it is highly unlikely that the sum of the exponents of L and C would greatly vary from unity. Thus Mr. David Durand in using this method of attack upon the American data which I assembled found their sum to be 1.01, while Mrs. Handsaker and I found it to be 1.067 for Victoria. In the latter study, the value of b had to be reduced to .71, which gives it a very doubtful economic meaning.
16 On Quantitative Thinking, p. 137.
17 Nor, it may be added, need commodities have a constant elasticity. Only a little reflection is needed for an example, to show the essential absurdity of believing that an article like wheat must have a highly inelastic demand over its entire range. For if it did, a handful would be worth the entire national income.
18 Douglas, , The Theory of Wages, p. 220.Google Scholar
19 Ibid., p. 221.
20 See Bowley, A. L., The Change in the Distribution of the National Income, 1880-1913 (Oxford, 1920), pp. 24–5Google Scholar; Bowley, A. L. and Stamp, J. C., The National Income 1924 (Oxford, 1927), p. 50.Google Scholar For data for the other countries, see Stamp, J. C., “The Wealth and Income of the Chief Powers” (Journal of the Royal Statistical Society, vol. LXXXII, 1919, pp. 441–93)CrossRefGoogle Scholar; Dalton, , Some Aspects of the Inequality of Incomes, pp. 209 ff. Google Scholar; Bresciani-Turroni, C., “On Some Changes in the Distribution of Income and Property in Germany after 1913” (Revue Al Qanoun Wal Iqtisad, Cairo, 1931)Google Scholar; King, W. L., The National Income (New York, National Bureau of Economic Research, 1930).Google Scholar
21 Cassel, G., The Theory of Social Economy, trans. McCabe, J. (New York, 1924), pp. 34–42.Google Scholar
22 Cassel, , Fundamental Thoughts in Economics (New York, 1925), p. 41.Google Scholar
23 I.e., in The Theory of Social Economy, pp. 35-6.
24 On Quantitative Thinking, pp. 136-7.
25 I.e., production.
26 Cassel, , The Theory of Social Economy, p. 41.Google Scholar
27 This is Professor Cassel's own numerical illustration. See his Quantitative Thinking, pp. 134-5. The recent study by Lindahl, , Dahlgren, , and Kock, on The National Income of Sweden 1861-1930 (London, 1937),Google Scholar does not show this complete constancy of the proportion of the national income which is saved. Thus, the proportion which the production of durable goods formed of all material commodities produced was as follows (ibid., pp. 108-9):

The percentage of total national income invested in durable goods other than buildings was 5.2 per cent in 1861-70; 7.2 per cent in 1876-80; 15.6 per cent in 1921-30. If buildings are included, the record for a shorter period of years does, however, show some tendency towards uniformity: i.e., 22.9 per cent for the years 1896-1900; 25.5 per cent for 1901-10; 27.8 per cent for 1911-20; and 26.9 per cent for 1921-30 (ibid., pp. 234-5, 254-5). There is, however, no fundamental reason why this uniformity should continue to exist, or why it should be true of other countries.
28 Cassel, , The Theory of Social Economy, pp. 441–51.Google Scholar
29 Ibid., pp. 556-71, 586-95, 642-6.
30 Cassel, , Fundamental Thoughts in Economics, pp. 41–2.Google Scholar
31 On Quantitative Thinking, p. 136.
32 Snyder, Carl, “Measurement Versus Theory in Economics” (in Economic Essays in Honor of Gustav Cassel (London, 1933), pp. 591–7),Google Scholar and Business Cycles and Business Measurements (New York, 1927), p. 51.Google Scholar See also Snyder, , “The Measurement of Monetary Phenomena” (in The Norman Wait Harris Memorial Foundation, Ninth Institute, Gold and Monetary Stabilization, 1932, pp. 256–8),Google Scholar where he states that a world production index covering the period from 1865 to the World War showed a characteristic rate of 3 per cent.
33 Douglas, , The Theory of Wages, p. 168.Google Scholar
34 See Douglas, , “An Estimate of the Growth of Capital in the United Kingdom 1865-1909” (Journal of Economic and Business History, vol. II, 1930, pp. 659–84)Google Scholar; Douglas, , The Theory of Wages, pp. 465–7.Google Scholar There is also some material in the recent study by Lindahl, , Dahlgren, , and Kock, , The National Income of Sweden vol. I, pp. 251–94.Google Scholar
35 I.e., the income elasticity of savings is appreciably greater than unity.
36 On Quantitative Thinking, p. 124.
37 Cassel, , The Theory of Social Economy, p. 75.Google Scholar
38 See Wicksell, K.'s review of The Theory of Social Economy, first printed in Ekonomisk Tidskrift, 1919,Google Scholar no. 9, and Schmoller, 's Jahrbuch, 1928, vol. LII-2, no. 5,Google Scholar and reprinted in the English translation of his Lectures on Political Economy (London, 1934), vol. I, pp. 219–57.Google Scholar Also the review of the same book by Edgeworth, F. Y. reprinted in Papers Relating to Political Economy (London, 1925), vol. III, pp. 266–72.Google Scholar