No CrossRef data available.
Article contents
ON UNRAMIFIED SOLVABLE EXTENSIONS OF SMALL NUMBER FIELDS
Published online by Cambridge University Press: 09 November 2020
Abstract
We investigate unramified extensions of number fields with prescribed solvable Galois group G and certain extra conditions. In particular, we are interested in the minimal degree of a number field K, Galois over 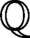 $\mathbb {Q}$
, such that K possesses an unramified G-extension. We improve the best known bounds for the degree of such number fields K for certain classes of solvable groups, in particular for nilpotent groups.
$\mathbb {Q}$
, such that K possesses an unramified G-extension. We improve the best known bounds for the degree of such number fields K for certain classes of solvable groups, in particular for nilpotent groups.
- Type
- Research Article
- Information
- Copyright
- © 2020 Australian Mathematical Publishing Association Inc.
References
Baumslag, G., ‘Wreath products and
 $p$
-groups’, Math. Proc. Cambridge Philos. Soc. 55(3) (1959), 224–231.CrossRefGoogle Scholar
$p$
-groups’, Math. Proc. Cambridge Philos. Soc. 55(3) (1959), 224–231.CrossRefGoogle Scholar
Bosma, W., Cannon, J. and Playoust, C., ‘The Magma algebra system. I. The user language’, J. Symbolic Comput. 24(3–4) (1997), 235–265.CrossRefGoogle Scholar
Hall, P., ‘A contribution to the theory of groups of prime power order’, Proc. Lond. Math. Soc. Ser. 2 36(1) (1934), 29–95.CrossRefGoogle Scholar
Kim, K.-S., ‘Construction of unramified extensions with a prescribed Galois group’, Osaka J. Math. 52 (2015), 1039–1050.Google Scholar
Kim, K.-S., ‘Construction of unramified extensions with a prescribed solvable Galois group’, Acta Arith. 190 (2019), 49–56.CrossRefGoogle Scholar
Kim, K.-S. and König, J., ‘On Galois extensions with prescribed decomposition groups’, J. Number Theory, to appear.Google Scholar
König, J., Neftin, D. and Sonn, J., ‘Unramified extensions over low degree number fields’, J. Number Theory 212 (2020), 72–87.CrossRefGoogle Scholar
Neukirch, J., Schmidt, A. and Wingberg, K., Cohomology of Number Fields (Springer, Berlin, 2000).Google Scholar
Nomura, A., ‘On the existence of unramified
 $p$
-extensions with prescribed Galois group’, Osaka J. Math. 47 (2010), 1159–1165.Google Scholar
$p$
-extensions with prescribed Galois group’, Osaka J. Math. 47 (2010), 1159–1165.Google Scholar
Uchida, K., ‘Unramified extensions of quadratic number fields, II’, Tohoku Math. J. 22 (1970), 220–224.Google Scholar
Wood, M. M., ‘Nonabelian Cohen–Lenstra moments’, Duke Math. J. 168(3) (2019), 377–427.CrossRefGoogle Scholar
Yamamoto, Y., ‘On unramified Galois extensions of quadratic number fields’, Osaka J. Math. 7 (1970), 57–76.Google Scholar



