Published online by Cambridge University Press: 17 April 2009
Let J ⊆ C∞ (Rn) be any ideal. Since a function of the variables 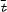 = (t1,…,tn) is a function of the variables
= (t1,…,tn) is a function of the variables 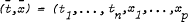 which does not depend on
which does not depend on 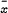 , we have J ⊆ C∞ (IRn+P). Of course, J is not an ideal of C∞ (IRn+P), but it generates an ideal that we call
, we have J ⊆ C∞ (IRn+P). Of course, J is not an ideal of C∞ (IRn+P), but it generates an ideal that we call 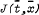 . Consider the following statement (1) on J: “Given any
. Consider the following statement (1) on J: “Given any  if and only if for every fixed
if and only if for every fixed 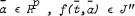 .
.
In this paper we show that statement (1) holds for a large class of finitely generated ideals although not for all of them. We say that ideals satisfying statement (1) have line determined extensions. We characterize these ideals to be closed ideals J(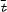 ) (in the sense of Whitney) such that for all p ∈ ℕ, the ideal
) (in the sense of Whitney) such that for all p ∈ ℕ, the ideal 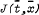 is also closed. Finally, some non-trivial examples are developed.
is also closed. Finally, some non-trivial examples are developed.